Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
lời giải
a) \(\left\{{}\begin{matrix}-2x+\dfrac{3}{5}>\dfrac{2x-7}{3}\left(1\right)\\x-\dfrac{1}{2}< \dfrac{5\left(3x-1\right)}{2}\left(2\right)\end{matrix}\right.\)
(1)\(\Leftrightarrow\)
\(\dfrac{3}{5}+\dfrac{7}{3}>\left(\dfrac{2}{3}+2\right)x\)
\(\dfrac{44}{15}>\dfrac{8}{3}x\) \(\Rightarrow x< \dfrac{44.3}{15.8}=\dfrac{11}{5.2}=\dfrac{11}{10}\)
Nghiêm BPT(1) là \(x< \dfrac{11}{10}\)
(2) \(\Leftrightarrow2x-1< 15x-5\Rightarrow13x>4\Rightarrow x>\dfrac{4}{13}\)
Ta có: \(\dfrac{4}{13}< \dfrac{11}{10}\) => Nghiệm hệ (a) là \(\dfrac{4}{13}< x< \dfrac{11}{10}\)
a: TH1: x>=2
=>2x-4<=x+12
=>x<=16
=>2<=x<=16
TH2: x<2
=>4-2x<=x+12
=>-3x<=8
=>x>=-8/3
=>-8/3<=x<2
b: TH1: x>=1
BPT sẽ là \(\dfrac{x-1}{x+2}< 1\)
=>(x-1-x-2)/(x+2)<0
=>x+2<0
=>x<-2(loại)
TH2: x<1
BPT sẽ là \(\dfrac{1-x}{x+2}-1< 0\)
=>(1-x-x-2)/(x+2)<0
=>(-2x-1)/(x+2)<0
=>(2x+1)/(x+2)>0
=>x>-1/2 hoặc x<-2
=>-1/2<x<1 hoặc x<-2
a: =>4x+12<=2x-1
=>2x<=-13
=>x<=-13/2
b: =>x^2-2x+1+4<0
=>(x-1)^2+4<0(loại)
c: =>(x-2+x+3)/(x+3)<0
=>(2x+1)/(x+3)<0
=>-3<x<-1/2
pt(1)\(\dfrac{\left(x-3\right)^2}{3}-\dfrac{\left(2x-1\right)^2}{12}\le x\)
\(\Leftrightarrow\dfrac{4\left(x-3\right)^2}{12}-\dfrac{\left(2x-1\right)^2}{12}\le x\)
\(\Leftrightarrow\dfrac{\left(2x-6\right)^2-\left(2x-1\right)^2}{12}\le x\)
\(\Leftrightarrow-5\cdot\left(4x-7\right)\le12x\)
\(\Leftrightarrow-20x+35\le12x\)
\(\Leftrightarrow32x\ge35\)
\(\Leftrightarrow x\ge\dfrac{35}{32}\left(1\right)\)
Pt(2)\(\Leftrightarrow2+x+1< \dfrac{12-x+1}{4}\)
\(\Leftrightarrow x+3< \dfrac{13-x}{4}\)
\(\Leftrightarrow4x+12< 13-x\)
\(\Leftrightarrow5x< 1\)
\(\Leftrightarrow x< \dfrac{1}{5}\left(2\right)\)
(1) và (2) mâu thuẫn =>không có x tm cả 2 bpt trên
a,\(\dfrac{5x-2}{2-2x}+\dfrac{2x-1}{2}=1-\dfrac{x^2-x-3}{1-x}\)
<=>\(\dfrac{5x-2}{2\left(1-x\right)}+\dfrac{2x-1}{2}=1-\dfrac{x^2-x-3}{1-x}\)
<=>\(\dfrac{5x-2}{2\left(1-x\right)}+\dfrac{\left(2x-1\right)\left(1-x\right)}{2\left(1-x\right)}=\dfrac{2\left(1-x\right)}{2\left(1-x\right)}-\dfrac{2\left(x^2-x-3\right)}{2\left(1-x\right)}\)
=>\(5x-2+2x-2x^2-1+x=2-2x-2x^2+2x+6\)
<=>\(-2x^2+8x-3=-2x^2+8\)
<=>\(8x=11< =>x=\dfrac{11}{8}\)
vậy..........
b,\(\dfrac{1-6x}{x-2}+\dfrac{9x+4}{x+2}=\dfrac{x\left(3x-1\right)+1}{\left(x-2\right)\left(x+2\right)}\)
<=>\(\dfrac{\left(1-6x\right)\left(x+2\right)}{\left(x-2\right)\left(x+2\right)}+\dfrac{\left(9x+4\right)\left(x-2\right)}{\left(x+2\right)\left(x-2\right)}=\dfrac{x\left(3x-1\right)+1}{\left(x-2\right)\left(x+2\right)}\)
=>\(x+2-6x^2-12x+9x^2-18x+4x-8=3x^2-x+1\)
<=>\(3x^2-25x-6=3x^2-x+1\)
<=>\(-24x=7< =>x=\dfrac{-7}{24}\)
vậy..................
câu c tương tự nhé :)
a) Đkxđ: \(x\ne1,x\ne0\)
⇔x+1x−1+2>x−1x⇔2x−1+2>−1x⇔x+1x−1+2>x−1x⇔2x−1+2>−1x
⇔2x−1+1x+2>0⇔2x+x−1+2(x2−x)(x−1)x=2x2+x−1(x−1)(x)>0⇔2x−1+1x+2>0⇔2x+x−1+2(x2−x)(x−1)x=2x2+x−1(x−1)(x)>0
Tử {delta =9}
−1<x<12⇒Tử<0
0<x<1⇒M<0
Nghiệm BPT là
[x<−10<x<12 hoặc x>1
e: =>-3<5x-12<3
=>9<5x<15
=>9/5<x<3
f: =>3x+15>=3 hoặc 3x+15<=-3
=>3x>=-12 hoặc 3x<=-18
=>x<=-6 hoặc x>=-4
b: =>(2x-7)(x-5)<=0
=>7/2<=x<=5
a)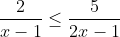
<=> f(x) =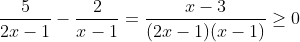
.
Xét dấu của f(x) ta được tập nghiệm của bất phương trình:
T =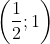
∪ [3; +∞).
b)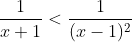
<=> f(x) =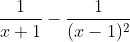
= 
.
f(x) không xác định với x = ± 1.
Xét dấu của f(x) cho tập nghiệm của bất phương trình:
T = (-∞; - 1) ∪ (0; 1) ∪ (1; 3).
c)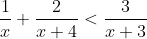
<=> f(x) =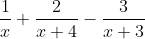 
=
.
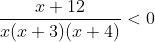Tập nghiệm: \(\left(-12;-4\right)\cup\left(-3;0\right)\).