
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Đổi 30 phút = 0,5 giờ
Quãng sông từ A đến B dài là:
\(x\) \(\times\) 0,5 + y \(\times\) 1 = 0,5\(x\) + y (km)
Kết luận Quãng đường từ A đên B dài: 0,5\(x\) + y (km)

Lời giải:
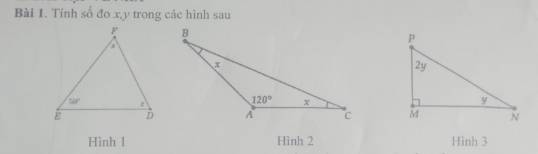
Áp dụng tính chất tổng 3 góc trong 1 tam giác bằng $180^0$
Hình 1: Hình không rõ ràng. Bạn xem lại.
Hình 2: $x+x+120^0=180^0$
$2x+120^0=180^0$
$2x=60^0$
$x=60^0:2=30^0$
Hình 3:
$2y+y+90^0=180^0$
$3y=180^0-90^0=90^0$
$y=90^0:3=30^0$

\(5x=3y\Rightarrow x=\dfrac{3y}{5}\)
Thay \(x=\dfrac{3y}{5}\) vào biểu thức \(x^2-y^2=-4\) ta có:
\(\left(\dfrac{3y}{5}\right)^2-y^2=-4\)
\(\dfrac{9y^2}{25}-y^2=-4\)
\(-\dfrac{16}{25}y^2=-4\)
\(y^2=-\dfrac{4}{\dfrac{-16}{25}}\)
\(y^2=\dfrac{25}{4}\)
\(\Rightarrow y=-\dfrac{5}{2};y=\dfrac{5}{2}\)
*) \(y=-\dfrac{5}{2}\Rightarrow x=\dfrac{3.\left(-\dfrac{5}{2}\right)}{5}=-\dfrac{3}{2}\)
*) \(y=\dfrac{5}{2}\Rightarrow x=\dfrac{3.\dfrac{5}{2}}{5}=\dfrac{3}{2}\)
Vậy ta được các cặp giá trị \(\left(x;y\right)\) thỏa mãn:
\(\left(-\dfrac{3}{2};-\dfrac{5}{2}\right);\left(\dfrac{3}{2};\dfrac{5}{2}\right)\)

Lời giải:
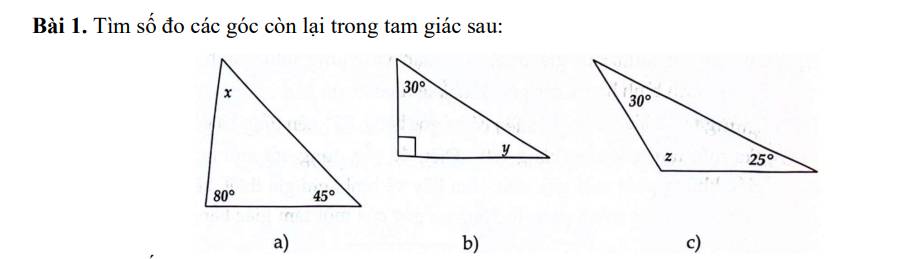
Áp dụng tính chất tổng 3 góc trong một tam giác bằng $180^0$
a.
$x=180^0-80^0-45^0=55^0$
b.
$y=180^0-30^0-90^0=60^0$
c.
$z=180^0-30^0-25^0=125^0$
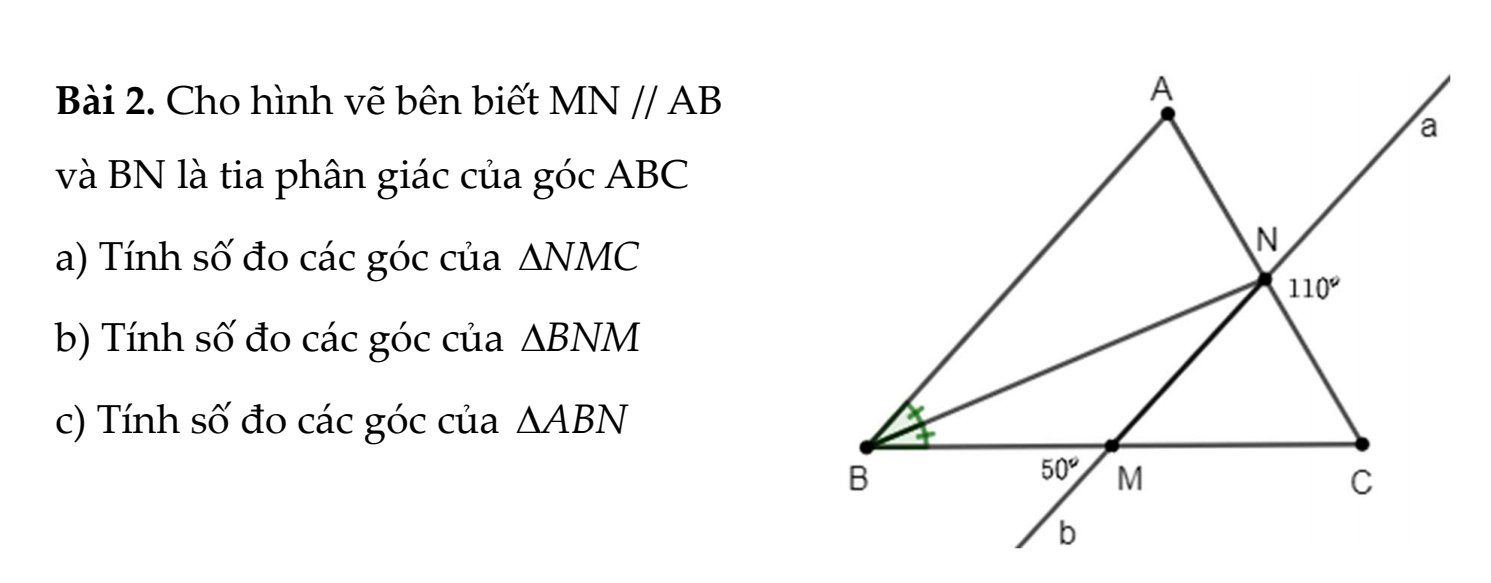


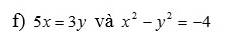
a: Ta có: \(\widehat{bMB}=\widehat{NMC}\)(hai góc đối đỉnh)
mà \(\widehat{bMB}=50^0\)
nên \(\widehat{NMC}=50^0\)
Ta có: \(\widehat{MNC}+\widehat{aNC}=180^0\)(hai góc kề bù)
=>\(\widehat{MNC}+110^0=180^0\)
=>\(\widehat{MNC}=70^0\)
Xét ΔMNC có \(\widehat{NMC}+\widehat{MNC}+\widehat{C}=180^0\)
=>\(\widehat{C}+50^0+70^0=180^0\)
=>\(\widehat{C}=60^0\)
b: Ta có: \(\widehat{NMB}+\widehat{NMC}=180^0\)(hai góc kề bù)
=>\(\widehat{NMB}+50^0=180^0\)
=>\(\widehat{NMB}=130^0\)
Ta có: MN//AB
=>\(\widehat{CMN}=\widehat{CBA}\)(hai góc đồng vị)
=>\(\widehat{CBA}=50^0\)
BN là phân giác của góc CBA
=>\(\widehat{NBM}=\dfrac{\widehat{ABC}}{2}=25^0\)
Xét ΔNMB có \(\widehat{NMB}+\widehat{BNM}+\widehat{NBM}=180^0\)
=>\(\widehat{MNB}=180^0-130^0-25^0=25^0\)
c: BN là phân giác của góc CBA
=>\(\widehat{ABN}=\dfrac{\widehat{ABC}}{2}=25^0\)
Xét ΔABC có \(\widehat{ABC}+\widehat{ACB}+\widehat{BAC}=180^0\)
=>\(\widehat{BAN}+60^0+50^0=180^0\)
=>\(\widehat{BAN}=70^0\)
Xét ΔBAN có \(\widehat{BAN}+\widehat{ABN}+\widehat{ANB}=180^0\)
=>\(\widehat{ANB}=180^0-75^0-25^0=85^0\)