Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(5x=3y\Rightarrow x=\dfrac{3y}{5}\)
Thay \(x=\dfrac{3y}{5}\) vào biểu thức \(x^2-y^2=-4\) ta có:
\(\left(\dfrac{3y}{5}\right)^2-y^2=-4\)
\(\dfrac{9y^2}{25}-y^2=-4\)
\(-\dfrac{16}{25}y^2=-4\)
\(y^2=-\dfrac{4}{\dfrac{-16}{25}}\)
\(y^2=\dfrac{25}{4}\)
\(\Rightarrow y=-\dfrac{5}{2};y=\dfrac{5}{2}\)
*) \(y=-\dfrac{5}{2}\Rightarrow x=\dfrac{3.\left(-\dfrac{5}{2}\right)}{5}=-\dfrac{3}{2}\)
*) \(y=\dfrac{5}{2}\Rightarrow x=\dfrac{3.\dfrac{5}{2}}{5}=\dfrac{3}{2}\)
Vậy ta được các cặp giá trị \(\left(x;y\right)\) thỏa mãn:
\(\left(-\dfrac{3}{2};-\dfrac{5}{2}\right);\left(\dfrac{3}{2};\dfrac{5}{2}\right)\)

Lời giải:
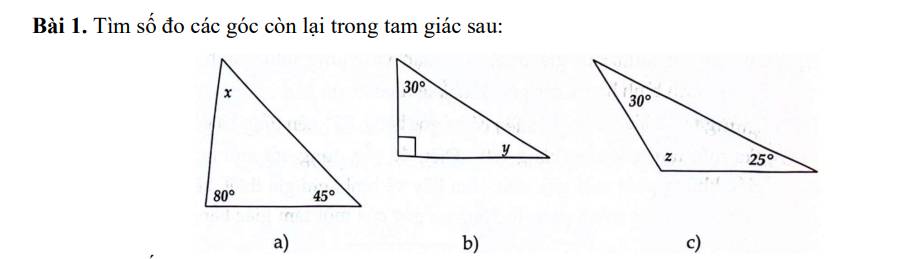
Áp dụng tính chất tổng 3 góc trong một tam giác bằng $180^0$
a.
$x=180^0-80^0-45^0=55^0$
b.
$y=180^0-30^0-90^0=60^0$
c.
$z=180^0-30^0-25^0=125^0$

Đổi 30 phút = 0,5 giờ
Quãng sông từ A đến B dài là:
\(x\) \(\times\) 0,5 + y \(\times\) 1 = 0,5\(x\) + y (km)
Kết luận Quãng đường từ A đên B dài: 0,5\(x\) + y (km)

Lời giải:
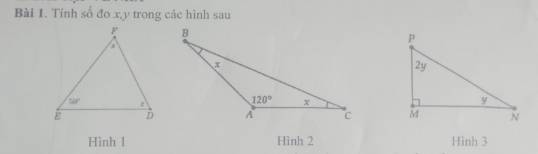
Áp dụng tính chất tổng 3 góc trong 1 tam giác bằng $180^0$
Hình 1: Hình không rõ ràng. Bạn xem lại.
Hình 2: $x+x+120^0=180^0$
$2x+120^0=180^0$
$2x=60^0$
$x=60^0:2=30^0$
Hình 3:
$2y+y+90^0=180^0$
$3y=180^0-90^0=90^0$
$y=90^0:3=30^0$
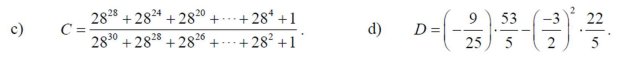
 Giúp mình với các bạn,mình cần gấp câu c1 và câu d2 ạ !
Giúp mình với các bạn,mình cần gấp câu c1 và câu d2 ạ !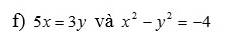


c; C = \(\dfrac{28^{28}+28^{24}+...+28^4+1}{28^{30}+28^{28}+...+28^2+1}\)
A = 1 + 284 + 288 + 2812 + ...2828
284A = 284 + 288 + 2812 + ... + 2828 + 2832
284A - A = 284+ 288+...+2828+ 2832- (1 + 284 + 288+...+2828)
(284 - 1)A = 284 + 288+ ...+ 2828 + 2832 - 1 - 284- ...- 2828
(284 - 1)A = (2832 - 1) + (284 - 284) + (288 - 288) + ... + (2828 - 2828)
(284 - 1)A = 2832 - 1 + 0 + 0... + 0
A = (2832 - 1): (284 - 1)
Đặt B = 2830 + 2828 + ... + 282 + 1
282B = 2832 + 2830 + ... + 284 + 282
282B - B = 2832 + 2830 + ... + 284 + 282 - (2830 + 2828 +...+1)
(282 - 1)B = 2832 + 2830+...+284 + 282 - 2830 - 2828 - ... 282- 1
(282 - 1)B = (2832 - 1) + (2830 - 2830) +...+(282 - 282)
(282 - 1)B = (2832 - 1) + 0 + 0 +...+ 0
(282 - 1)B = 2832 - 1
B = (2832 - 1): (282 - 1)
C = \(\dfrac{A}{B}\) = \(\dfrac{28^{32}-1}{28^4-1}\) : \(\dfrac{28^{32}-1}{28^2-1}\)
C = \(\dfrac{28^{32}-1}{28^4-1}\) \(\times\) \(\dfrac{28^2-1}{28^{32}-1}\)
C = \(\dfrac{28^2-1}{28^4-1}\)
C = \(\dfrac{1}{785}\)
Câu d:
\(\dfrac{x-1}{99}\) + \(\dfrac{x-2}{98}\) + \(\dfrac{x-3}{97}\) = \(\dfrac{x-4}{96}\) + \(\dfrac{x-5}{95}\) + \(\dfrac{x-6}{94}\)
(\(\dfrac{x-1}{99}\)-1)+(\(\dfrac{x-2}{98}\)-1)+(\(\dfrac{x-3}{97}\)-1) = (\(\dfrac{x-4}{96}\)-1) + (\(\dfrac{x-5}{95}\)-1)+(\(\dfrac{x-6}{94}\)-1)
\(\dfrac{x-100}{99}\)+\(\dfrac{x-100}{98}\)+\(\dfrac{x-100}{97}\) = \(\dfrac{x-100}{96}\)+\(\dfrac{x-100}{95}\)+\(\dfrac{x-100}{94}\)
\(\dfrac{x-100}{99}\)+\(\dfrac{x-100}{98}\)+\(\dfrac{x-100}{97}\)- \(\dfrac{x-100}{96}\)-\(\dfrac{x-100}{95}\)-\(\dfrac{x-100}{94}\) = 0
(\(x-100\)).(\(\dfrac{1}{99}\)+\(\dfrac{1}{98}\)+\(\dfrac{1}{97}\) - \(\dfrac{1}{96}\)-\(\dfrac{1}{95}\)-\(\dfrac{1}{94}\)) = 0
Vì\(\dfrac{1}{98}< \dfrac{1}{98}< \dfrac{1}{97}< \dfrac{1}{96}< \dfrac{1}{95}< \dfrac{1}{94}\)
Nên (\(\dfrac{1}{99}\) + \(\dfrac{1}{98}\) + \(\dfrac{1}{97}\) )- (\(\dfrac{1}{96}\) + \(\dfrac{1}{95}\) +\(\dfrac{1}{94}\) )< 0
⇒\(x-100\) = 0
Vậy \(x\) = 100