
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Ta có : |x - 2| ; |x - 5| ; |x - 18| ≥0∀x∈R≥0∀x∈R
=> |x - 2| + |x - 5| + |x - 18| ≥0∀x∈R≥0∀x∈R
=> D có giá trị nhỏ nhất khi x = 2;5;18
Mà x ko thể đồng thời nhận 3 giá trị
Nên GTNN của D là : 16 khi x = 5 ok nha bạn
x^2/x-1 = x^2-4x+4/x-1 + 4 = (x-2)^1/x-1 + 4 >= 4
Dấu "=" xảy ra <=> x-2 = 0 <=> x = 2 (tm)
Vậy GTNN của x^2/x-1 = 4 <=> x= 2
k mk nha

4: Đặt \(x=\dfrac{a+b}{a-b};y=\dfrac{b+c}{b-c};z=\dfrac{c+a}{c-a}\).
Ta có \(\left(x+1\right)\left(y+1\right)\left(z+1\right)=\dfrac{2a.2b.2c}{\left(a-b\right)\left(b-c\right)\left(c-a\right)}=\left(x-1\right)\left(y-1\right)\left(z-1\right)\)
\(\Rightarrow xy+yz+zx=-1\).
Bất đẳng thức đã cho tương đương:
\(x^2+y^2+z^2\ge2\Leftrightarrow\left(x+y+z\right)^2-2\left(xy+yz+zx\right)-2\ge0\Leftrightarrow\left(x+y+z\right)^2\ge0\) (luôn đúng).
Vậy ta có đpcm
mình xí câu 45,47,51 :>
45. a) Áp dụng bất đẳng thức Cauchy-Schwarz dạng Engel ta có :
\(\dfrac{1}{a}+\dfrac{2}{b}=\dfrac{1}{a}+\dfrac{4}{2b}\ge\dfrac{\left(1+2\right)^2}{a+2b}=\dfrac{9}{a+2b}\left(đpcm\right)\)
Đẳng thức xảy ra <=> a=b
b) Áp dụng bất đẳng thức Cauchy-Schwarz dạng Engel ta có :
\(\dfrac{1}{a}+\dfrac{1}{b}+\dfrac{1}{b}\ge\dfrac{\left(1+1+1\right)^2}{a+b+b}=\dfrac{9}{a+2b}\)(1)
\(\dfrac{1}{b}+\dfrac{1}{c}+\dfrac{1}{c}\ge\dfrac{\left(1+1+1\right)^2}{b+c+c}=\dfrac{9}{b+2c}\)(2)
\(\dfrac{1}{c}+\dfrac{1}{a}+\dfrac{1}{a}\ge\dfrac{\left(1+1+1\right)^2}{c+a+a}=\dfrac{9}{c+2a}\)(3)
Cộng (1),(2),(3) theo vế ta có đpcm
Đẳng thức xảy ra <=> a=b=c

A = - \(x^2\) - 4\(x\)
A = -(\(x^2\) + 4\(x\) + 4) + 4
A = -(\(x\) + 2)2 + 4
Vì (\(x\) + 2)2 ≥ 0 ⇒ -(\(x\) + 2)2 ≤ 0 ⇒ - (\(x\) + 2)2 + 4 ≤ 4
⇒ Amax = 4 ⇔ \(x\) + 2 = 0 ⇔ \(x\) = -2
Kết luận giá trị lớn nhất của A là 4 xảy ra khi \(x\) = -2
B = - 9\(x^2\) + 24\(x\) - 18
B = - (9\(x^2\) - 24\(x\) + 16) - 2
B = -(3\(x\) - 4)2 - 2
(3\(x\) - 4)2 ≥ 0 ⇒ -(3\(x\) - 4)2 ≤ 0 ⇒ -(3\(x\) - 4)2 - 2 ≤ -2
Bmax = -2 ⇔ 3\(x\) - 4 = 0 ⇔ \(x\) = \(\dfrac{4}{3}\)
Kết luận giá trị lớn nhất của B là: -2 xảy ra khi \(x\) = \(\dfrac{4}{3}\)

\(A=-x^2-4x\)
\(\Rightarrow A=-x^2-4x-4+4\)
\(\Rightarrow A=-\left(x^2+4x+4\right)+4\)
\(\Rightarrow A=-\left(x+2\right)^2+4\)
mà \(-\left(x+2\right)^2\le0,\forall x\)
\(\Rightarrow A=-\left(x+2\right)^2+4\le0+4=4\)
Vậy GTLN của A là 4
\(B=-9x^2+24x-18\)
\(\Rightarrow B=-9x^2+24x-16+16-18\)
\(\Rightarrow B=-\left(9x^2-24x+16\right)+16-18\)
\(\Rightarrow B=-\left(3x-4\right)^2-2\)
mà \(-\left(3x-4\right)^2\le0,\forall x\)
\(\Rightarrow B=-\left(3x-4\right)^2-2\le0-2=-2\)
Vậy GTLN của B là -2


a, ĐKXĐ:\(2x^3-2x^2\ne0\Rightarrow2x^2\left(x-1\right)\ne0\Rightarrow\left\{{}\begin{matrix}2x^2\ne0\\x-1\ne0\end{matrix}\right.\Rightarrow\left\{{}\begin{matrix}x\ne0\\x\ne1\end{matrix}\right.\)
b, \(A=\dfrac{5x^2-5x}{2x^3-2x^2}\)
\(\Rightarrow A=\dfrac{5x\left(x-1\right)}{2x^2\left(x-1\right)}\)
\(\Rightarrow A=\dfrac{5}{2x}\)
Để A=1\(\Rightarrow\dfrac{5}{2x}=1\)
\(\Rightarrow2x=5\\ \Rightarrow x=\dfrac{5}{2}\)
a, đk \(2x^2\left(x-1\right)\ne0\Leftrightarrow x\ne0;x\ne1\)
b, \(A=\dfrac{5x\left(x-1\right)}{2x^2\left(x-1\right)}=\dfrac{5}{2x}=1\Rightarrow5=2x\Leftrightarrow x=\dfrac{5}{2}\left(tm\right)\)

a)
\(\left|x\right|=2=>\left[{}\begin{matrix}x=2\\x=-2\left(loaividieukien\right)\end{matrix}\right.\)
thay x=2 vào biểu thức B ta có
\(\dfrac{2\cdot2+2}{2+2}=\dfrac{6}{4}=1,5\)
b)
\(\dfrac{x+1}{2x-2}+\dfrac{1}{2-2x^2}\\ =\dfrac{x+1}{2x-2}-\dfrac{1}{2x^2-2}\\ =\dfrac{x+1}{2\left(x-1\right)}-\dfrac{1}{2\left(x-1\right)\left(x+1\right)}\\ =\dfrac{\left(x+1\right)^2}{2\left(x-1\right)\left(x+1\right)}-\dfrac{1}{2\left(x-1\right)\left(x+1\right)}\\ =\dfrac{x^2+2x+1-1}{2\left(x-1\right)\left(x+1\right)}\\ =\dfrac{x^2+2x}{2\left(x-1\right)\left(x+1\right)}\\ =\dfrac{x\left(x+2\right)}{2\left(x-1\right)\left(x+1\right)}\)

\(\dfrac{4x+2}{4x-2}+\dfrac{3-6x}{6x-6}\left(dkxd:x\ne\dfrac{1}{2};x\ne1\right)\)
\(=\dfrac{2\left(2x+1\right)}{2\left(2x-1\right)}+\dfrac{3\left(1-2x\right)}{6\left(x-1\right)}\)
\(=\dfrac{2x+1}{2x-1}+\dfrac{1-2x}{2\left(x-1\right)}\)
\(=\dfrac{2x+1}{2x-1}+\dfrac{1-2x}{2x-2}\)
\(=\dfrac{\left(2x+1\right)\left(2x-2\right)}{\left(2x-1\right)\left(2x-2\right)}+\dfrac{\left(1-2x\right)\left(2x-1\right)}{\left(2x-1\right)\left(2x-2\right)}\)
\(=\dfrac{4x^2-2x-2}{\left(2x-1\right)\left(2x-2\right)}+\dfrac{-4x^2+4x-1}{\left(2x-1\right)\left(2x-2\right)}\)
\(=\dfrac{4x^2-2x-2-4x^2+4x-1}{\left(2x-1\right)\left(2x-2\right)}\)
\(=\dfrac{2x-3}{\left(2x-1\right)\left(2x-2\right)}\)
\(=\dfrac{2x-3}{4x^2-6x+2}\)

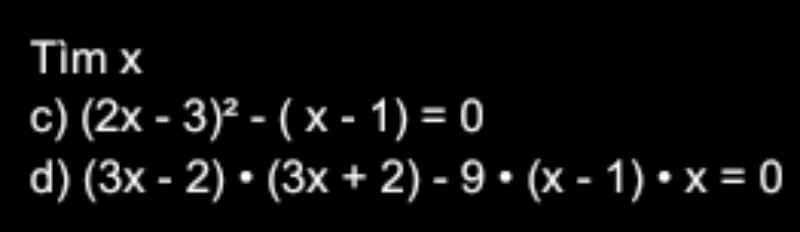


 mọi người giúp mình với mình đang cần gấp :(
mọi người giúp mình với mình đang cần gấp :( Mọi người giúp mình với ạ mình cần gấp!!!!!
Mọi người giúp mình với ạ mình cần gấp!!!!!

\(c)\left(2x-3\right)^2-\left(x-1\right)=0\\ \Leftrightarrow\left(4x^2-12x+9\right)-\left(x-1\right)=0\\ \Leftrightarrow4x^2-12x+9-x+1=0\\ \Leftrightarrow4x^2-13x+10=0\\ \Leftrightarrow\left(4x^2-8x\right)-\left(5x-10\right)=0\\ \Leftrightarrow4x\left(x-2\right)-5\left(x-2\right)=0\\ \Leftrightarrow\left(x-2\right)\left(4x-5\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x-2=0\\4x-5=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=2\\x=\dfrac{5}{4}\end{matrix}\right.\)
\(d)\left(3x-2\right)\cdot\left(3x+2\right)-9\left(x-1\right)\cdot x=0\\ \Leftrightarrow\left(3x\right)^2-2^2-9\left(x-1\right)x=0\\ \Leftrightarrow9x^2-4-9x^2+9x=0\\ \Leftrightarrow9x-4=0\\ \Leftrightarrow9x=4\\ \Leftrightarrow x=\dfrac{4}{9}\)