Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Đặt \(F=\sqrt{x\left(x+1\right)\left(x+2\right)\left(x+3\right)+5}\)
\(=\sqrt{\left(x^2+3x\right)\left(x^2+3x+2\right)+5}\left(#\right)\)
# đặt \(t=x^2+3x\) ta có
\(\left(#\right)=\sqrt{t\cdot\left(t+2\right)+5}=\sqrt{\left(t+1\right)^2+4}\)
(#) đạt giá trị nhỏ nhất của F=2 khi t+1=0 hay t=-1
Vậy \(F_{min}=2\) khi \(x=\frac{-3\pm\sqrt{5}}{2}\)

a: Ta có: \(\sqrt{x}\left(\sqrt{x}-3\right)-5\left(\sqrt{x}+3\right)\)
\(=x-3\sqrt{x}-5\sqrt{x}-15\)
\(=x-8\sqrt{x}-15\)
b: Ta có: \(3\left(\sqrt{x}+2\right)+\left(\sqrt{x}+3\right)\left(2-\sqrt{x}\right)\)
\(=3\sqrt{x}+6+2\sqrt{x}-x+6-3\sqrt{x}\)
\(=-x+2\sqrt{x}+12\)
c: Ta có: \(\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)-5\left(\sqrt{x}-1\right)\)
\(=x-9-5\sqrt{x}+5\)
\(=x-5\sqrt{x}-4\)
d: Ta có: \(3\left(\sqrt{x}-2\right)-\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)\)
\(=3\sqrt{x}-6-x+1\)
\(=-x+3\sqrt{x}-5\)

C=|x-2021|+|1-x|>=|x-2021+1-x|=2020
Dấu = xảy ra khi 1<=x<=2021

\(A=\)\(\sqrt{x+2\left(1+\sqrt{x+1}\right)}+\sqrt{x+2\left(1-\sqrt{x+1}\right)}\) (đk: \(x\ge-1\))
\(=\sqrt{\left(x+1\right)+2\sqrt{x+1}+1}+\sqrt{\left(x+1\right)-2\sqrt{x+1}+1}\)
\(=\sqrt{\left(\sqrt{x+1}+1\right)^2}+\sqrt{\left(\sqrt{x+1}-1\right)^2}\)
\(=\sqrt{x+1}+1+\left|\sqrt{x+1}-1\right|\)
\(=\left[{}\begin{matrix}\sqrt{x+1}+1+\sqrt{x+1}-1;\sqrt{x+1}\ge1\\\sqrt{x+1}+1-\left(\sqrt{x+1}-1\right);\sqrt{x+1}< 1\end{matrix}\right.\)
\(=\left[{}\begin{matrix}2\sqrt{x+1};x\ge0\\2;-1\le x< 0\end{matrix}\right.\)
Có \(2\sqrt{x+1}\ge2\) tại \(x\ge0\)
\(\Rightarrow\min\limits_{x\ge0}A=2\)
Dấu = xảy ra <=> x=0 mà tại \(-1\le x< 0\) thì A=2
Vậy giá trị nhỏ nhất của biểu thức là 2 tại x=0 hoặc \(-1\le x< 0\)
(Ủa đề zì kì)
\(ĐKXĐ:x\ge-1\)
Đặt \(A=\sqrt{x+2\left(1+\sqrt{x+1}\right)}+\sqrt{x+2\left(1-\sqrt{x+1}\right)}\)
\(=\sqrt{x+1+2\sqrt{x+1}+1}+\sqrt{x+1-2\sqrt{x+1}+1}\)
\(=\sqrt{\left(\sqrt{x+1}+1\right)^2}+\sqrt{\left(\sqrt{x+1}-1\right)^2}\)
\(=\left|\sqrt{x+1}+1\right|+\left|\sqrt{x+1}-1\right|\)
\(=\left|\sqrt{x+1}+1\right|+\left|1-\sqrt{x+1}\right|\)
\(\ge\left|\sqrt{x+1}+1+1-\sqrt{x+1}\right|=2\)
Dấu "=" xảy ra khi \(\left(\sqrt{x+1}+1\right)\left(1-\sqrt{x+1}\right)\ge0\)
\(\Leftrightarrow1-\sqrt{x+1}\ge0\)
\(\Leftrightarrow\sqrt{x+1}\le1\)
\(\Leftrightarrow x\le0\). Mà \(x\ge-1\) Nên \(-1\le x\le0\)
Vậy Min \(A=2\) khi \(-1\le x\le0\)

\(ĐKXĐ:\left\{{}\begin{matrix}x>0\\x\ne1\end{matrix}\right.\)
\(P\left(x\right)=\dfrac{x^2-\sqrt{x}}{x+\sqrt{x}+1}-\dfrac{2x+\sqrt{x}}{\sqrt{x}}+\dfrac{2\left(x-1\right)}{\sqrt{x}-1}\)
\(P\left(x\right)=\dfrac{\sqrt{x}\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}{\left(x+\sqrt{x}+1\right)}-\dfrac{\sqrt{x}\left(2\sqrt{x}+1\right)}{\sqrt{x}}+\dfrac{2\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}{\sqrt{x}-1}\)
\(P\left(x\right)=x-\sqrt{x}-2\sqrt{x}-2+2\sqrt{x}+2\)
\(P\left(x\right)=x-\sqrt{x}\)
Ta có : \(\dfrac{P\left(x\right)}{2020\sqrt{x}}=\dfrac{x-\sqrt{x}}{2020\sqrt{x}}=\dfrac{\sqrt{x}\left(\sqrt{x}-1\right)}{2020\sqrt{x}}=\dfrac{\sqrt{x}-1}{2020}\)
Để \(\dfrac{P\left(x\right)}{2020\sqrt{x}}min\Leftrightarrow\dfrac{\sqrt{x}-1}{2020}min\Leftrightarrow\sqrt{x}-1\) min (vì 2020 > 0)
Lại có : \(\sqrt{x}-1\ge-1\forall x\)
Dấu "=" xảy ra <=> x = 0
Vậy Min\(\dfrac{P\left(x\right)}{2020\sqrt{x}}=\dfrac{-1}{2020}\Leftrightarrow x=0\)

a. ĐKXĐ \(x\ge0\)và \(x\ne9\)
Ta có \(K=\left(\frac{2\sqrt{x}}{\sqrt{x}+3}+\frac{\sqrt{x}}{\sqrt{x}-3}-\frac{3\left(\sqrt{x}+3\right)}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}\right):\left(\frac{2\sqrt{x}-2}{\sqrt{x}-3}-1\right)\)
\(=\frac{2\sqrt{x}\left(\sqrt{x}-3\right)+\sqrt{x}\left(\sqrt{x}+3\right)-3\sqrt{x}-9}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}:\frac{2\sqrt{x}-2-\sqrt{x}+3}{\sqrt{x}-3}\)
\(=\frac{2x-6\sqrt{x}+x+3\sqrt{x}-3\sqrt{x}-9}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}:\frac{\sqrt{x}+1}{\sqrt{x}-3}\)
\(=\frac{3x-6\sqrt{x}-9}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}.\frac{\sqrt{x}-3}{\sqrt{x}+1}=\frac{3\left(x-2\sqrt{x}-3\right)}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}.\frac{\sqrt{x}-3}{\sqrt{x}+1}\)
\(=\frac{3\left(\sqrt{x}+1\right)\left(\sqrt{x}-3\right)}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}.\frac{\sqrt{x}-3}{\sqrt{x}+1}=\frac{3\left(\sqrt{x}-3\right)}{\sqrt{x}+3}\)
b. Để \(K< -1\Rightarrow\frac{3\sqrt{x}-9+\sqrt{x}+3}{\sqrt{x}+3}< 0\Rightarrow\frac{4\sqrt{x}-6}{\sqrt{x}+3}< 0\Rightarrow4\sqrt{x}-6< 0\)vì \(\sqrt{x}+3\ge3\)
\(\Rightarrow0\le x< \frac{9}{4}\left(tm\right)\)
Vậy với \(0\le x< \frac{9}{4}\)thì K<-1
c. \(K=\frac{3\sqrt{x}-9}{\sqrt{x}+3}=3+\frac{-18}{\sqrt{x}+3}\)
Ta có \(\sqrt{x}+3\ge3\Rightarrow\frac{1}{\sqrt{x}+3}\le\frac{1}{3}\Rightarrow-\frac{18}{\sqrt{x}+3}\ge-6\Rightarrow3+\frac{-18}{\sqrt{x}+3}\ge-3\)
\(\Rightarrow K\ge-3\)
Vậy \(MinK=-3\Leftrightarrow\sqrt{x}=0\Leftrightarrow x=0\)

\(F=\sqrt{x\left(x+1\right)\left(x+2\right)\left(x+3\right)+5}=\sqrt{\left(x^2+3x\right)\left(x^2+3x+2\right)+5}\) ( * )
*Đặt \(t=x^2+3x\)Ta có :
( * ) \(=\sqrt{t.\left(t+2\right)+5}=\sqrt{\left(t+1\right)^2+4}\)
( * ) Đạt GTNN của F khi bằng 2 khi \(t+1=0\) hay \(t=-1\)
Vậy \(^{minF=2\Leftrightarrow x=\frac{-3\pm\sqrt{5}}{2}}\)
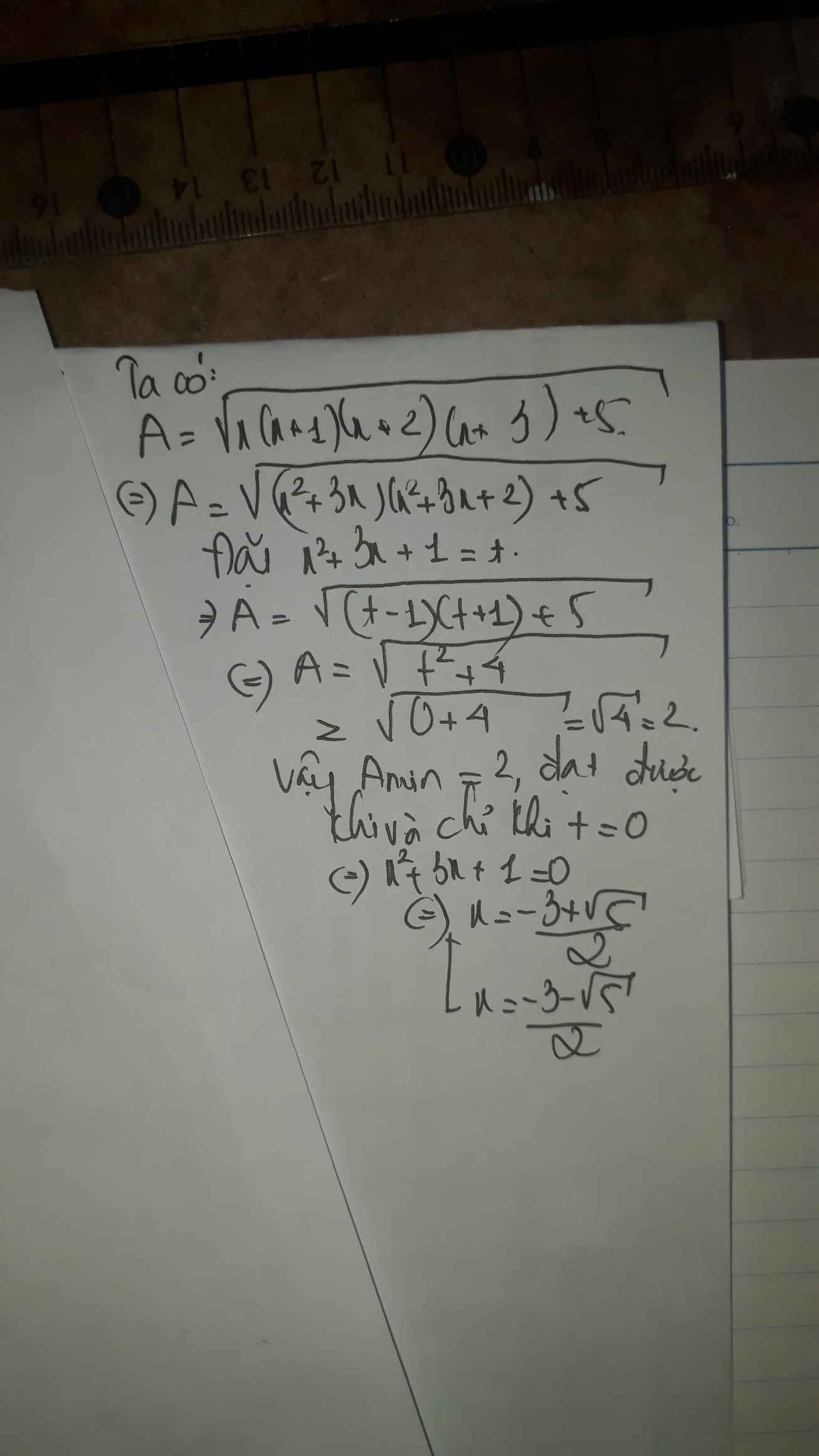

Ta có: x(x+1)(x+2)(x+3)+5
= x(x+3)(x+1)(x+2)+5 = (x2 +3x)(x2 +3x +2)+5 = (x2 +3x +1 -1)(x2 +3x +1 +1) +5
= (x2 +3x +1)2 -1 +5 = (x2 +3x +1)2 +4
Vì (x2 +3x +1)2 ≥0 (∀x) nên (x2 +3x +1) +4 ≥4
⇒ C ≥ √4 ⇒ C ≥ 2
⇒ C đạt GTNN bằng 2 ⇔ (x2 +3x +1)2 =0
Bạn làm tiếp nha......
Phan Văn KhởiPhùng Khánh LinNguyễn Thị Ngọc ThơhHoàng Ngọc Anh giup mk voi