
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a: \(\Leftrightarrow4^x\left(\dfrac{3}{2}+\dfrac{5}{3}\cdot4^2\right)=4^8\left(\dfrac{3}{2}+\dfrac{5}{3}\cdot4^2\right)\)
=>4^x=4^8
=>x=8
b: \(\Leftrightarrow2^x\cdot\dfrac{1}{2}+2^x\cdot2=2^{10}\left(2^2+1\right)\)
=>2^x=2^11
=>x=11
c: =>1/6*6^x+6^x*36=6^15(1+6^3)
=>6^x=6*6^15
=>x=16
d: \(\Leftrightarrow8^x\left(\dfrac{5}{3}\cdot8^2-\dfrac{3}{5}\right)=8^9\left(\dfrac{5}{3}\cdot8^2-\dfrac{3}{5}\right)\)
=>x=9

\(M\left(x\right)=x^4+\frac{11}{2}x^2+x+6=\left(x^4+\frac{9}{2}x^2+\frac{81}{16}\right)+\left(x^2+x+\frac{1}{4}\right)+\frac{11}{16}\)
=> \(M\left(x\right)=\left(x^4+2.\frac{9}{4}x^2+\left(\frac{9}{4}\right)^2\right)+\left(x^2+2.\frac{1}{2}x+\left(\frac{1}{2}\right)^2\right)+\frac{11}{16}\)
=> \(M\left(x\right)=\left(x^2+\frac{9}{4}\right)^2+\left(x+\frac{1}{2}\right)^2+\frac{11}{16}\)
Nhận thấy: Do \(\left(x^2+\frac{9}{4}\right)^2>0;\left(x+\frac{1}{2}\right)^2\ge0\)Với mọi x
=> \(M\left(x\right)>\frac{11}{16}\) với mọi x
=> Đa thức M(x) vô nghiệm (không có nghiệm)

1,
\(\left(2x+1\right)^3=-0,001\\ \left(2x+1\right)^3=\left(-0.1\right)^3\\ \Leftrightarrow2x+1=-0.1\\ 2x=-1.1\\ x=-\dfrac{11}{10}:2\\ x=-\dfrac{11}{20}\\ Vậy...\)
2,
\(\left(2x-3\right)^4=\left(2x-3\right)^6\\ \Leftrightarrow\left(2x-3\right)^6-\left(2x-3\right)^4=0\\ \Leftrightarrow\left(2x-3\right)^4\cdot\left[\left(2x-3\right)^2-1\right]=0\\ \Rightarrow\left\{{}\begin{matrix}\left(2x-3\right)^4=0\\\left(2x-3\right)^2-1=0\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}2x-3=0\\\left(2x-3\right)^2=1\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}2x=3\\2x-3=1\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{3}{2}\\x=2\end{matrix}\right.\\ Vậyx\in\left\{\dfrac{3}{2};2\right\}\)
3, Làm tương tự câu 2
5,
\(9^x:3^x=3\\ \left(9:3\right)^x=3\\ 3^x=3\\ \Rightarrow x=1\\ Vậy...\)
6,
\(3^x+3^{x+3}=756\\ 3^x+3^x\cdot3^3\\ 3^x\cdot\left(1+27\right)=756\\ 3^x\cdot28=756\\ \Leftrightarrow3^x=27\\ 3^x=3^3\\ \Rightarrow x=3\\ vậy...\)
7,
\(5^{x+1}+6\cdot5^{x+1}=875\\ 5^{x+1}\cdot\left(1+6\right)=875\\ 5^{x+1}\cdot7=875\\ \Leftrightarrow5^{x+1}=125\\ \Leftrightarrow5^{x+1}=5^3\Leftrightarrow x+1=3\\ \Rightarrow x=2\\ Vậy...\)
9,

1) Tính
a) 253 : 52 = (52)3 : 52 = 56 : 52 = 54 = 625
\(b)\left(\dfrac{3}{7}\right)^{21}:\left(\dfrac{9}{49}\right)^6=\left(\dfrac{3}{7}\right)^{21}:\left[\left(\dfrac{3}{7}\right)^2\right]^6=\left(\dfrac{3}{7}\right)^{21}:\left(\dfrac{3}{7}\right)^{12}=\left(\dfrac{3}{7}\right)^9\) d) 9 . 32 . \(\dfrac{1}{81}\) . 32 = 32 . 32 . \(\dfrac{1}{3^4}\) . 32 = 9
2) Tìm x thuộc Q, biết:
a) 3x + 2 = 27
=> 3x + 2 = 33
x + 2 = 3
x = 3 - 2
x = 1
b) \(\left(\dfrac{1}{2}x-3\right)^4=81\)
\(\Rightarrow\left(\dfrac{1}{2}x-3\right)^4=3^4\)
\(\dfrac{1}{2}x-3=3^{ }\)
\(\dfrac{1}{2}x=3+3\)
\(\dfrac{1}{2}x=9\)
\(x=9:\dfrac{1}{2}\)
\(x=18\)
c) \(\left(x-\dfrac{1}{2}\right)^3=-27\)
\(\Rightarrow\left(x-\dfrac{1}{2}\right)^3=\left(-3\right)^3\)
\(x-\dfrac{1}{2}=-3\)
\(x=-3+\dfrac{1}{2}\)
\(x=\dfrac{-5}{2}\)
d) 5 . 5x + 1 = 125
5x + 1 = 125 : 5
5x + 1 = 25
5x + 1 = 52
x + 1 = 2
x = 2 - 1
x = 1.

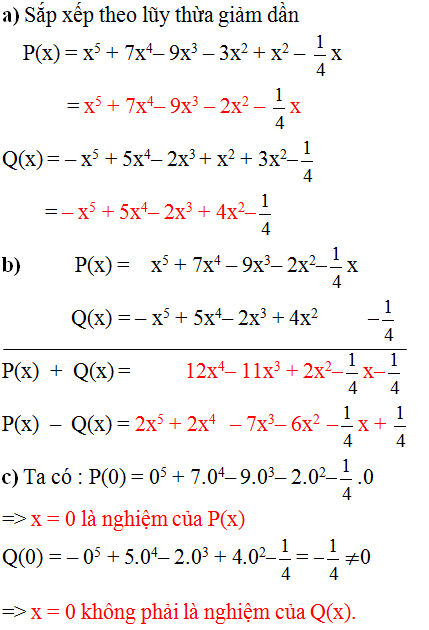
a. P(x)+Q(x)=(3x4 + x3- x2- \(\dfrac{1}{4}\)x)+(3x4- 4x3+x2-\(\dfrac{1}{4}\))=6x4-3x3+\(\dfrac{1}{2}\)
Tương tự làm P(x)-Q(X) nhé !!!
b. Thay x = 0 vào đa thức P(x) ta có :
.....................................................
thay x = 0 vào đa thức Q(x) ta có:
......................................................
=> đpcm

Câu 1 :
\(\text{a) }B=\dfrac{4^6\cdot9^5+6^9\cdot120}{8^4\cdot3^{12}-6^{11}}\\ B=\dfrac{\left(2^2\right)^6\cdot\left(3^2\right)^5+\left(2\cdot3\right)^9\cdot\left(2^3\cdot3\cdot5\right)}{\left(2^3\right)^4\cdot3^{12}-6^{11}}\\ B=\dfrac{2^{12}\cdot3^{10}+2^9\cdot3^9\cdot2^3\cdot3\cdot5}{2^{12}\cdot3^{12}-\left(2\cdot3\right)^{11}}\\ B=\dfrac{2^{12}\cdot3^{10}+2^{12}\cdot3^{10}\cdot5}{2^{12}\cdot3^{12}-2^{11}\cdot3^{11}}\\ B=\dfrac{2^{12}\cdot3^{10}\left(1+5\right)}{2^{11}\cdot3^{11}\left(6-1\right)}\\ B=\dfrac{2\cdot6}{3\cdot5}\\ B=\dfrac{4}{5}\\ \)
\(\text{b) }C=\dfrac{5\cdot4^{15}\cdot9^9-4\cdot3^{20}\cdot8^9}{5\cdot2^9\cdot6^{19}-7\cdot2^{29}\cdot27^6}\\ C=\dfrac{5\cdot\left(2^2\right)^{15}\cdot\left(3^2\right)^9-2^2\cdot3^{20}\cdot\left(2^3\right)^9}{5\cdot2^9\cdot\left(2\cdot3\right)^{19}-7\cdot2^{29}\cdot\left(3^3\right)^6}\\ C=\dfrac{5\cdot2^{30}\cdot3^{18}-2^2\cdot3^{20}\cdot2^{27}}{5\cdot2^9\cdot2^{19}\cdot3^{19}-7\cdot2^{29}\cdot3^{18}}\\ C=\dfrac{5\cdot2^{30}\cdot3^{18}-2^{29}\cdot3^{20}}{5\cdot2^{28}\cdot3^{19}-7\cdot2^{29}\cdot3^{18}}\\ C=\dfrac{2^{29}\cdot3^{18}\left(10-9\right)}{2^{28}\cdot3^{18}\left(15-14\right)}\\ C=\dfrac{2^{29}\cdot3^{18}}{2^{28}\cdot3^{18}}\\ C=2\\ \)
\(\text{c) }D=\dfrac{49^{24}\cdot125^{10}\cdot2^8-5^{30}\cdot7^{49}\cdot4^5}{5^{29}\cdot16^2\cdot7^{48}}\\ D=\dfrac{\left(7^2\right)^{24}\cdot\left(5^3\right)^{10}\cdot2^8-5^{30}\cdot7^{49}\cdot\left(2^2\right)^5}{5^{29}\cdot\left(2^4\right)^2\cdot7^{48}}\\ D=\dfrac{7^{48}\cdot5^{30}\cdot2^8-5^{30}\cdot7^{49}\cdot2^{10}}{5^{29}\cdot2^8\cdot7^{48}}\\ D=\dfrac{7^{48}\cdot5^{30}\cdot2^8\left(1-28\right)}{5^{29}\cdot2^8\cdot7^{48}}\\ D=5\cdot\left(-27\right)\\ D=-135\)
Câu 2 :
\(\text{a) }9^{x+1}-5\cdot3^{2x}=324\\ \Leftrightarrow9^x\cdot9-5\cdot9^x=81\cdot4\\ \Leftrightarrow9^x\left(9-5\right)=9^2\cdot4\\ \Leftrightarrow9^x\cdot4=9^2\cdot4\\ \Leftrightarrow9^x=9^2\\ \Leftrightarrow x=2\\ \text{Vậy }x=2\\ \)
Sorry . Mình chỉ biết đến đây thôi

Chạy tiếp sức
(a) bản chất chỉ là bước đệm để làm câu (b)
b)
lấy kq câu (a) của @ trước đó
\(M\left(x\right)=x^4+\dfrac{21}{4}x^2+\dfrac{1}{4}x^2+x+1+5\)
\(M\left(x\right)=x^4+\dfrac{21}{4}x^2+\left(\dfrac{x}{2}+1\right)^2+5\)
\(\left\{{}\begin{matrix}x^4\ge0\forall x\\\dfrac{21}{4}x^2\ge0\forall x\\\left(\dfrac{x}{2}+1\right)^2\ge0\forall x\\\end{matrix}\right.\) =>\(M\left(x\right)=x^4+\dfrac{21}{4}x^2+\left(\dfrac{x}{2}+1\right)^2+5>0\)với mọi x => M(x) không có nghiệm=> Nếu (a) đúng => dpcm
a) \(M\left(x\right)=P\left(x\right)+Q\left(x\right)\)
\(=x^4-5x+2x^2+1+5x+3x^2+5+\dfrac{1}{2}x^2+x\\ =x^4+\dfrac{11}{2}x^2+x+6\)

a.
| x | = 5,6
=>\(\left[{}\begin{matrix}x=5,6\\x=-5,6\end{matrix}\right.\)
Vậy \(x\in\left\{-5,6;5,6\right\}\)
b, \(\left|x-3,5\right|=5\)
=>\(\left[{}\begin{matrix}x-3,5=5\\x-3,5=-5\end{matrix}\right.\)
=>\(\left[{}\begin{matrix}x=8,5\\x=-1,5\end{matrix}\right.\)
Vậy \(x\in\left\{-1,5;8,5\right\}\)
c,\(\left|x-\dfrac{3}{4}\right|-\dfrac{1}{2}=0\)
=> \(\left|x-\dfrac{3}{4}\right|=\dfrac{1}{2}\)
=>\(\left[{}\begin{matrix}x-\dfrac{3}{4}=\dfrac{1}{2}\\x-\dfrac{3}{4}=-\dfrac{1}{2}\end{matrix}\right.\)
=> \(\left[{}\begin{matrix}x=\dfrac{5}{4}\\x=\dfrac{1}{4}\end{matrix}\right.\)
Vậy \(x\in\left\{\dfrac{1}{4};\dfrac{5}{4}\right\}\)
d,\(\left|4x\right|-\left(\left|-13,5\right|\right)=\left|\dfrac{1}{4}\right|\)
=> \(\left|4x\right|-13,5=\dfrac{1}{4}\)
=> \(\left|4x\right|=13,75\)
=>\(\left[{}\begin{matrix}4x=13,75\\4x=-13,75\end{matrix}\right.\)
=>\(\left[{}\begin{matrix}x=3,4375\\x=-3,4375\end{matrix}\right.\)
Vậy \(x\in\left\{-3,4375;3,4375\right\}\)
e, ( x - 1 ) 3 = 27
=> x - 1 = 3
=> x = 4
Vậy x = 4
f, ( 2x - 3)2 = 36
=> \(\left[{}\begin{matrix}2x-3=6\\2x-3=-6\end{matrix}\right.\)
=> \(\left[{}\begin{matrix}x=4,5\\x=-1,5\end{matrix}\right.\)
Vậy x\(\in\left\{-1,5;4,5\right\}\)
g, \(5^{x+2}=625\)
=> \(5^{x+2}=5^4\)
=> x + 2 = 4
=> x = 2
Vậy x = 2
h, ( 2x - 1)3 = -8
=> 2x - 1 = -2
=> x = \(\dfrac{-1}{2}\)
Vậy x = \(\dfrac{-1}{2}\)
i, \(\dfrac{1}{4}.\dfrac{2}{6}.\dfrac{3}{8}.\dfrac{4}{10}.\dfrac{5}{12}...\dfrac{30}{62}.\dfrac{31}{64}=2^x\)
=> \(\dfrac{1.2.3.4.5...30.31}{4.6.8.10.12...62.64}=2^x\)
=>\(\dfrac{1.2.3.4.5...30.31}{\left(2.3.4.5...30.31.32\right)\left(2.2.2.2...2.2_{ }\right)}=2^x\)(có 31 số 2)
=> \(\dfrac{1}{32.2^{31}}=2^x\)
=> \(\dfrac{1}{2^{36}}=2^x\)
=> x = -36
Vậy x = -36

II: Tự luận
Câu 1:
a: Ta có: \(\dfrac{-5}{3}x^2+\dfrac{3}{5}=0\)
\(\Leftrightarrow-\dfrac{5}{3}x^2=-\dfrac{3}{5}\)
\(\Leftrightarrow x^2=\dfrac{9}{25}\)
=>x=3/5 hoặc x=-3/5
b: Ta có: \(3x^3+x^2=0\)
\(\Leftrightarrow x^2\left(3x+1\right)=0\)
=>x=0 hoặc x=-1/3
c: Ta có: \(x^2-4x+3=0\)
=>(x-1)(x-3)=0
=>x=1 hoặc x=3

Ta có: \(\dfrac{a}{2}=\dfrac{b}{3}=\dfrac{c}{4}\Rightarrow\left(\dfrac{a}{2}\right)^2=\left(\dfrac{b}{3}\right)^2=\left(\dfrac{c}{4}\right)^2\)
\(\Rightarrow\dfrac{a^2}{4}=\dfrac{b^2}{9}=\dfrac{z^2}{16}\)\(\Rightarrow\dfrac{a^2}{4}=\dfrac{b^2}{9}=\dfrac{2c^2}{32}\)
Áp dụng tính chất của dãy tỉ số bằng nhau, ta có:
\(\dfrac{a^2}{4}=\dfrac{b^2}{9}=\dfrac{2c^2}{32}=\dfrac{a^2+b^2-2c^2}{4+9-32}=\dfrac{-76}{-19}=4\)
\(\Rightarrow\dfrac{a^2}{4}=4\Rightarrow a=4\)
\(\dfrac{b^2}{9}=4\Rightarrow b=6\)
\(\dfrac{2c^2}{32}=4\Rightarrow2c^c=128\Rightarrow c=8\)
Vậy \(\left\{{}\begin{matrix}a=4\\b=6\\c=8\end{matrix}\right.\)
a = 4 hoặc a = -4
b = 6 hoặc b = -6
c = 8 hoặc c = -8
\(x^4+\dfrac{11}{2}x^2+x+6=\left(x^4+4x^2+4\right)+\dfrac{1}{2}\left(x^2+2x+1\right)+\dfrac{1}{2}x^2+\dfrac{3}{2}\)
\(=\left(x^2+2\right)^2+\dfrac{1}{2}\left(x+1\right)^2+\dfrac{1}{2}x^2+\dfrac{3}{2}\)
Do \(\left\{{}\begin{matrix}\left(x^2+2\right)^2>0\\\dfrac{1}{2}\left(x+1\right)^2\ge0\\\dfrac{1}{2}x^2\ge0\end{matrix}\right.\) với mọi x
\(\Rightarrow\left(x^2+2\right)^2+\dfrac{1}{2}\left(x+1\right)^2+\dfrac{1}{2}x^2+\dfrac{3}{2}>0\) với mọi x
Vậy đa thức không có nghiệm