PL
1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
ND
0
IM
3 tháng 8 2016
\(4x^2+3xy-11y^2=5x^2-x^2-2xy+5xy-10y^2-y^2\)
\(=5\left(x^2+xy+2y^2\right)-\left(x^2+2xy+y^2\right)=5\left(x^2+xy+2y^2\right)-\left(x+y\right)^2\)
Ta có \(4x^2+3xy-11y^2\) chia hết cho 5
=> \(\left(x+y\right)^2\) chia hết cho 5
Mà 5 là số nguyên tố
=> x+y chia hết cho 5
Mặt khác
\(x^4-y^4=\left(x^2-y^2\right)\left(x^2+y^2\right)=\left(x+y\right)\left(x-y\right)\left(x^2+y^2\right)\)
=> \(x^4-y^4\) chia hết cho 5 (đpcm)
11 tháng 8 2016
bạn đăng vừa thôi nhé chứ đăng nhiều thế này ít người khiên trì giải hết lắm bạn nên đăng từng bài cho đỡ dài
PT
17 tháng 9 2018
Ta có:
\(\left(2n-1\right)^3-\left(2n-1\right)\)
\(=\left(2n-1\right)\left[\left(2n-1\right)^2-1\right]\)
\(=\left(2n-1\right)\left(2n-1+1\right)\left(2n-1-1\right)\)
\(=\left(2n-1\right).2n.\left(2n-2\right)\)
\(=4n\left(2n-1\right)\left(n-1\right)\)
Vì \(4n\left(2n-1\right)\left(n-1\right)\) chia hết cho 4 ( Do chứa thừa số 4 )
Đồng thời \(4n\left(2n-1\right)\left(n-1\right)\) chia hết cho 2 ( Do n(n-1) là tích của hai số tự nhiên liên tiếp
\(\Rightarrow4n\left(2n-1\right)\left(n-1\right)\) chia hết cho 8
29 tháng 7 2016
(2n-1)^3-(2n-1)
=(2n-1)((2n-1)2-1)
=(2n-1)(2n-1+1)(2n-1-1)
=2n(2n-1)(2n-2)
=4n(2n-1)(n-1)
=> 4n(2n-1)(n-1) chia hết cho 4 (1)
mà (2n-1)(n-1)=(n+n-1)(n-1)
=> (2n1)(n-1) chia hết cho 2 (2)
Từ (1) và (2), ta suy ra (2n-1)^3 - (2n-1) chia hết cho 8
3 tháng 9 2018
\(C=x^{14}-10x^{13}+10x^{12}-10x^{11}+...+10x^2-10x+10\)
\(=x^{14}-\left(x+1\right)x^{13}+\left(x+1\right)x^{12}-\left(x+1\right)x^{11}+..+\left(x+1\right)x^2-\left(x+1\right)x+x+1\)
\(=x^{14}-x^{14}-x^{13}+x^{13}+x^{12}-x^{12}-x^{11}+...+x^3+x^2-x^2-x+x+1\)
\(=1\)
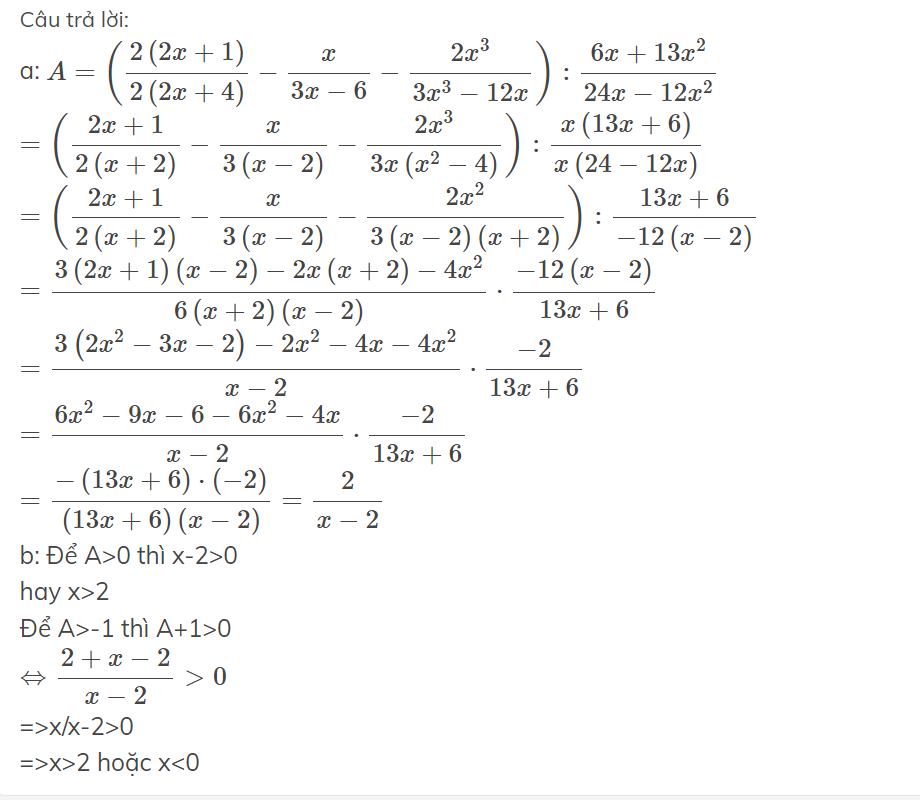
\(\text{ Dễ thấy: }120=2^3.3.5\)
\(\text{ Ta có: }x^5+10x^4+35x^3+50x^2+24x.\)
\(=x\left(x^4+10x^3+35x^2+50x+24\right)\)
\(=x\left[x^3\left(x+1\right)+9x^2\left(x+1\right)+26x\left(x+1\right)+24\left(x+1\right)\right]\)
\(=x\left(x+1\right)\left(x^3+9x^2+26x+24\right)\)
\(=x\left(x+1\right)\left[x^2\left(x+2\right)+7x\left(x+2\right)+12\left(x+2\right)\right]\)
\(=x\left(x+1\right)\left(x+2\right)\left(x^2+7x+12\right)\)
\(=x\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)\left(\text{ lm hơi tắt thông cảm!!}\right)\)
\(\Rightarrow x\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)⋮2\)
\(\Rightarrow x\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)⋮3\)
\(\Rightarrow x\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)⋮4\)
\(\Rightarrow x\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)⋮5\)
Vì 2,3,5 là các số nguyên tố cùng nhau nên
\(x\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)⋮2.3.4.5=120\)