Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a: \(VT=\dfrac{3\sqrt{6}}{2}+\dfrac{2\sqrt{6}}{3}-\dfrac{4\sqrt{6}}{2}\)
\(=\dfrac{-\sqrt{6}}{2}+\dfrac{2\sqrt{6}}{3}=\dfrac{-3\sqrt{6}+4\sqrt{6}}{6}=\dfrac{\sqrt{6}}{6}\)
b: \(VT=\dfrac{\left(\sqrt{6x}+\dfrac{\sqrt{6x}}{3}+\sqrt{6x}\right)}{\sqrt{6x}}\)
\(=1+\dfrac{1}{3}+1=2\dfrac{1}{3}\)

1) Để biểu thức \(\sqrt{-2x+3}\) xác định thì \(-2x+3\ge0\Leftrightarrow-2x\ge-3\Leftrightarrow x\le\dfrac{3}{2}\)
2) Để biểu thức \(\sqrt{\dfrac{2}{x^2}}\) xác định thì \(\left\{{}\begin{matrix}x^2\ge0\\x^2\ne0\end{matrix}\right.\)\(\Leftrightarrow\)\(x\ne0\)
3) Để biểu thức \(\sqrt{\dfrac{4}{x+3}}\) xác định thì \(\left\{{}\begin{matrix}x+3\ge0\\x+3\ne0\end{matrix}\right.\)\(\Leftrightarrow\)\(\left\{{}\begin{matrix}x\ge-3\\x\ne-3\end{matrix}\right.\)\(\Leftrightarrow x>-3\)
4) Ta có -5<0
x2+6>0
Suy ra \(\dfrac{-5}{x^2+6}< 0\)
Vậy với mọi x thì \(\sqrt{\dfrac{-5}{x^2+6}}\) sẽ không xác định
5) Để biểu thức \(\sqrt{3x+4}\) xác định thì \(3x+4\ge0\Leftrightarrow3x\ge-4\Leftrightarrow x\ge\dfrac{-4}{3}\)
6) Ta có \(x^2\ge0\Leftrightarrow x^2+1\ge1>0\)
Vậy với mọi x thì biểu thức \(\sqrt{1+x^2}\) sẽ luôn xác định
7) Để biểu thức \(\sqrt{\dfrac{3}{1-2x}}\) xác định thì \(\left\{{}\begin{matrix}1-2x\ge0\\1-2x\ne0\end{matrix}\right.\)\(\Leftrightarrow\)\(\left\{{}\begin{matrix}2x\le1\\2x\ne1\end{matrix}\right.\)\(\Leftrightarrow\)\(\left\{{}\begin{matrix}x\le\dfrac{1}{2}\\x\ne\dfrac{1}{2}\end{matrix}\right.\)\(\Leftrightarrow x< \dfrac{1}{2}\)
8) Để biểu thức \(\sqrt{\dfrac{-3}{3x+5}}\) xác định thì \(\left\{{}\begin{matrix}3x+5\le0\\3x+5\ne0\end{matrix}\right.\)\(\Leftrightarrow\)\(\left\{{}\begin{matrix}3x\le-5\\3x\ne-5\end{matrix}\right.\)\(\Leftrightarrow\)\(\left\{{}\begin{matrix}x\le\dfrac{-5}{3}\\x\ne\dfrac{-5}{3}\end{matrix}\right.\)\(\Leftrightarrow x< \dfrac{-5}{3}\)

Bài 2
\(P=\frac{2\sqrt{3+\sqrt{5-\sqrt{13+\sqrt{48}}}}}{\sqrt{6}+\sqrt{2}}\)
\(=\frac{2\sqrt{3+\sqrt{5-\sqrt{12+2\sqrt{12}+1}}}}{\sqrt{6}+\sqrt{2}}\)
\(=\frac{2\sqrt{3+\sqrt{5-\sqrt{\left(\sqrt{12}+1\right)^2}}}}{\sqrt{6}+\sqrt{2}}\)
\(=\frac{2\sqrt{3+\sqrt{5-\sqrt{12}-1}}}{\sqrt{6}+\sqrt{2}}\)
\(=\frac{2\sqrt{3+\sqrt{4-\sqrt{12}}}}{\sqrt{6}-\sqrt{2}}\)
\(=\frac{2\sqrt{3+\sqrt{3-2\sqrt{3}+1}}}{\sqrt{6}+\sqrt{2}}\)
\(=\frac{2\sqrt{3+\sqrt{\left(\sqrt{3}-1\right)^2}}}{\sqrt{6}+\sqrt{2}}\)
\(=\frac{2\sqrt{3+\sqrt{3}-1}}{\sqrt{6}+\sqrt{2}}\)
\(=\frac{\sqrt{2}\cdot\sqrt{2}\cdot\sqrt{2+\sqrt{3}}}{\sqrt{2}\left(\sqrt{3}+1\right)}\)
\(=\frac{\sqrt{2}\cdot\sqrt{4+2\sqrt{3}}}{\sqrt{2}\left(\sqrt{3}+1\right)}\)
\(=\frac{\sqrt{3+2\sqrt{3}+1}}{\left(\sqrt{3}+1\right)}\)
=\(\frac{\sqrt{\left(\sqrt{3}+1\right)^2}}{\left(\sqrt{3}+1\right)}\)
\(=\frac{\sqrt{3}+1}{\left(\sqrt{3}+1\right)}=1\)
Vậy P là một số nguyên

a) Vì biểu thức \(\sqrt{\dfrac{-5}{x^2+6}}\)có -5<0 nên làm cho cả phân số âm
Từ đó suy ra căn thức vô nghiệm
Vậy không có giá trị nào của x để biểu thức trên xác định
b) \(\sqrt{\left(x-1\right)\left(x-3\right)}\)
Để biểu thức trên xác định thì chia ra 4 TH (vì để xác định thì cả x-1 và x-3 cùng dương hoặc cùng âm)
\(\left[\begin {array} {} \begin{cases} x-1\geq0\\ x-3\geq0 \end{cases} \Leftrightarrow \begin{cases} x\geq1\\ x\geq3 \end{cases} \Rightarrow x\geq3 \\ \begin{cases} x-1\leq0\\ x-3\leq0 \end{cases} \Leftrightarrow \begin{cases} x\leq1\\ x\leq3 \end{cases} \Rightarrow x\leq1 \end{array} \right.\)
c) \(\sqrt{x^2-4}\) \(\Leftrightarrow\)\(\sqrt{\left(x-2\right)\left(x+2\right)}\)
Rồi làm như câu b
d) \(\sqrt{\dfrac{2-x}{x+3}}\)
Để biểu thức trên xác định thì
\(\begin{cases}2-x\ge0\\x+3>0\end{cases}\Leftrightarrow\begin{cases}x\ge2\\x>-3\end{cases}\) \(\Rightarrow\) \(x\ge2\) hoặc \(x>-3\)
e) Ở các biểu thức sau này nếu chỉ có căn thức có ẩn và + (hoặc trừ) với 1 số thì chỉ cần biến đổi cái có ẩn còn cái số thì kệ xác nó đi ![]() )
)
\(\sqrt{x^2-3x}\Leftrightarrow\sqrt{x\left(x-3\right)}\)
Để biểu thức trên xác định thì \(x\ge0\) và \(x-3\ge0\Leftrightarrow x\ge3\)
Bữa sau mình làm tiếp
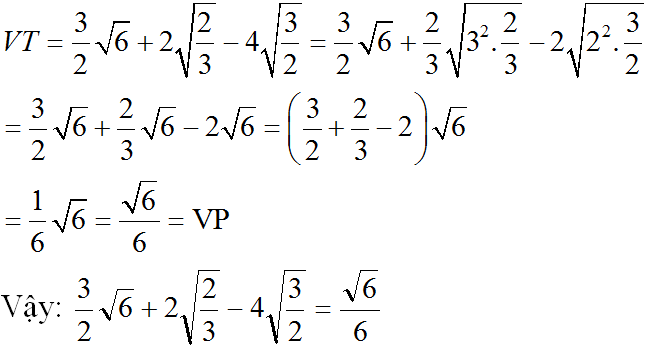
1.có \(x^2-2x+2=x^2-2x+1+1=\left(x-1\right)^2+1\ge1\)
\(=>\dfrac{x^2-2x+2}{2012}\ge\dfrac{1}{2012}>0\)
Vậy biểu thức trên xác định với mọi x
2. đề này sai thử x=0,8 vào căn kia sẽ ra âm nên ko thể xác định với mọi x
1) Ta có: \(x^2-2x+2=\left(x-1\right)^2+1>0\forall x\)
\(\Leftrightarrow\dfrac{x^2-2x+2}{2012}>0\forall x\)
Do đó: \(\sqrt{\dfrac{x^2-2x+2}{2012}}\) xác định được với mọi x