
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


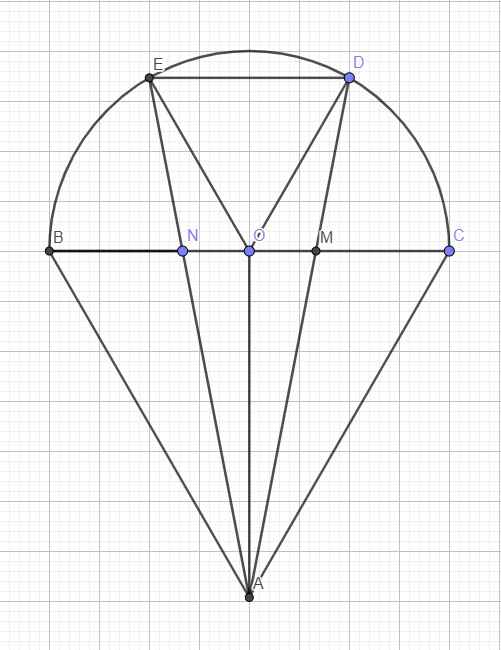
Gọi O là tâm đường tròn \(\Rightarrow\) O là trung điểm BC
\(\stackrel\frown{BE}=\stackrel\frown{ED}=\stackrel\frown{DC}\Rightarrow\widehat{BOE}=\widehat{EOD}=\widehat{DOC}=\dfrac{180^0}{3}=60^0\)
Mà \(OD=OE=R\Rightarrow\Delta ODE\) đều
\(\Rightarrow ED=R\)
\(BN=NM=MC=\dfrac{2R}{3}\Rightarrow\dfrac{NM}{ED}=\dfrac{2}{3}\)
\(\stackrel\frown{BE}=\stackrel\frown{DC}\Rightarrow ED||BC\)
Áp dụng định lý talet:
\(\dfrac{AN}{AE}=\dfrac{MN}{ED}=\dfrac{2}{3}\Rightarrow\dfrac{EN}{AN}=\dfrac{1}{2}\)
\(\dfrac{ON}{BN}=\dfrac{OB-BN}{BN}=\dfrac{R-\dfrac{2R}{3}}{\dfrac{2R}{3}}=\dfrac{1}{2}\)
\(\Rightarrow\dfrac{EN}{AN}=\dfrac{ON}{BN}=\dfrac{1}{2}\) và \(\widehat{ENO}=\widehat{ANB}\) (đối đỉnh)
\(\Rightarrow\Delta ENO\sim ANB\left(c.g.c\right)\)
\(\Rightarrow\widehat{NBA}=\widehat{NOE}=60^0\)
Hoàn toàn tương tự, ta có \(\Delta MDO\sim\Delta MAC\Rightarrow\widehat{MCA}=\widehat{MOD}=60^0\)
\(\Rightarrow\Delta ABC\) đều




Lời giải:
Ta có \(P=\frac{1}{a^2+b^2}+\frac{1}{2ab}+\frac{1}{4ab}+\frac{1}{4ab}+4ab\)
Áp dụng BĐT Cauchy-Schwarz:
\(\frac{1}{a^2+b^2}+\frac{1}{2ab}\geq \frac{4}{a^2+b^2+2ab}=\frac{4}{(a+b)^2}\geq 4\)
Áp dụng BĐT AM-GM: \(\frac{1}{4ab}+4ab\geq 2\).
Và \(1\geq a+b\geq 2\sqrt{ab}\rightarrow ab\leq \frac{1}{4}\)
Do đó \(P\geq 4+1+2=7\) hay \(P_{\min}=7\)
Dấu bằng xảy ra khi \(a=b=\frac{1}{2}\)

1. a) Ta có :A=99...9000...0+25(n chữ số 9,n +2 chữ số 0)
Đặt a=11...1(n chữ số 1 ) suy ra : 10n=9a+1.Khi đó :
A=9a.(9a+1).100+25=8100a2+900a+25=(90a+5)2=99...952
2.a)
Ta có :A=11...1\(\times\)10n+11...1-22...2(n chữ số 1 ,n chữ số 2)
Đặt a=11...1 (n chữ số 1) suy ra 10n=9a+1,22...2=2a.Khi đó :
A=(a(9a+1)+a)-2a=9a2=(3a)2=33...32(n chữ số 3)
b)Tương tự :B=a(9a+1)+a+4a+1=9a2+6a+1=(3a+1)2=33..342(n -1 chữ số 3)

Áp dụng BĐT \(\dfrac{1}{x}+\dfrac{1}{y}\ge\dfrac{4}{x+y}\)(Tự chứng minh BĐT này )
\(B\ge\dfrac{4}{\left(a+b\right)^2+1}\)
![]() cảm ơn Định đã trả lời giúp mk . Nhưng bn làm sai rồi vì nếu làm như vậy sẽ ko tìm ra a, b
cảm ơn Định đã trả lời giúp mk . Nhưng bn làm sai rồi vì nếu làm như vậy sẽ ko tìm ra a, b











Bài 1:
a: \(A=2\sqrt{3}-\sqrt{27}+\sqrt{4-2\sqrt{3}}\)
\(=2\sqrt{3}-3\sqrt{3}+\sqrt{3}-1\)
=-1
đọc kĩ đề , chỉ cần làm câu 4 ý b thôi