Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Dễ thấy:
Vecto \(\overrightarrow {OM} \) và \(\overrightarrow {OA} \)có cùng giá nên chúng cùng phương.
Mà vecto \(\overrightarrow {OM} \) và \(\overrightarrow {OA} \)cùng nằm trên tia OM nên chúng cùng chiều
Vậy vecto \(\overrightarrow {OM} \) và \(\overrightarrow {OA} \)cùng hướng.
Ngoài ra, \(\left| {\overrightarrow {OM} } \right| = OM = \sqrt 2 \) và \(\left| {\overrightarrow {OA} } \right| = OA = 1\)
\( \Rightarrow \left| {\overrightarrow {OM} } \right| = \sqrt 2 .\left| {\overrightarrow {OA} } \right|\)
Ta kết luận \(\overrightarrow {OM} = \sqrt 2 .\overrightarrow {OA} \).

a) Gọi M, N lần lượt là điểm đầu và điểm cuối của vecto \(\overrightarrow a \).
Vì \(\overrightarrow a = \overrightarrow {AB} \Leftrightarrow \overrightarrow {MN} = \overrightarrow {AB} \) nên tứ giác MNBA là hình bình hành.
Nói cách khác B là đỉnh thứ tư của hình bình hành tạo bởi vecto \(\overrightarrow a \) và điểm A.
Tương tự, C là đỉnh thứ tư của hình bình hành tạo bởi vecto \(\overrightarrow b \) và điểm B.
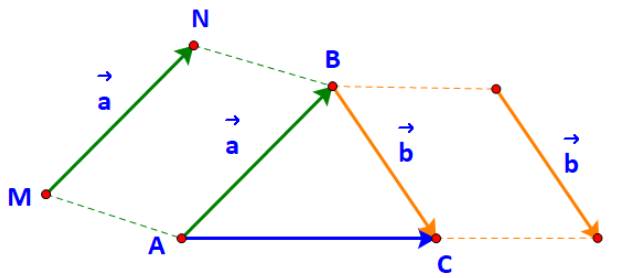
b) Dễ thấy: tổng của hai vecto \(\overrightarrow {AB} \) và \(\overrightarrow {BC} \) là vecto \(\overrightarrow {AC} \).
Do đó tổng của hai vecto \(\overrightarrow a \) và \(\overrightarrow b \)bằng vecto \(\overrightarrow {AC} \).
Ta có viết: \(\overrightarrow a + \overrightarrow b = \overrightarrow {AB} + \overrightarrow {BC} = \overrightarrow {AC} \)

Dễ thấy:
\(AD = BC\) nhưng \(AD\) và \(BC\) không song song với nhau. Do đó hai vectơ \(\overrightarrow {AD} \) và \(\overrightarrow {BC} \) không bằng nhau.
\(CD > AB\) do đó hai vectơ \(\overrightarrow {AB} \) và \(\overrightarrow {CD} \) không bằng nhau.
\(AC\) và \(BD\) không song song với nhau. Do đó hai vectơ \(\overrightarrow {AC} \) và \(\overrightarrow {BD} \) không bằng nhau.

\(\Leftrightarrow\left\{{}\begin{matrix}5=2x+1.y\\12=3.x-4.y\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}x=\dfrac{32}{11}\\y=-\dfrac{9}{11}\end{matrix}\right.\)

Tham khảo:
Kí hiệu O, E, F là các điểm như trên hình vẽ.
Dễ thấy: tứ giác OEMF là hình bình hành nên \(\overrightarrow {OE} + \overrightarrow {OF} = \overrightarrow {OM} \) hay \(\overrightarrow v + \overrightarrow u = \overrightarrow {OM} \)
Và \(\overrightarrow {OC} = 3.\overrightarrow {OM} \Rightarrow 3\left( {\overrightarrow v + \overrightarrow u } \right) = 3.\overrightarrow {OM} = \overrightarrow {OC} \)
Mặt khác: \(\overrightarrow {OA} = 3.\overrightarrow {OF} = 3\;\overrightarrow u ;\;\overrightarrow {OB} = 3.\overrightarrow {OE} = 3\;\overrightarrow v \)
Và \(\overrightarrow {OB} + \overrightarrow {OA} = \overrightarrow {OC} \) hay \(3\;\overrightarrow v + 3\;\overrightarrow u = \overrightarrow {OC} \)
\( \Rightarrow 3\left( {\overrightarrow v + \overrightarrow u } \right) = 3\;\overrightarrow v + 3\;\overrightarrow u \)

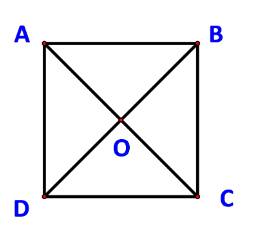
a) Do ABCD cũng là một hình bình hành nên \(\overrightarrow {DA} + \overrightarrow {DC} = \overrightarrow {DB} \)
\( \Rightarrow \;|\overrightarrow {DA} + \overrightarrow {DC} |\; = \;|\overrightarrow {DB} |\; = DB = a\sqrt 2 \)
b) Ta có: \(\overrightarrow {AD} + \overrightarrow {DB} = \overrightarrow {AB} \) \( \Rightarrow \overrightarrow {AB} - \overrightarrow {AD} = \overrightarrow {DB} \)
\( \Rightarrow \left| {\overrightarrow {AB} - \overrightarrow {AD} } \right| = \left| {\overrightarrow {DB} } \right| = DB = a\sqrt 2 \)
c) Ta có: \(\overrightarrow {DO} = \overrightarrow {OB} \)
\( \Rightarrow \overrightarrow {OA} + \overrightarrow {OB} = \overrightarrow {OA} + \overrightarrow {DO} = \overrightarrow {DO} + \overrightarrow {OA} = \overrightarrow {DA} \)
\( \Rightarrow \left| {\overrightarrow {OA} + \overrightarrow {OB} } \right| = \left| {\overrightarrow {DA} } \right| = DA = a.\)

Chị ơi giúp e cái này tìm 3 giá trị của x sao cho 0,6<x<0,61
Gọi I là tâm đường tròn nội tiếp tam giác ABC
\(\Rightarrow a\overrightarrow{IA}+b\overrightarrow{IB}+c\overrightarrow{IC}=0\)
Ta có:
\(A=\left|a\overrightarrow{MA}+b\overrightarrow{MB}+c\overrightarrow{MC}\right|=\left|\left(a+b+c\right)\overrightarrow{MI}+a\overrightarrow{IA}+b\overrightarrow{IB}+c\overrightarrow{IC}\right|\)
\(=\left|\left(a+b+c\right)\overrightarrow{MI}\right|=\left(a+b+c\right).MI\)
\(Amin\Leftrightarrow MImin\)
\(\Leftrightarrow\) M trùng I

Tham khảo:
Gọi M, N lần lượt là điểm đầu và điểm cuối của vecto \(\overrightarrow a \).
Từ B, M, N ta dựng hình bình hành BMNC.
Khi đó: \(\overrightarrow {MN} = \overrightarrow {BC} \) hay \(\overrightarrow a = \overrightarrow {BC} \).
\( \Rightarrow \overrightarrow a + \overrightarrow a = \overrightarrow {AB} + \overrightarrow {BC} = \overrightarrow {AC} \)
a) Vì \(\overrightarrow {AB} = \overrightarrow a = \overrightarrow {BC} \) nên A, B, C thẳng hàng và B là trung điểm của AC.
Vậy \(\overrightarrow a + \overrightarrow a \) và \(\overrightarrow {AB} \) cùng hướng, \(\left| {\overrightarrow a + \overrightarrow a } \right| = 2.\left| {\overrightarrow {AB} } \right|\)
b) Ta có: \(\overrightarrow a + \overrightarrow a \) và \(\overrightarrow {AB} \) cùng hướng, \(\left| {\overrightarrow a + \overrightarrow a } \right| = 2.\left| {\overrightarrow {AB} } \right|\)
Mà \(\overrightarrow {AB} = \overrightarrow a \) nên: \(\overrightarrow a + \overrightarrow a \) và \(\overrightarrow a \) cùng hướng, \(\left| {\overrightarrow a + \overrightarrow a } \right| = 2.\left| {\overrightarrow a } \right|\).