Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a.
\(\overrightarrow{IA}+2\left(\overrightarrow{IA}+\overrightarrow{AB}\right)=\overrightarrow{0}\Leftrightarrow3\overrightarrow{IA}+2\overrightarrow{AB}=0\)
\(\Leftrightarrow\overrightarrow{AI}=\frac{2}{3}\overrightarrow{AB}\)
Vậy I là điểm nằm trên đoạn thẳng AB sao cho \(AI=\frac{2}{3}AB\)
b.
Gọi G là trọng tâm tam giác ABC
\(\overrightarrow{KG}+\overrightarrow{GA}+2\left(\overrightarrow{KG}+\overrightarrow{GB}\right)=\overrightarrow{CG}+\overrightarrow{GB}\)
\(\Leftrightarrow3\overrightarrow{KG}+\overrightarrow{GA}+\overrightarrow{GB}+\overrightarrow{GC}=0\Leftrightarrow3\overrightarrow{KG}=\overrightarrow{0}\)
\(\Leftrightarrow\) K trùng G hay K là trọng tâm tam giác
c.
\(\overrightarrow{MG}+\overrightarrow{GA}+\overrightarrow{MG}+\overrightarrow{GB}+2\left(\overrightarrow{MG}+\overrightarrow{GC}\right)=0\)
\(\Leftrightarrow4\overrightarrow{MG}+\overrightarrow{GC}=0\Leftrightarrow\overrightarrow{GM}=\frac{1}{4}\overrightarrow{GC}\)
Vậy M là điểm nằm trên đoạn thẳng CG sao cho \(GM=\frac{1}{4}CG\)

a) gọi I là trung điểm của đoạn thẳng AB
=> IA+ IB=0
| 2MI|= |BA|
|MI|= 1/2|BA|
=> M thuộc đường tròn tâm I, bán kính =1/2 BA
B) gọi G là trọng tâm của tam giác ABC
=> GA+ GB+ GC=0
gọi I là trung điểm của đoạn thẳng AB
=> IA+ IB=0
| 3MG|= 3/2| 2 MI|
3| MG|= 3| MI|
| MG|= | MI|
=> M thuộc đường trung trực của đoạn thẳng GI

a) Từ điểm I trên AB thỏa mãn IA = 1/2 IB ta vẽ đường song song với BC. Điểm N nằm trên đó.
B) tương tự câu a)
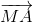 +
+ = 2
= 2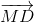
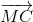 =
= 
a)Giả sử điểm K thỏa mãn:
\(\overrightarrow{KA}+2\overrightarrow{KB}=\overrightarrow{CB}\)\(\Leftrightarrow\overrightarrow{KB}+\overrightarrow{BA}+2\overrightarrow{KB}=\overrightarrow{CB}\)
\(\Leftrightarrow3\overrightarrow{KB}=\overrightarrow{CB}-\overrightarrow{BA}\)
\(\Leftrightarrow\overrightarrow{KB}=\overrightarrow{CB}+\overrightarrow{AB}\).
Xác định: \(\overrightarrow{CB}+\overrightarrow{AB}\).
A B C D
Lấy điểm D sao cho B là trung điểm của DC.
\(\overrightarrow{CB}+\overrightarrow{AB}=\overrightarrow{BD}+\overrightarrow{AB}=\overrightarrow{AD}\).
Điểm K xác định sao cho : \(\overrightarrow{KB}=\overrightarrow{AD}\) hay tứ giác AKBD là hình bình hành.
A B C D K
b) Gọi G là trọng tâm tam giác ABC.
Ta có \(\overrightarrow{MA}+\overrightarrow{MB}+2\overrightarrow{MC}=\overrightarrow{MG}+\overrightarrow{GA}+\overrightarrow{MG}+\overrightarrow{GB}+2\overrightarrow{MG}\)\(+2\overrightarrow{GC}\)
\(=4\overrightarrow{MG}+\left(\overrightarrow{GA}+\overrightarrow{GB}+\overrightarrow{GC}\right)+\overrightarrow{GC}\)
\(=4\overrightarrow{MG}+\overrightarrow{GC}\).
Giả sử điểm M thỏa mãn:
\(\overrightarrow{MA}+\overrightarrow{MB}+2\overrightarrow{MC}=\overrightarrow{0}\Leftrightarrow4\overrightarrow{MG}+\overrightarrow{GC}=\overrightarrow{0}\)
\(\Leftrightarrow\overrightarrow{MG}=\dfrac{\overrightarrow{CG}}{4}\).
Điểm M được xác định để \(\overrightarrow{MG}=\dfrac{\overrightarrow{CG}}{4}\).
A B C G T M
Gọi T là trung điểm của AB nên \(\overrightarrow{CG}=2\overrightarrow{GT}\).
Vì vậy điểm M được xác định là trung điểm của GT.