Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Để pt có 2 nghiệm trái dấu \(\Leftrightarrow ac< 0\Leftrightarrow-\left(m^2-4\right)< 0\Rightarrow\left[{}\begin{matrix}m>2\\m< -2\end{matrix}\right.\)
Do \(x_1< x_2\Rightarrow x_1< 0< x_2\Rightarrow\left\{{}\begin{matrix}\left|x_1\right|=-x_1\\\left|x_2\right|=x_2\end{matrix}\right.\)
\(\left|x_1\right|>\left|x_2\right|\Leftrightarrow-x_1>x_2\Leftrightarrow x_1+x_2< 0\Leftrightarrow\frac{-\left(m+3\right)}{-1}< 0\Rightarrow m< -3\)

để pt có 2 nghiệm phân biệt thì: đenta > 0
mà ddeenta = m2 - 6m - 7 > 0
giải ra ta đc: m<-1 hay m>7 (1)
áp dụng hệ thức vi-et đc x1 + x2 = m-1 và x1.x2= m+2
kết 2 biểu thức trên dễ dàng làm đc x12 + x22 = m2-4m-3
bđt trên (=) (x12+x22)/x12.x22 - 1 > 0
thay vào đc (-16m -7)/(m2+4m+4) > 0 =) m khác -2 và m<-7/16
kết hợp vs (1) =) m<-1 và m khác -2

1: \(\text{Δ}=\left(-m\right)^2-4\left(m-2\right)=m^2-4m+8=\left(m-2\right)^2+4>0\)
=>Phương trình luôn có hai nghiệm phân biệt
Theo đề, ta có: m-2<0
=>m<2
2: \(\Leftrightarrow\dfrac{x_1^2+1}{x_1}\cdot\dfrac{x_2^2+1}{x_2}=9\)
\(\Leftrightarrow\dfrac{\left(x_1\cdot x_2\right)^2+\left(x_1+x_2\right)^2-2x_1x_2+1}{x_1x_2}=9\)
\(\Leftrightarrow\dfrac{\left(m-2\right)^2+\left(-m\right)^2-2\left(m-2\right)+1}{m-2}=9\)
\(\Leftrightarrow m^2-4m+4+m^2-2m+4+1=9m-18\)
\(\Leftrightarrow2m^2-6m+9-9m+18=0\)
=>2m^2-15m+27=0
hay \(m\in\varnothing\)
3: =>m=0

Đặt \(f\left(x\right)=x^2-2\left(m-2\right)x-9m+10\)
Để pt có 2 nghiệm thỏa mãn \(x_1< 2< x_2\)
\(\Leftrightarrow1.f\left(2\right)< 0\)
\(\Leftrightarrow4-4\left(m-2\right)-9m+10< 0\)
\(\Leftrightarrow13m>22\Rightarrow m>\frac{22}{13}\)
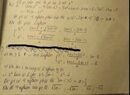

\(\text{Δ}=\left(2m+6\right)^2-4\left(m^2-3\right)\)
\(=4m^2+24m+36-4m^2+12=24m+48\)
Để phương trình có hai nghiệm thì 24m+48>=0
=>m>=-2
\(P=5\left(-2m-6\right)-2\left(m^2-3\right)\)
\(=-10m-30-2m^2+6\)
\(=-2m^2-10m-24\)
\(=-2\left(m^2+5m+12\right)\)
\(=-2\left(m^2+5m+\dfrac{25}{4}+\dfrac{23}{4}\right)\)
\(=-2\left(m+\dfrac{5}{2}\right)^2-\dfrac{23}{2}< =-\dfrac{23}{2}\)
Dấu = xảy ra khi m=-5/2