
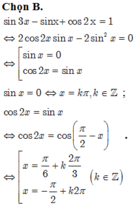
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

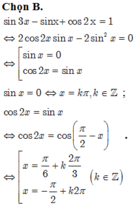

a.\(\frac{k\Pi}{2}+\frac{\alpha}{2}\)
b.\(\left\{{}\begin{matrix}x=\frac{1}{4}arcsin\left(\frac{1}{3}\right)+\frac{k\Pi}{2}-\frac{1}{8}\\x=\Pi-\frac{1}{4}arcsin\left(\frac{1}{3}\right)+\frac{k\Pi}{2}-\frac{1}{8}\end{matrix}\right.\)

Câu g đề thiếu
Câu 2:
\(sin\left(2x+\frac{\pi}{6}\right)=\frac{2}{5}\)
\(\Leftrightarrow\left[{}\begin{matrix}2x+\frac{\pi}{6}=arcsin\left(\frac{2}{5}\right)+k2\pi\\2x+\frac{\pi}{6}=\pi-arcsin\left(\frac{2}{5}\right)+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-\frac{\pi}{12}+\frac{1}{2}arcsin\left(\frac{2}{5}\right)+k\pi\\x=\frac{5\pi}{12}-\frac{1}{2}arcsin\left(\frac{2}{5}\right)+k\pi\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x\approx-0,056\left(rad\right)\\x\approx1,1\left(rad\right)\end{matrix}\right.\)

1/ ĐKXĐ: \(\cos2x\ne0\)
\(\frac{\cos4x}{\cos2x}=\frac{\sin2x}{\cos2x}\)\(\Leftrightarrow\cos4x-\sin2x=0\)
\(\Leftrightarrow2\cos^22x-1-\sin2x=0\)
\(\Leftrightarrow2-2\sin^22x-1-\sin2x=0\)
\(\Leftrightarrow2\sin^22x+\sin2x-1=0\)
\(\Leftrightarrow\left[{}\begin{matrix}\sin2x=\frac{1}{2}=\sin\frac{\pi}{6}\\\sin2x=-1=\sin\frac{-\pi}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}2x=\frac{\pi}{6}+2k\pi\\2x=\frac{5\pi}{6}+2k\pi\\2x=\frac{-\pi}{2}+2k\pi\left(l\right)\\2x=\frac{3\pi}{2}+2k\pi\left(l\right)\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\frac{\pi}{12}+k\pi\\x=\frac{5\pi}{12}+k\pi\end{matrix}\right.\)
2/ \(\sin2.4x+\cos4x=1+2\sin2x.\cos\left(2x+4x\right)\)
\(\Leftrightarrow2\sin4x.\cos4x+\cos4x=1+2\sin2x.\left(\cos2x.\cos4x-\sin2x.\sin4x\right)\)
\(\Leftrightarrow2\sin4x.\cos4x+\cos4x=1+2\sin2x.\cos2x.\cos4x-2\sin^22x.\sin4x\)
\(\Leftrightarrow2\sin4x.\cos4x+\cos4x=1+\sin4x.\cos4x-\sin4x+\cos4x.\sin4x\)
Đến đây bn tự giải nốt nhé, lm kiểu bthg thôi bởi vì đã quy về hết sin4x và cos4x r

a) cosx - √3sinx = √2 ⇔ cosx - tan![]() sinx = √2
sinx = √2
⇔ cos![]() cosx - sin
cosx - sin![]() sinx = √2cos
sinx = √2cos![]() ⇔ cos(x +
⇔ cos(x + ![]() ) =
) = ![]()
⇔ 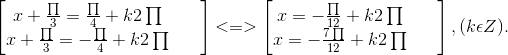
b) 3sin3x - 4cos3x = 5 ⇔ ![]() sin3x -
sin3x - ![]() cos3x = 1.
cos3x = 1.
Đặt α = arccos![]() thì phương trình trở thành
thì phương trình trở thành
cosαsin3x - sinαcos3x = 1 ⇔ sin(3x - α) = 1 ⇔ 3x - α = ![]() + k2π
+ k2π
⇔ x = ![]() , k ∈ Z (trong đó α = arccos
, k ∈ Z (trong đó α = arccos![]() ).
).