Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(tan\left(\dfrac{3\pi}{2}-\alpha\right)+cot\left(3\pi-\alpha\right)-cos\left(\dfrac{\pi}{2}-\alpha\right)+2.sin\left(\pi+\alpha\right)\)
\(=tan\left(\pi+\dfrac{\pi}{2}-\alpha\right)+cot\left(-\alpha\right)-sin\alpha+2\left(sin\pi.cos\alpha+cos\pi.sin\alpha\right)\)
\(=tan\left(\dfrac{\pi}{2}-\alpha\right)-cot\alpha-sin\alpha+2.-sin\alpha\)
\(=cot\alpha-cot\alpha-3sin\alpha\)
\(=-3sin\alpha\)

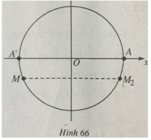
(h.66) Ta có
A M 2 = MA’ = MA + AA’
Suy ra
Sđ A M 2 = -α + π + k2π, k ∈ Z.
Vậy đáp án là B.
6.13. (h.67) Ta có
Sđ A M 3 = -sđ AM = -α + k2π, k ∈ Z.
Đáp án: D
a/ \(cosa=-\sqrt{1-sin^2a}=-\frac{\sqrt{5}}{3}\)
\(tana=\frac{sina}{cosa}=-\frac{2\sqrt{5}}{5}\) ; \(cota=\frac{1}{tana}=-\frac{\sqrt{5}}{2}\)
b/ \(\frac{1}{cos^2a}=1+tan^2a\Rightarrow cos^2a=\frac{1}{1+tan^2a}\)
\(\Rightarrow cosa=-\frac{1}{\sqrt{1+tan^2a}}=-\frac{\sqrt{3}}{3}\); \(sina=-\sqrt{1-cos^2a}=-\frac{\sqrt{6}}{3}\)
\(cota=\frac{1}{tana}=\frac{\sqrt{2}}{2}\)
c/ \(sina=\sqrt{1-cos^2a}=\frac{\sqrt{5}}{5}\); \(tana=\frac{sina}{cosa}=\frac{1}{2}\); \(cota=\frac{1}{tana}=2\)
d/ \(sina=\sqrt{1-cos^2a}=\frac{\sqrt{209}}{15}\); \(tana=\frac{sina}{cosa}=\frac{\sqrt{209}}{4}\); \(cota=\frac{1}{tana}=\frac{4}{\sqrt{209}}\)
e/ \(\frac{1}{sin^2a}=1+cot^2a\Rightarrow sin^2a=\frac{1}{1+cot^2a}\Rightarrow sina=\frac{-1}{\sqrt{1+cot^2a}}\)
\(\Rightarrow sina=-\frac{\sqrt{10}}{10}\); \(cosa=\sqrt{1-sin^2a}=\frac{3\sqrt{10}}{10}\); \(cota=\frac{1}{tana}=-\frac{1}{3}\)
f/ \(cosa=-\frac{1}{\sqrt{1+tan^2a}}=-\frac{\sqrt{5}}{5}\); \(sina=tana.cosa=\frac{2\sqrt{5}}{5}\); \(cota=\frac{1}{tana}=-\frac{1}{2}\)
g/ Đề sai, trong khoảng \(\pi< a< \frac{3\pi}{2}\) thì \(\left\{{}\begin{matrix}sina< 0\\cosa< 0\end{matrix}\right.\) nên \(tana>0\)
\(\Rightarrow tana\) không thể nhận giá trị âm, ko có góc \(\alpha\)