
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Bài 1:
a)\(\frac{2}{3}.\frac{5}{2}-\frac{3}{4}.\frac{2}{3}=\frac{5}{3}-\frac{1}{2}=\frac{7}{6}\)
b)\(2.\left(\frac{-3}{2}\right)^2-\frac{7}{2}=\frac{2.9}{4}-\frac{7}{2}=\frac{9-7}{2}=\frac{2}{2}=1\)
c)\(-\frac{3}{4}.\frac{68}{13}-0,75.\frac{36}{13}=\frac{-3.4.17}{4.13}-\frac{3.9.4}{4.13}=\frac{-51-27}{13}=\frac{-78}{13}=-6\)
Bài 2:
a)|x-1,4|=1,6
\(\Rightarrow\left[\begin{array}{nghiempt}x-1,4=1,6\\x-1,4=-1,6\end{array}\right.\)
\(\Rightarrow\left[\begin{array}{nghiempt}x=3\\x=-0,2\end{array}\right.\)
b) \(\frac{3}{4}-x=\frac{4}{5}\)
\(x=\frac{3}{4}-\frac{4}{5}=-\frac{1}{20}\)
c)(1-2x)3=-8
(1-2x)3=(-2)3
1-2x=-2
2x=3
x=\(\frac{3}{2}\)
Bài 3:
\(\frac{x}{2}=\frac{y}{5}=\frac{z}{7}=k\)
\(\Rightarrow\begin{cases}x=2k\\y=5k\\z=7k\end{cases}\)
A=\(\frac{2k-5k+7k}{2k+2.5k-7k}=\frac{4k}{5k}=\frac{4}{5}\)
=> x=4/5 . 2= 8/5
y=4/5 . 5=4
z=4/5.7=28/5

\(A=\dfrac{1}{2}+\dfrac{1}{2^2}+\dfrac{1}{2^3}+...+\dfrac{1}{2^{100}}\)
\(2A=1+\dfrac{1}{2}+\dfrac{1}{2^2}+...+\dfrac{1}{2^{99}}\)
\(2A-A=\left(1+\dfrac{1}{2}+\dfrac{1}{2^2}+...+\dfrac{1}{2^{99}}\right)-\left(\dfrac{1}{2}+\dfrac{1}{2^2}+\dfrac{1}{2^3}+...+\dfrac{1}{2^{100}}\right)\)
\(A=1-\dfrac{1}{2^{100}}< 1\)
Vậy A < B.
Giải:
Có: \(A=\dfrac{1}{2}+\dfrac{1}{2^2}+\dfrac{1}{2^3}+...+\dfrac{1}{2^{100}}\)
\(\Leftrightarrow\dfrac{1}{2}A=\dfrac{1}{2^2}+\dfrac{1}{2^3}+\dfrac{1}{2^4}+...+\dfrac{1}{2^{101}}\)
Lấy vế trừ theo vế, ta được:
\(A-\dfrac{1}{2}A=\dfrac{1}{2}A=\dfrac{1}{2}-\dfrac{1}{2^{101}}\)
\(\Leftrightarrow\dfrac{1}{2}A=\dfrac{1}{2}-\dfrac{1}{2^{101}}\)
\(\Leftrightarrow A=\dfrac{\dfrac{1}{2}-\dfrac{1}{2^{101}}}{\dfrac{1}{2}}\)
\(\Leftrightarrow A=\dfrac{\dfrac{1}{2}\left(1-\dfrac{1}{2^{100}}\right)}{\dfrac{1}{2}}\)
\(\Leftrightarrow A=1-\dfrac{1}{2^{100}}\)
Lại có \(B=1\)
Vì \(1-\dfrac{1}{2^{100}}< 1\)
Nên \(A< B\)
Vậy \(A< B\).
Chúc bạn học tốt!

a) ΔABC có:
\(\widehat{A}\) + \(\widehat{B}\) + \(\widehat{C}\) = 180o hay 100o + \(\widehat{B}\) + \(\widehat{C}\) = 180o
\(\Rightarrow\) \(\widehat{B}\) + \(\widehat{C}\) = 180o - 100o = 80o
Ta có: \(\widehat{B}\) + \(\widehat{C}\) = 80o(cm trên) ; \(\widehat{B}\) - \(\widehat{C}\) = 50o (gt)
\(\Rightarrow\) \(\widehat{B}\) = (80o + 50o ) : 2 = 65o
\(\widehat{C}\) = (80o - 50o) : 2 = 15o
b) ΔABC có:
\(\widehat{B}\) + \(\widehat{A}\) + \(\widehat{C}\) = 180o hay 80o + \(\widehat{A}\) + \(\widehat{C}\) = 180o
\(\Rightarrow\) \(\widehat{A}\) + \(\widehat{C}\) = 180o - 80o = 100o
Ta có: 3 . \(\widehat{A}\) = 2 . \(\widehat{C}\) => \(\frac{\widehat{A}}{2}\) = \(\frac{\widehat{C}}{3}\)
Áp dụng tính chất của dãy tỉ số bằng nhau ta có:
\(\frac{\widehat{A}}{2}\) = \(\frac{\widehat{C}}{3}\) = \(\frac{\widehat{A}+\widehat{C}}{2+3}\) = \(\frac{100}{5}\) = 20
\(\Rightarrow\) \(\begin{cases}\widehat{A}=40^o\\\widehat{C}=60^o\end{cases}\)

a, Ta có :
\(A=\left(\dfrac{1}{2}-1\right)\left(\dfrac{1}{3}-1\right)...........\left(\dfrac{1}{10}-1\right)\)
\(=\left(\dfrac{1}{2}-\dfrac{2}{2}\right)\left(\dfrac{1}{3}-\dfrac{3}{3}\right).........\left(\dfrac{1}{10}-\dfrac{10}{10}\right)\)
\(=\dfrac{-1}{2}.\dfrac{-2}{3}...............\dfrac{-9}{10}\)
\(=\dfrac{-1.\left(-2\right)............\left(-9\right)}{2.3........9.10}\)
\(=\dfrac{-1}{10}< \dfrac{-1}{9}\)
\(\Leftrightarrow A< \dfrac{-1}{9}\)
b, \(B=\left(\dfrac{1}{4}-1\right)\left(\dfrac{1}{9}-1\right)..........\left(\dfrac{1}{100}-1\right)\)
\(=\left(\dfrac{1}{4}-\dfrac{4}{4}\right)\left(\dfrac{1}{9}-\dfrac{9}{9}\right).........\left(\dfrac{1}{100}-\dfrac{100}{100}\right)\)
\(=\dfrac{-3}{4}.\dfrac{-8}{9}..............\dfrac{-99}{100}\)
\(=\dfrac{1.\left(-3\right).2\left(-4\right)............9\left(-11\right)}{2^2.3^2.......10^2}\)
\(=\dfrac{1.2.3........9}{2.3.......10}.\dfrac{\left(-3\right)\left(-4\right)....\left(-11\right)}{2.3...10}\)
\(=\dfrac{1}{10}.\dfrac{-11}{1}\)
\(=\dfrac{-11}{10}>\dfrac{-11}{21}\)
\(\Leftrightarrow B>\dfrac{-11}{21}\)

Có \(\dfrac{a}{b}=2\Rightarrow a=2b\)
Mà a-b=2
\(\Rightarrow2b-b=2\Rightarrow b=2\Rightarrow a=2+2=4\)
\(\dfrac{a}{b}=2\)
\(\Rightarrow\) \(\dfrac{a}{2}=\dfrac{b}{1}\)
Áp dụng tính chất dãy tỉ số bằng nhau, ta có:
\(\dfrac{a}{2}=\dfrac{b}{1}=\dfrac{a-b}{2-1}=\dfrac{2}{1}=2\)
\(\Rightarrow\left\{{}\begin{matrix}a=2.2\\b=2.1\end{matrix}\right.\Rightarrow\left\{{}\begin{matrix}a=4\\b=2\end{matrix}\right.\)
Vậy, a = 4; b = 2

a) Ta có: IS \(\perp\) IH tại I
IS \(\perp\) SK tại S
Do đó IH // SK (quan hệ từ vuông góc đến song song)
Vậy IH // SK
b) Có nhiều cách:
C1: Vì IH // SK
=> Góc H1 + góc K1 = 180o
=> Góc K1 = 180o - góc H1

ĐỀ 2
I. PHẦN TRẮC NGHIỆM: (3 điểm)
Khoanh tròn chữ cái đứng trước câu trả lời đúng:
1. Trong các khẳng định sau, khẳng định sai là:
A. I ⊂ R
B. I ∪ Q = R
C. Q ⊂ I
D. Q ⊂ R
2. Kết quả của phép nhân (-0,5)3.(-0,5) bằng:
A. (-0,5)3
B. (-0,5)
C. (-0,5)2
D. (0,5)4
3. Giá trị của (-2/3) ³ bằng:

=> Chọn B
4. Nếu | x | = |-9 |thì:
A. x = 9 hoặc x = -9
B. x = 9
B. x = -9
D. Không có giá trị nào của x để thỏa mãn
5. Kết quả của phép tính 36.34. 32 bằng:
A. 2712
B. 312
C. 348
D. 2748
=> 39168
6. Kết quả của phép tính ![]()

I. TRẮC NGHIỆM KHÁCH QUAN (3đ)
Khoanh tròn vào đáp án đúng trong các câu sau:
Câu 1: Trong các câu sau, câu nào sai
A. Nếu a là số thực thì a là số hữu tỉ hoặc số vô tỉ
B. Nếu b là số vô tỉ thì b viết được dưới dạng số thập phân vô hạn không tuần hoàn
C. Nếu c là số vô tỉ thì c cũng là số thực
D. Nếu c là số thực thì c cũng là số vô tỉ
Câu 2: Kết qủa của phép tính 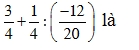
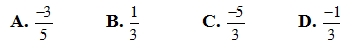
=> Chọn B
Câu 3: Kết qủa của phép tính 36 . 32 =
A. 98
B. 912
C. 38
D. 312
=> 1152
Câu 4: Từ đẳng thức a.d = b.c có thể suy ra tỉ lệ thức nào sau đây:
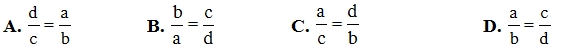
=> Chọn D
Câu 5: Viết số thập phân hữu hạn dưới dạng phân số tối giản :
![]()
=> Chọn A
Câu 6: Nếu √x = 3 thì x =
A. 3
B. 9
C. -9
D. ±9
II. TRẮC NGHIỆM TỰ LUẬN (7đ)
Bài 1 (1,5đ) Tính:
\(=\left(\frac{8}{9}+\frac{1}{9}\right)+\left(\frac{15}{23}-\frac{15}{13}\right)+\frac{1}{2}\) \(=\left(-\frac{5}{7}\right)\times\left(12,5+1,5\right)\) \(=15\times\frac{4}{9}-\frac{7}{3}\)
\(=\frac{9}{9}+0+0,5\) \(=\left(-\frac{5}{7}\right)\times14\) \(=\frac{20}{3}-\frac{7}{3}\)
\(=1+0,5\) \(=-10\) \(=\frac{13}{3}\)
\(=1,5\)
Bài 2 (2đ): Ba lớp 8A, 8B, 8C trồng được 180 cây . Tính số cây trồng được của mỗi lớp, biết rằng số cây trồng được của mỗi lớp lần lượt tỉ lệ với 4 : 6 : 8
Gọi số cây trồng được của lớp 8A, 8B, 8C theo thứ tự là a, b và c.
Áp dụng tính chất của dãy tỉ số bằng nhau, ta có:
\(\frac{a}{4}=\frac{b}{6}=\frac{c}{8}=\frac{a+b+c}{4+6+8}=\frac{180}{18}=10\)
\(\left[\begin{array}{nghiempt}\frac{a}{4}=10\\\frac{b}{6}=10\\\frac{c}{8}=10\end{array}\right.\)
\(\left[\begin{array}{nghiempt}a=10\times4\\b=10\times6\\c=10\times8\end{array}\right.\)
\(\left[\begin{array}{nghiempt}a=40\\b=60\\c=80\end{array}\right.\)
Vậy số cây trồng được của lớp 8A, 8B, 8C theo thứ tự là 40 cây, 60 cây và 80 cây.
Bài 3 (1,5đ): Tìm x, biết
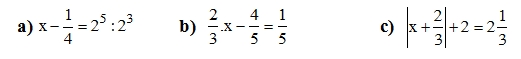
\(x-\frac{1}{4}=2^2\) \(\frac{2}{3}x=\frac{1}{5}+\frac{4}{5}\) \(\left|x+\frac{2}{3}\right|+\frac{6}{3}=\frac{7}{3}\)
\(x-\frac{1}{4}=4\) \(\frac{2}{3}x=\frac{5}{5}\) \(\left|x+\frac{2}{3}\right|=\frac{9}{3}-\frac{7}{3}\)
\(x=\frac{16}{4}+\frac{1}{4}\) \(x=1\div\frac{2}{3}\) \(\left|x+\frac{2}{3}\right|=\frac{2}{3}\)
\(x=\frac{17}{4}\) \(x=1\times\frac{3}{2}\) \(x+\frac{2}{3}=\pm\frac{2}{3}\)
\(x=\frac{3}{2}\) \(\left[\begin{array}{nghiempt}x+\frac{2}{3}=\frac{2}{3}\\x+\frac{2}{3}=-\frac{2}{3}\end{array}\right.\)
\(\left[\begin{array}{nghiempt}x=\frac{2}{3}-\frac{2}{3}\\x=-\frac{2}{3}-\frac{2}{3}\end{array}\right.\)
\(\left[\begin{array}{nghiempt}x=0\\x=-\frac{4}{3}\end{array}\right.\)
Bài 4 (1đ): So sánh các số sau: 2550 và 2300
2550 > 2300
Bài 5 (1đ): Cho N = 9/ (√x -5). Tìm x ∈ Z để N có giá trị nguyên.
\(N\in Z\)
\(\Leftrightarrow9⋮\sqrt{x}-5\)
\(\Leftrightarrow\sqrt{x}-5\in\text{Ư}\left(9\right)=\left\{-9;-3;-1;1;3;9\right\}\)
\(\Leftrightarrow\sqrt{x}\in\left\{-4;2;4;6;8;14\right\}\)
\(\Leftrightarrow x\in\left\{\sqrt{-4};\sqrt{2};\sqrt{4};\sqrt{6};\sqrt{8};\sqrt{14}\right\}\)
mà \(x\in Z\)
=> x = 2

baif2 : a ) 49+x=51
x=51-49
x=2
b) x-17=15-x
x+x=17+15
2x=32
=>x=32:2
=>x=16
c) 56-6.(13-x)=116
6(13-x)=56-116
6(13-x) = -60
=>13-x=-60:6
13-x = -10
=> x=13-(-10) = 13+10
=> x=23

Bài 1:
a) \(A=75\left(1+4+4^2+...+4^{100}\right)+25\)
Ta thấy 75.4 = 300. Vậy nên \(A=75+300+300.4+300.4^2+....+300.4^{99}+25\)
\(A=300\left(1+4+4^2+...+4^{99}\right)+\left(75+25\right)\)
\(A=300\left(1+4+4^2+...+4^{99}\right)+100⋮100\)
Vậy A chia hết 100.
b) \(x^2+y^2=2y-1\Leftrightarrow x^2+\left(y^2-2y+1\right)=0\Leftrightarrow x^2+\left(y-1\right)^2=0\)
Vậy thì \(\hept{\begin{cases}x^2=0\\\left(y-1\right)^2=0\end{cases}}\Leftrightarrow\hept{\begin{cases}x=0\\y=1\end{cases}}\)
Bài 2:
Từ đề bài ta có:
\(a\left(a+b+c\right)+b\left(a+b+c\right)+c\left(a+b+c\right)=\left(a+b+c\right)^2=20+24-28=16\)
\(\Rightarrow\orbr{\begin{cases}a+b+c=4\\a+b+c=-4\end{cases}}\)
TH1: a + b + c = 4; khi đó ta có:
\(\hept{\begin{cases}a=20:\left(a+b+c\right)=5\\b=24:\left(a+b+c\right)=6\\c=-28:\left(a+b+c\right)=-7\end{cases}}\)
Vậy a = 5; b = 6 và c = -7.
TH1: a + b + c = -4; khi đó ta có:
\(\hept{\begin{cases}a=20:\left(a+b+c\right)=-5\\b=24:\left(a+b+c\right)=-6\\c=-28:\left(a+b+c\right)=7\end{cases}}\)
Vậy a = -5; b = -6 và c = 7.








Chọn đáp án B