Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

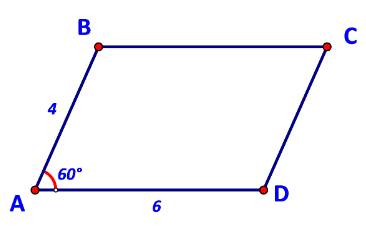
a) \(\overrightarrow {BD} = \overrightarrow {AD} - \overrightarrow {AB} ;\;\overrightarrow {AC} = \overrightarrow {AB} + \overrightarrow {AD} .\)
b) \(\overrightarrow {AB} .\overrightarrow {AD} = 4.6.\cos \widehat {BAD} = 24.\cos {60^o} = 12.\)
\(\begin{array}{l}\overrightarrow {AB} .\overrightarrow {AC} = \overrightarrow {AB} (\overrightarrow {AB} + \overrightarrow {AD} ) = {\overrightarrow {AB} ^2} + \overrightarrow {AB} .\overrightarrow {AD} = {4^2} + 12 = 28.\\\overrightarrow {BD} .\overrightarrow {AC} = (\overrightarrow {AD} - \overrightarrow {AB} )(\overrightarrow {AB} + \overrightarrow {AD} ) = {\overrightarrow {AD} ^2} - {\overrightarrow {AB} ^2} = {6^2} - {4^2} = 20.\end{array}\)
c) Áp dụng định lí cosin cho tam giác ABD ta có:
\(\begin{array}{l}\quad \;B{D^2} = A{B^2} + A{D^2} - 2.AB.AD.\cos A\\ \Leftrightarrow B{D^2} = {4^2} + {6^2} - 2.4.6.\cos {60^o} = 28\\ \Leftrightarrow BD = 2\sqrt 7 .\end{array}\)
Áp dụng định lí cosin cho tam giác ABC ta có:
\(\begin{array}{l}\quad \;A{C^2} = A{B^2} + B{C^2} - 2.AB.BC.\cos B\\ \Leftrightarrow A{C^2} = {4^2} + {6^2} - 2.4.6.\cos {120^o} = 76\\ \Leftrightarrow AC = 2\sqrt {19} .\end{array}\)


Ta có :
\(\widehat{ABD}=\widehat{ADB}\)
\(\widehat{ABD}=\widehat{BDC}\)
\(\Rightarrow\widehat{BDC}=\widehat{ADB}\)
Suy ra \(\widehat{BAD}=\pi-2\widehat{BDC}\)
Từ đó ta có :
\(\tan\widehat{BAD}=-\tan2\widehat{BDC}=-\dfrac{2\tan\widehat{BDC}}{1-\tan^2\widehat{BDC}}=-\dfrac{2.\dfrac{3}{4}}{1-9\cdot16}=-\dfrac{3}{2}.\dfrac{16}{7}=-\dfrac{24}{7}\)Vì \(\dfrac{\pi}{2}< \widehat{BAD}< \pi\) nên \(\cos\widehat{BAD}< 0\)
Do đó : \(\cos\widehat{BAD}=-\dfrac{1}{\sqrt{1+\tan^2\widehat{BAD}}}=-\dfrac{1}{\sqrt{1+\dfrac{576}{49}}}=-\dfrac{7}{25}\)
\(\sin\widehat{BAD}=\cos\widehat{BAD}\tan\widehat{BAD}=\dfrac{-7}{25}.\dfrac{-24}{7}=\dfrac{24}{25}\)

Xét ΔABD có
\(cosBAD=\dfrac{AB^2+AD^2-BD^2}{2\cdot AB\cdot AD}\)
=>\(8^2+6^2-BD^2=2\cdot8\cdot6\cdot cos60=48\)
=>\(BD^2=100-48=52\)
=>\(BD=2\sqrt{13}\left(cm\right)\)
Xét ΔBAC có \(cosABC=\dfrac{BA^2+BC^2-AC^2}{2\cdot BA\cdot BC}\)
=>\(8^2+6^2-AC^2=2\cdot8\cdot6\cdot cos120=-48\)
=>\(AC^2=148\)
=>\(AC=2\sqrt{37}\left(cm\right)\)

Câu 3:
\(\left|\overrightarrow{AC}+\overrightarrow{AH}\right|=\sqrt{AC^2+AH^2+2\cdot AC\cdot AH\cdot cos30}\)
\(=\sqrt{a^2+\left(\dfrac{a\sqrt{3}}{2}\right)^2+2\cdot a\cdot\dfrac{a\sqrt{3}}{2}\cdot\dfrac{\sqrt{3}}{2}}\)
\(=\sqrt{a^2+\dfrac{3}{4}a^2+\dfrac{3a^2}{4}}=\dfrac{\sqrt{7}}{2}a\)

Gọi E là giao điểm của AC và BD
Hình vẽ:
\(\overrightarrow{MN}=\overrightarrow{DN}-\overrightarrow{DM}=\dfrac{2}{3}\overrightarrow{DB}+\dfrac{3}{4}\overrightarrow{AD}\)
\(=\dfrac{4}{3}\overrightarrow{EB}+\dfrac{3}{4}\overrightarrow{BC}\)
\(=\dfrac{4}{3}\left(\overrightarrow{AB}-\overrightarrow{AE}\right)+\dfrac{3}{4}\left(\overrightarrow{AC}-\overrightarrow{AB}\right)\)
\(=\dfrac{4}{3}\left(\overrightarrow{AB}-\dfrac{1}{2}\overrightarrow{AC}\right)+\dfrac{3}{4}\left(\overrightarrow{AC}-\overrightarrow{AB}\right)=\dfrac{7}{12}\overrightarrow{AB}+\dfrac{1}{2}\overrightarrow{AC}\)
\(\overrightarrow{MC}=\overrightarrow{MD}+\overrightarrow{DC}=\dfrac{3}{4}\overrightarrow{AD}+\overrightarrow{AB}\)
\(=\dfrac{3}{4}\overrightarrow{BC}+\overrightarrow{AB}\)
\(=\dfrac{3}{4}\left(\overrightarrow{AC}-\overrightarrow{AB}\right)+\overrightarrow{AB}\)
\(=\dfrac{1}{4}\overrightarrow{AB}+\dfrac{3}{4}\overrightarrow{AC}\)
\(\overrightarrow{MB}=\overrightarrow{AB}-\overrightarrow{AM}=\overrightarrow{AB}-\dfrac{1}{4}\overrightarrow{AD}\)
\(=\overrightarrow{AB}-\dfrac{1}{4}\overrightarrow{BC}\)
\(=\overrightarrow{AB}-\dfrac{1}{4}\left(\overrightarrow{AC}-\overrightarrow{AB}\right)\)
\(=\dfrac{5}{4}\overrightarrow{AB}-\dfrac{1}{4}\overrightarrow{AC}\)

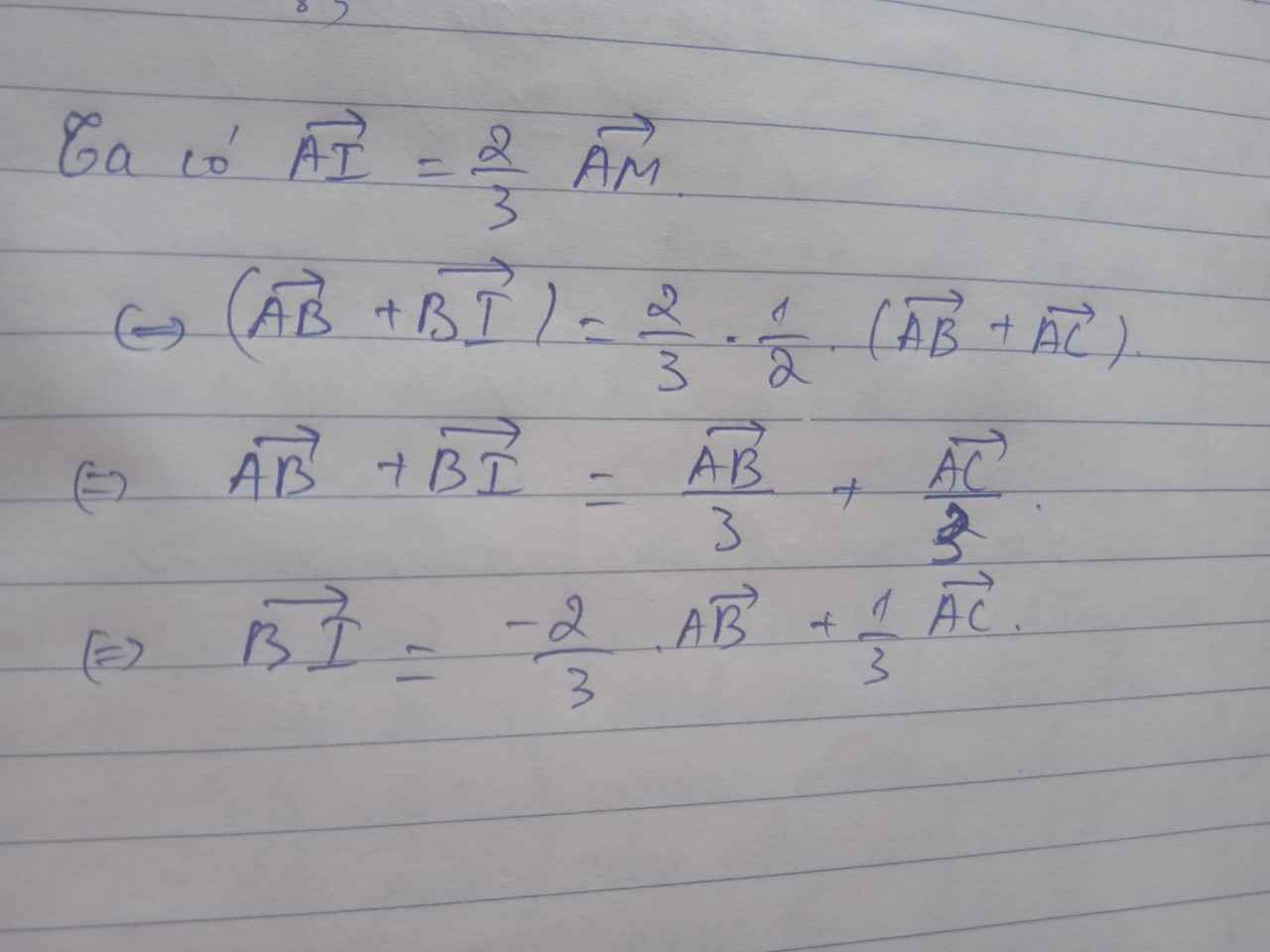
\(AC^2+BD^2=2\left(AB^2+AD^2\right)\)
\(\Leftrightarrow AB^2+AC^2=5BD^2\)
Áp dụng BĐT Cauchy:
\(cosBAD=\dfrac{AB^2+AD^2-BD^2}{2AB.AD}\ge\dfrac{4BD^2}{AB^2+AD^2}=\dfrac{4BD^2}{5BD^2}=\dfrac{4}{5}\)
\(\Rightarrow sinBAD=\sqrt{1-cos^2BAD}\le\sqrt{1-\dfrac{16}{25}}=\dfrac{3}{5}\)
\(\Rightarrow\dfrac{1}{sinBAD}\ge\dfrac{5}{3}\)
\(\Rightarrow cotBAD=\dfrac{cosBAD}{sinBAD}\ge\dfrac{4}{5}.\dfrac{5}{3}=\dfrac{4}{3}\)