MA
1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
MA
1
AH
Akai Haruma
Giáo viên
28 tháng 3 2022
Lời giải:
Đạo hàm \(y'=\frac{-1}{2\sqrt{4-x}}+\frac{1}{2\sqrt{4+x}}\)
Đoạn tìm đạo hàm tại $y'\geq 0$ ý bạn là gì nhỉ?
HQ
Hà Quang Minh
Giáo viên
22 tháng 9 2023
Đặt \(h\left( x \right) = f\left( x \right) + g\left( x \right) = \frac{1}{{x - 1}} + \sqrt {4 - x} \). Ta có:
\(\begin{array}{l}h\left( 2 \right) = \frac{1}{{2 - 1}} + \sqrt {4 - 2} = 1 + \sqrt 2 \\\mathop {\lim }\limits_{x \to 2} h\left( x \right) = \mathop {\lim }\limits_{x \to x} \left( {\frac{1}{{x - 1}} + \sqrt {4 - x} } \right) = \frac{1}{{2 - 1}} + \sqrt {4 - 2} = 1 + \sqrt 2 \end{array}\)
Vì \(\mathop {\lim }\limits_{x \to 2} h\left( x \right) = h\left( 2 \right)\) nên hàm số \(y = f\left( x \right) + g\left( x \right)\) liên tục tại \(x = 2\).
HQ
Hà Quang Minh
Giáo viên
22 tháng 9 2023
a) • \(y = f\left( x \right) = \frac{1}{{x - 1}}\)
ĐKXĐ: \(x - 1 \ne 0 \Leftrightarrow x \ne 1\)
Vậy hàm số có tập xác định: \(D = \mathbb{R}\backslash \left\{ 1 \right\}\).
• \(y = g\left( x \right) = \sqrt {4 - x} \)
ĐKXĐ: \(4 - x \ge 0 \Leftrightarrow x \le 4\)
Vậy hàm số có tập xác định: \(D = \left( { - \infty ;4} \right]\).
b) • Với mọi \({x_0} \in \left( { - \infty ;1} \right)\), ta có:
\(\mathop {\lim }\limits_{x \to {x_0}} f\left( x \right) = \mathop {\lim }\limits_{x \to {x_0}} \frac{1}{{x - 1}} = \frac{{\mathop {\lim }\limits_{x \to {x_0}} 1}}{{\mathop {\lim }\limits_{x \to {x_0}} x - \mathop {\lim }\limits_{x \to {x_0}} 1}} = \frac{1}{{{x_0} - 1}} = f\left( {{x_0}} \right)\)
Vậy hàm số \(y = f\left( x \right)\) liên tục tại mọi điểm \({x_0} \in \left( { - \infty ;1} \right)\).
Tương tự ta có hàm số \(y = f\left( x \right)\) liên tục tại mọi điểm \({x_0} \in \left( {1; + \infty } \right)\).
Ta có: Hàm số không xác định tại điểm \({x_0} = 1\)
\(\mathop {\lim }\limits_{x \to {1^ + }} f\left( x \right) = \mathop {\lim }\limits_{x \to {1^ + }} \frac{1}{{x - 1}} = + \infty ;\mathop {\lim }\limits_{x \to {1^ - }} f\left( x \right) = \mathop {\lim }\limits_{x \to {1^ - }} \frac{1}{{x - 1}} = - \infty \)
Vì \(\mathop {\lim }\limits_{x \to {1^ + }} f\left( x \right) \ne \mathop {\lim }\limits_{x \to {1^ - }} f\left( x \right)\) nên không tồn tại \(\mathop {\lim }\limits_{x \to 1} f\left( x \right)\).
Vậy hàm số \(y = f\left( x \right)\) không liên tục tại điểm \({x_0} = 1\).
• Với mọi \({x_0} \in \left( { - \infty ;4} \right)\), ta có:
\(\mathop {\lim }\limits_{x \to {x_0}} g\left( x \right) = \mathop {\lim }\limits_{x \to {x_0}} \sqrt {4 - x} = \sqrt {\mathop {\lim }\limits_{x \to {x_0}} 4 - \mathop {\lim }\limits_{x \to {x_0}} x} = \sqrt {4 - {x_0}} = g\left( {{x_0}} \right)\)
Vậy hàm số \(y = g\left( x \right)\) liên tục tại mọi điểm \({x_0} \in \left( { - \infty ;4} \right)\).
Ta có: \(g\left( 4 \right) = \sqrt {4 - 4} = 0\)
\(\mathop {\lim }\limits_{x \to {4^ - }} g\left( x \right) = \mathop {\lim }\limits_{x \to {4^ - }} \sqrt {4 - x} = \sqrt {\mathop {\lim }\limits_{x \to {4^ - }} 4 - \mathop {\lim }\limits_{x \to {4^ - }} x} = \sqrt {4 - 4} = 0 = g\left( 4 \right)\)
Vậy hàm số \(y = g\left( x \right)\) liên tục tại điểm \({x_0} = 4\).
Hàm số không xác định tại mọi \({x_0} \in \left( {4; + \infty } \right)\) nên hàm số \(y = g\left( x \right)\) không liên tục tại mọi điểm \({x_0} \in \left( {4; + \infty } \right)\).
Vậy hàm số \(y = g\left( x \right)\) liên tục trên nửa khoảng \(\left( { - \infty ;4} \right]\).
AH
Akai Haruma
Giáo viên
27 tháng 2 2022
Lời giải:
Để hàm số trên liên tục tại $x_0=0$ thì:
\(\lim\limits_{x\to 0+}f(x)=\lim\limits_{x\to 0-}f(x)=f(0)\)
\(\Leftrightarrow \lim\limits_{x\to 0+}(a+\frac{4-x}{x+2})=\lim\limits_{x\to 0-}(\frac{\sqrt{1-x}+\sqrt{1+x}}{x})=a+2\)
\(\Leftrightarrow a+2=\lim\limits_{x\to 0-}\frac{\sqrt{1-x}+\sqrt{1+x}}{x}\)
Mà \(\lim\limits_{x\to 0-}\frac{\sqrt{1-x}+\sqrt{1+x}}{x}=-\infty \) nên không tồn tại $a$ để hàm số liên tục tại $x_0=0$
10 tháng 9 2023
a) \(x^3-4x^2-5x+6=\sqrt[3]{7x^2+9x-4}\)
\(\Leftrightarrow-7x^2-9x+4+x^3+3x^2+4x+2=\sqrt[3]{7x^2+9x-4}\)
\(\Leftrightarrow-\left(7x^2+9x-4\right)+\left(x+1\right)^3+x+1=\sqrt[3]{7x^2+9x-4}\) (*)
Đặt \(\sqrt[3]{7x^2+9x-4}=a;x+1=b\)
Khi đó (*) \(\Leftrightarrow-a^3+b^3+b=a\)
\(\Leftrightarrow\left(b-a\right).\left(b^2+ab+a^2+1\right)=0\)
\(\Leftrightarrow b=a\)
Hay \(x+1=\sqrt[3]{7x^2+9x-4}\)
\(\Leftrightarrow\left(x+1\right)^3=7x^2+9x-4\)
\(\Leftrightarrow x^3-4x^2-6x+5=0\)
\(\Leftrightarrow x^3-4x^2-5x-x+5=0\)
\(\Leftrightarrow\left(x-5\right)\left(x^2+x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=5\\x=\dfrac{-1\pm\sqrt{5}}{2}\end{matrix}\right.\)
15 tháng 9 2023
1) \(f\left(x\right)=2x-5\)
\(f'\left(x\right)=2\)
\(\Rightarrow f'\left(4\right)=2\)
2) \(y=x^2-3\sqrt[]{x}+\dfrac{1}{x}\)
\(\Rightarrow y'=2x-\dfrac{3}{2\sqrt[]{x}}-\dfrac{1}{x^2}\)
3) \(f\left(x\right)=\dfrac{x+9}{x+3}+4\sqrt[]{x}\)
\(\Rightarrow f'\left(x\right)=\dfrac{1.\left(x+3\right)-1.\left(x+9\right)}{\left(x-3\right)^2}+\dfrac{4}{2\sqrt[]{x}}\)
\(\Rightarrow f'\left(x\right)=\dfrac{x+3-x-9}{\left(x-3\right)^2}+\dfrac{2}{\sqrt[]{x}}\)
\(\Rightarrow f'\left(x\right)=\dfrac{12}{\left(x-3\right)^2}+\dfrac{2}{\sqrt[]{x}}\)
\(\Rightarrow f'\left(x\right)=2\left[\dfrac{6}{\left(x-3\right)^2}+\dfrac{1}{\sqrt[]{x}}\right]\)
\(\Rightarrow f'\left(1\right)=2\left[\dfrac{6}{\left(1-3\right)^2}+\dfrac{1}{\sqrt[]{1}}\right]=2\left(\dfrac{3}{2}+1\right)=2.\dfrac{5}{2}=5\)
HT
8 tháng 4 2021
1/ \(y'=\dfrac{\left(\sqrt{x+1}\right)'x-x'\sqrt{x+1}}{x^2}=\dfrac{\dfrac{x}{2\sqrt{x+1}}-\sqrt{x+1}}{x^2}=\dfrac{-x-2}{2x^2\sqrt{x+1}}\)
2/ \(y'=\dfrac{1-x^2-\left(1-x^2\right)'x}{\left(1-x^2\right)^2}=\dfrac{1+x^2}{\left(1-x^2\right)^2}\)
3/ \(y'=\dfrac{-\left(x-\sqrt{x+1}\right)'}{\left(x-\sqrt{x+1}\right)^2}=\dfrac{-1+\dfrac{1}{2\sqrt{x+1}}}{\left(x-\sqrt{x+1}\right)^2}\)
4/ \(y'=f'\left(x\right)=2x-\dfrac{2x}{x^4}=2x-\dfrac{2}{x^3}\)
\(y'=0\Leftrightarrow\dfrac{2x^4-2}{x^3}=0\Leftrightarrow x=\pm1\)
5/ \(y'=\dfrac{\dfrac{1}{2\sqrt{1+x}}}{2\sqrt{1+\sqrt{1+x}}}\Rightarrow f\left(x\right).f'\left(x\right)=\sqrt{1+\sqrt{1+x}}.\dfrac{1}{4\sqrt{1+x}.\sqrt{1+\sqrt{1+x}}}=\dfrac{1}{4\sqrt{1+x}}=\dfrac{1}{2\sqrt{2}}\)
\(\Leftrightarrow2\sqrt{1+x}=\sqrt{2}\Leftrightarrow1+x=\dfrac{1}{2}\Leftrightarrow x=-\dfrac{1}{2}\)
Hãy nhớ câu tính đạo hàm này, bởi nó liên quan đến nguyên hàm sau này sẽ học
17 tháng 9 2023
1) \(y=x^2-3\sqrt[]{x}+\dfrac{1}{x}\)
\(\Rightarrow y=2x-\dfrac{3}{2\sqrt[]{x}}-\dfrac{1}{x^2}\)
2) \(f\left(x\right)=\dfrac{x+9}{x+3}+4\sqrt[]{x}\)
\(\Rightarrow f'\left(x\right)=\dfrac{1.\left(x+3\right)-1\left(x+9\right)}{\left(x+3\right)^2}+\dfrac{2}{\sqrt[]{x}}\)
\(\Rightarrow f'\left(x\right)=\dfrac{x+3-x-9}{\left(x+3\right)^2}+\dfrac{2}{\sqrt[]{x}}\)
\(\Rightarrow f'\left(x\right)=\dfrac{-6}{\left(x+3\right)^2}+\dfrac{2}{\sqrt[]{x}}\)
\(\Rightarrow f'\left(1\right)=\dfrac{-6}{\left(1+3\right)^2}+\dfrac{2}{\sqrt[]{1}}=-\dfrac{3}{8}+2=\dfrac{13}{8}\)
NV
Nguyễn Việt Lâm
Giáo viên
23 tháng 4 2022
\(y'=\dfrac{\left(x+\sqrt{x^2+1}\right)'}{2\sqrt{x+\sqrt{x^2+1}}}=\dfrac{1+\dfrac{x}{\sqrt{x^2+1}}}{2\sqrt{x+\sqrt{x^2+1}}}=\dfrac{x+\sqrt{x^2+1}}{2\sqrt{x^2+1}.\sqrt{x+\sqrt{x^2+1}}}\)
\(=\dfrac{\sqrt{x+\sqrt{x^2+1}}}{2\sqrt{x^2+1}}\)
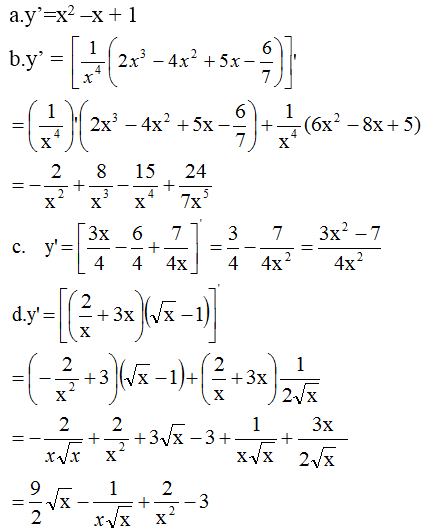
y' > 0 ⇔ \(\dfrac{-1}{2\sqrt{4-x}}+\dfrac{1}{2\sqrt{4+x}}>0\)
⇔ \(\dfrac{1}{2\sqrt{4+x}}>\dfrac{1}{2\sqrt{4-x}}\)
⇔ \(\dfrac{1}{\sqrt{4+x}}>\dfrac{1}{\sqrt{4-x}}\)
⇔ \(\left\{{}\begin{matrix}4-x>0\\4+x>0\\4+x< 4-x\end{matrix}\right.\)
⇔ \(\left\{{}\begin{matrix}4-x>0\\4+x>0\\x< 0\end{matrix}\right.\) ⇔ -4 < x < 0.
Bạn thêm dấu = ở số 0 vào nhé