Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a)\(y=\sqrt{3}sinx+cosx=2\left(\dfrac{\sqrt{3}}{2}sinx+\dfrac{1}{2}cosx\right)\)\(=2\left(sinx.cos\dfrac{\pi}{6}+cosx.sin\dfrac{\pi}{6}\right)\)\(=2sin\left(x+\dfrac{\pi}{6}\right)\)
Có \(-1\le sin\left(x+\dfrac{\pi}{6}\right)\le1\) \(\Leftrightarrow-2\le2sin\left(x+\dfrac{\pi}{6}\right)\le2\)
\(\Leftrightarrow-2\le y\le2\)
miny=-2 \(\Leftrightarrow sin\left(x+\dfrac{\pi}{6}\right)=-1\) \(\Leftrightarrow x+\dfrac{\pi}{6}=-\dfrac{\pi}{2}+2k\pi\left(k\in Z\right)\) \(\Leftrightarrow x=-\dfrac{2\pi}{3}+k2\pi\left(k\in Z\right)\)
maxy=2\(\Leftrightarrow sin\left(x+\dfrac{\pi}{6}\right)=1\) \(\Leftrightarrow x+\dfrac{\pi}{6}=\dfrac{\pi}{2}+k2\pi\left(k\in Z\right)\)\(\Leftrightarrow x=\dfrac{\pi}{3}+k2\pi\left(k\in Z\right)\)
b) \(y=sin2x-cos2x=\sqrt{2}sin\left(2x-\dfrac{\pi}{4}\right)\)
Có \(\sqrt{2}\ge\sqrt{2}sin\left(2x-\dfrac{\pi}{4}\right)\ge-\sqrt{2}\)
\(\Leftrightarrow\sqrt{2}\ge y\ge-\sqrt{2}\)
miny=\(-\sqrt{2}\) \(\Leftrightarrow sin\left(2x-\dfrac{\pi}{4}\right)=-1\)\(\Leftrightarrow2x-\dfrac{\pi}{4}=-\dfrac{\pi}{2}+k2\pi\left(k\in Z\right)\)\(\Leftrightarrow x=-\dfrac{\pi}{8}+k\pi\left(k\in Z\right)\)
maxy=\(\sqrt{2}\Leftrightarrow sin\left(2x-\dfrac{\pi}{4}\right)=1\)\(\Leftrightarrow x=\dfrac{3\pi}{8}+k\pi\left(k\in Z\right)\)
c) \(y=3sinx+4cosx=5\left(\dfrac{3}{5}sinx+\dfrac{4}{5}cosx\right)\)
Đặt \(cosa=\dfrac{3}{5}\) và \(sina=\dfrac{4}{5}\)(vì cos2a+sin2a=1)
\(y=5\left(sinx.cosa+cosx.sina\right)\)\(=5sin\left(x+a\right)\)
\(\Rightarrow-5\le y\le5\)
miny=-5 <=> \(sin\left(x+a\right)=-1\)\(\Leftrightarrow x=-\dfrac{\pi}{2}-arc.sina+k2\pi\left(k\in Z\right)\)
maxy=5 <=> \(sin\left(x+a\right)=1\)\(\Leftrightarrow x=\dfrac{\pi}{2}-arc.sina+k2\pi\left(k\in Z\right)\)
(P/s1:cái x ở câu c ấy trông nó ngu ngu??
P/s2:sau khi load lại câu hỏi ở 1 tab khác ,thấy 1 câu trả lời nhưng vẫn đăng vì cảm thấy bỏ đi hơi phí :?)
Áp dụng quy tắc sau: Nếu \(a\sin x+b\cos y=c\Leftrightarrow a^2+b^2\ge c^2\)
a/ \(3+1\ge y^2\Leftrightarrow4\ge y^2\Leftrightarrow-2\le y\le2\)
\(y_{max}=2\Leftrightarrow\sqrt{3}\sin x+\cos x=2\Leftrightarrow\dfrac{\sqrt{3}}{2}\sin x+\dfrac{1}{2}\cos x=1\Leftrightarrow\cos\dfrac{\pi}{6}.\sin x+\sin\dfrac{\pi}{6}.\cos x=1\)
\(\Rightarrow\sin\left(x+\dfrac{\pi}{6}\right)=1\Leftrightarrow x+\dfrac{\pi}{6}=\dfrac{\pi}{2}+k2\pi\Leftrightarrow x=\dfrac{\pi}{3}+k2\pi\)
\(y_{min}=-2\Leftrightarrow\sin\left(x+\dfrac{\pi}{6}\right)=-1\Leftrightarrow x+\dfrac{\pi}{6}=-\dfrac{\pi}{2}+k2\pi\Leftrightarrow x=-\dfrac{2}{3}\pi+k2\pi\)

\(0\le cos^2x\le1\Rightarrow2\le3-cos^2x\le3\)
\(\Rightarrow\frac{8}{3}\le y\le4\)
\(y_{min}=\frac{8}{3}\) khi \(cosx=0\)
\(y_{max}=4\) khi \(cos^2x=1\)
b/ \(0\le sin^23x\le1\Rightarrow1\le\sqrt{2-sin^23x}\le\sqrt{2}\)
\(\Rightarrow\frac{1}{\sqrt{2}}\le y\le1\)
\(y_{min}=\frac{1}{\sqrt{2}}\) khi \(sin3x=0\)
\(y_{max}=1\) khi \(sin^23x=1\)
c/ \(y=\sqrt{3}\left(sin^2x-cos^2x\right)\left(sin^2x+cos^2x\right)+sin2x+1\)
\(=-\sqrt{3}\left(cos^2x-sin^2x\right)+sin2x+1\)
\(=-\sqrt{3}cos2x+sin2x+1\)
\(=2\left(\frac{1}{2}sin2x-\frac{\sqrt{3}}{2}cos2x\right)+1=2sin\left(2x-\frac{\pi}{3}\right)+1\)
Do \(-1\le sin\left(2x-\frac{\pi}{3}\right)\le1\Rightarrow-1\le y\le3\)
\(y_{min}=-1\) khi \(sin\left(2x-\frac{\pi}{3}\right)=-1\)
\(y_{max}=3\) khi \(sin\left(2x-\frac{\pi}{3}\right)=1\)

Ta có:
\(-1\le\sin2x\le1\)
=> \(\sqrt{4-2.\left(1\right)^5}-8\le\sqrt{4-2.\left(\sin2x\right)^5}-8\le\sqrt{4-2.\left(-1\right)^5}-8\)
=> \(\sqrt{2}-8\le\sqrt{4-2.\left(\sin2x\right)^5}-8\le\sqrt{6}-8\)
=> tìm ddc min và max


a/ \(0\le cos^2x\le1\Rightarrow2\le y\le\sqrt{7}\)
\(y_{min}=2\) khi \(cos^2x=1\)
\(y_{max}=\sqrt{7}\) khi \(cos^2x=0\)
b/ \(y=\frac{2}{1+tan^2x}=\frac{2}{\frac{1}{cos^2x}}=2cos^2x\le2\)
\(\Rightarrow y_{max}=2\) khi \(cos^2x=1\)
\(y_{min}\) ko tồn tại
c/ \(y=1-cos2x+\sqrt{3}sin2x=2\left(\frac{\sqrt{3}}{2}sin2x-\frac{1}{2}cos2x\right)+1\)
\(y=2sin\left(2x-\frac{\pi}{6}\right)+1\)
Do \(-1\le sin\left(2x-\frac{\pi}{6}\right)\le1\Rightarrow-1\le y\le3\)

2.Biểu thức luôn xác định
\(y=\dfrac{4}{\sqrt{5-2cos^2sin^2x}}=\dfrac{4}{\sqrt{5-\dfrac{1}{2}sin^22x}}\)
Có: \(1\ge sin^22x\ge0\)
\(\Leftrightarrow-\dfrac{1}{2}\le-\dfrac{1}{2}sin^22x\le0\)
\(\Leftrightarrow\dfrac{3\sqrt{2}}{2}\le\sqrt{5-\dfrac{1}{2}sin^22x}\le\sqrt{5}\)
\(\Rightarrow\dfrac{4\sqrt{2}}{3}\ge y\ge\dfrac{4\sqrt{5}}{5}\)
miny=\(\dfrac{4\sqrt{5}}{5}\) \(\Leftrightarrow sin2x=0\)\(\Leftrightarrow x=\dfrac{k\pi}{2}\left(k\in Z\right)\)
maxy=\(\dfrac{4\sqrt{2}}{3}\Leftrightarrow\left[{}\begin{matrix}sin2x=1\\sin2x=-1\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{4}+k\pi\\x=\dfrac{-\pi}{4}+k\pi\end{matrix}\right.\)\(\left(k\in Z\right)\)
1.Biểu thức luôn xác định
Xét \(sin2x=0\) \(\Leftrightarrow x=\dfrac{k\pi}{2}\left(k\in Z\right)\) khi đó \(y=-6\)
Xét \(sin2x\ne0\)
=> \(1\ge sin^52x\ge-1\)
\(\Leftrightarrow4-1\le4-sin^52x\le4+1\)
\(\Leftrightarrow\sqrt{3}\le\sqrt{4-sin^52x}\le\sqrt{5}\)
\(\Leftrightarrow\sqrt{3}-8\le y\le\sqrt{5}-8\)
\(y=\sqrt{3}-8< -6\) , \(y=\sqrt{5}-8>-6\)
=>min= \(\sqrt{3}-8\) \(\Leftrightarrow sin2x=1\left(tm\right)\) \(\Leftrightarrow x=\dfrac{\pi}{4}+k\pi\left(k\in Z\right)\)
maxy=\(\sqrt{5}-8\)\(\Leftrightarrow sin2x=-1\left(tm\right)\) \(\Leftrightarrow x=-\dfrac{\pi}{4}+k\pi\left(k\in Z\right)\)
(câu này e ko chắc)
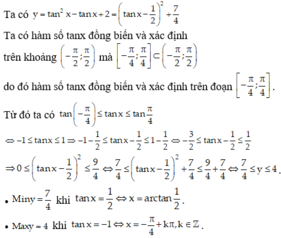

Đáp án A