Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a) \(\overrightarrow {OA} + 3\overrightarrow {OB} = \overrightarrow 0 \)
\(\begin{array}{l}
\overrightarrow {OA} + 3\overrightarrow {OB} = \vec 0\\
\Leftrightarrow \overrightarrow {OB} + \overrightarrow {BA} + 3\overrightarrow {OB} = \vec 0\\
\Leftrightarrow \overrightarrow {OB} + 3\overrightarrow {OB} = - \overrightarrow {BA} \\
\Leftrightarrow 4\overrightarrow {OB} = \overrightarrow {AB} \\
\Leftrightarrow \overrightarrow {OB} = \frac{1}{4}\overrightarrow {AB}
\end{array}\)
Vậy O thuộc đoạn AB sao cho \(OB = \frac{1}{4}AB\)
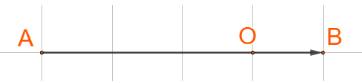
b) Ta có:
\(\begin{array}{l}
\overrightarrow {MA} + 3\overrightarrow {MB} = \left( {\overrightarrow {MO} + \overrightarrow {OA} } \right) + 3\left( {\overrightarrow {MO} + \overrightarrow {OB} } \right)\\
= \left( {\overrightarrow {MO} + 3\overrightarrow {MO} } \right) + \left( {\overrightarrow {OA} + 3\overrightarrow {OB} } \right)\\
= 4\overrightarrow {MO} + \overrightarrow 0 = 4\overrightarrow {MO} . (đpcm)
\end{array}\)

Tham khảo:
a) Ta có: \(\overrightarrow {MA} + \overrightarrow {MB} + 2\overrightarrow {MC} = \overrightarrow 0 \Leftrightarrow \overrightarrow {MA} + \left( {\overrightarrow {MA} + \overrightarrow {AB} } \right) + 2\left( {\overrightarrow {MA} + \overrightarrow {AC} } \right) = \overrightarrow 0 \)
\(\begin{array}{l} \Leftrightarrow 4\overrightarrow {MA} + \overrightarrow {AB} + 2\overrightarrow {AC} = \overrightarrow 0 \\ \Leftrightarrow 4\overrightarrow {AM} = \overrightarrow {AB} + 2\overrightarrow {AC} \\ \Leftrightarrow \overrightarrow {AM} = \frac{1}{4}\overrightarrow {AB} + \frac{1}{2}\overrightarrow {AC} \end{array}\)
Trên cạnh AB, AC lấy điểm D, E sao cho \(AD = \frac{1}{4}AB;\;\,AE = \frac{1}{2}AC\)
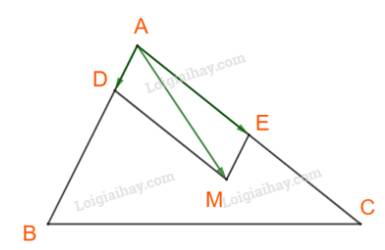
Khi đó \(\overrightarrow {AM} = \overrightarrow {AD} + \overrightarrow {AE} \) hay M là đỉnh thứ tư của hình bình hành AEMD.
b) Chứng minh rằng với mọi điểm O, ta có \(\overrightarrow {OA} + \overrightarrow {OB} + 2\overrightarrow {OC} = 4\overrightarrow {OM} \)
Với mọi điểm O, ta có: \(\left\{ \begin{array}{l}\overrightarrow {OA} = \overrightarrow {OM} + \overrightarrow {MA} ;\;\\\overrightarrow {OB} = \overrightarrow {OM} + \overrightarrow {MB} ;\;\,\\\overrightarrow {OC} = \overrightarrow {OM} + \overrightarrow {MC} \end{array} \right.\)
\(\begin{array}{l} \Rightarrow \overrightarrow {OA} + \overrightarrow {OB} + 2\overrightarrow {OC} = \left( {\overrightarrow {OM} + \overrightarrow {MA} } \right) + \left( {\overrightarrow {OM} + \overrightarrow {MB} } \right) + 2\left( {\overrightarrow {OM} + \overrightarrow {MC} } \right)\\ = 4\overrightarrow {OM} + \left( {\overrightarrow {MA} + \overrightarrow {MB} + 2\overrightarrow {MC} } \right) = 4\overrightarrow {OM} + \overrightarrow 0 = 4\overrightarrow {OM} .\end{array}\)
Vậy với mọi điểm O, ta có \(\overrightarrow {OA} + \overrightarrow {OB} + 2\overrightarrow {OC} = 4\overrightarrow {OM} \).
Tham khảo cách 2 câu a:
Cách 2:
Ta có: \(\overrightarrow {MA} + \overrightarrow {MB} + 2\overrightarrow {MC} = \overrightarrow 0 \Leftrightarrow \left( {\overrightarrow {MC} + \overrightarrow {CA} } \right) + \left( {\overrightarrow {MC} + \overrightarrow {CB} } \right) + 2\overrightarrow {MC} = \overrightarrow 0 \)
\(\begin{array}{l} \Leftrightarrow 4\overrightarrow {MC} + \overrightarrow {CA} + \overrightarrow {CB} = \overrightarrow 0 \\ \Leftrightarrow 4.\overrightarrow {CM} = \overrightarrow {CA} + \overrightarrow {CB} \end{array}\)
Gọi D là đỉnh thứ tư của hình bình hành ACBD.
Khi đó: \(\overrightarrow {CD} = \overrightarrow {CA} + \overrightarrow {CB} \)\( \Rightarrow 4.\overrightarrow {CM} = \overrightarrow {CD} \)
\( \Leftrightarrow \overrightarrow {CM} = \frac{1}{4}\overrightarrow {CD} \Leftrightarrow \overrightarrow {CM} = \frac{1}{2}\overrightarrow {CO} \)
Với O là tâm hình bình hành ACBD, cũng là trung điểm đoạn AB.
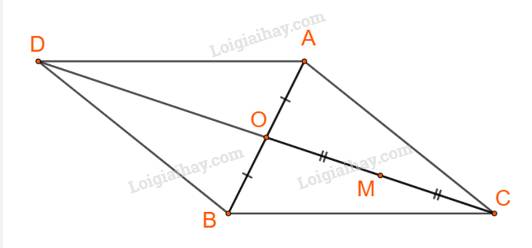
Vậy M là trung điểm của trung tuyến kẻ từ C của tam giác ABC.

A B C P N M
a)
Có: \(3\overrightarrow{OC}-\overrightarrow{OB}=3\left(\overrightarrow{OM}+\overrightarrow{MC}\right)-\left(\overrightarrow{OM}+\overrightarrow{MB}\right)\)
\(=2\overrightarrow{OM}+3\overrightarrow{MC}-\overrightarrow{MB}\)\(=2\overrightarrow{OM}+\overrightarrow{MB}-\overrightarrow{MB}=2\overrightarrow{OM}\). (Đpcm).
b)
Gọi G là trọng tâm tam giác ABC, ta chứng minh G cũng là trọng tâm tam giác MNP.
Ta có: \(\overrightarrow{GA}+\overrightarrow{GB}+\overrightarrow{GC}=\overrightarrow{0}\).
Ta cần chứng minh: \(\overrightarrow{GN}+\overrightarrow{GM}+\overrightarrow{GP}=\overrightarrow{0}\).
Thật vậy \(\overrightarrow{GN}+\overrightarrow{GM}+\overrightarrow{GP}=\overrightarrow{GC}+\overrightarrow{CN}+\overrightarrow{GB}+\overrightarrow{BM}+\overrightarrow{GA}+\overrightarrow{AP}\)
\(=\left(\overrightarrow{GA}+\overrightarrow{GB}+\overrightarrow{GC}\right)+\overrightarrow{CN}+\overrightarrow{BM}+\overrightarrow{AP}\)
\(=\overrightarrow{0}+\overrightarrow{CN}+\overrightarrow{BM}+\overrightarrow{AP}\)
\(=\dfrac{3}{4}\overrightarrow{CA}+\dfrac{3}{4}\overrightarrow{BC}+\dfrac{3}{4}\overrightarrow{AB}\)
\(=\dfrac{3}{4}\left(\overrightarrow{CA}+\overrightarrow{AB}\right)+\dfrac{3}{4}\overrightarrow{BC}\)
\(=\dfrac{3}{4}\overrightarrow{CB}+\dfrac{3}{4}\overrightarrow{BC}=\overrightarrow{0}\).
Vậy G cũng là trọng tâm tam giác MNP. (Đpcm).

câu 2 ( các kí hiệu vecto khi lm bài thỳ b tự viết nhé mk k viết kí hiệu để trả lời cho nhanh hỳ hỳ )
OA+ OB + OC = OA'+ OB' + OC'
<=> OA - OA' + OB - OB' + OC - OC' = 0
<=> A'A + B'B + C'C = 0
<=> 2 ( BA + CB + AC ) = 0
<=> 2 ( CB + BA + AC ) = 0
<=> 2 ( CA + AC ) = 0
<=> 0 = 0 ( luôn đúng )
câu 1 ( các kí hiệu vecto b cx tự viết nhá )
VT = OD + OC = OA + AD + OB + BC = OA + OB + AD + BC = BO + OB + AD + BC = 0 + AD + BC = AD + BC = VP ( đpcm)
a)
Cách 1:
Ta có: \(\overrightarrow {KA} + 2\overrightarrow {KB} = \overrightarrow 0 \).
\( \Leftrightarrow \overrightarrow {KA} = - 2\overrightarrow {KB} \)
Suy ra vecto \(\overrightarrow {KA} \) và vecto\(\;\overrightarrow {KB} \) cùng phương, ngược chiều và \(KA = 2.KB\)
\( \Rightarrow K,A,B\)thẳng hàng, K nằm giữa A và B thỏa mãn: \(KA = 2.KB\)
Cách 2:
Ta có: \(\overrightarrow {KA} + 2\overrightarrow {KB} = \overrightarrow 0 \).
\(\begin{array}{l} \Leftrightarrow \left( {\overrightarrow {KB} + \overrightarrow {BA} } \right) + 2\overrightarrow {KB} = \overrightarrow 0 \\ \Leftrightarrow 3.\overrightarrow {KB} + \overrightarrow {BA} = \overrightarrow 0 \\ \Leftrightarrow 3.\overrightarrow {KB} = \overrightarrow {AB} \\ \Leftrightarrow \overrightarrow {KB} = \frac{1}{3}\overrightarrow {AB} \end{array}\)
Vậy K thuộc đoạn AB sao cho \(KB = \frac{1}{3}AB\).
b)
Với O bất kì, ta có:
\(\frac{1}{3}\overrightarrow {OA} + \frac{2}{3}\overrightarrow {OB} = \frac{1}{3}\left( {\overrightarrow {OK} + \overrightarrow {KA} } \right) + \frac{2}{3}\left( {\overrightarrow {OK} + \overrightarrow {KB} } \right) = \left( {\frac{1}{3}\overrightarrow {OK} + \frac{2}{3}\overrightarrow {OK} } \right) + \left( {\frac{1}{3}\overrightarrow {KA} + \frac{2}{3}\overrightarrow {KB} } \right) = \overrightarrow {OK} + \frac{1}{3}\left( {\overrightarrow {KA} + 2\overrightarrow {KB} } \right) = \overrightarrow {OK}\)
Vì \(\overrightarrow {KA} + 2\overrightarrow {KB} = \overrightarrow 0 \)
Vậy với mọi điểm O, ta có \(\overrightarrow {OK} = \frac{1}{3}\overrightarrow {OA} + \frac{2}{3}\overrightarrow {OB} .\)