Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

b: Tọa độ giao là:
2x+5=x+3 và y=x+3
=>x=-2 và y=1
c: Thay x=-2 và y=1 vào (d), ta được:
m-3-6=1
=>m=10

b: Tọa độ giao điểm là:
\(\left\{{}\begin{matrix}x^2=-2x+3\\y=x^2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x^2+2x-3=0\\y=x^2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\left(x+3\right)\left(x-1\right)=0\\y=x^2\end{matrix}\right.\Leftrightarrow\left(x,y\right)\in\left\{\left(-3;9\right);\left(1;1\right)\right\}\)

a)
- Vẽ đường thẳng y = -x + 6
Cho x = 0 ⇒ y = 6 được điểm (0, 6)
Cho y = 0 ⇒ x = 6 được điểm (6, 0)
⇒ Đường thẳng y = -x + 6 đi qua các điểm (6; 0) và (0; 6).
- Lập bảng giá trị và vẽ đồ thị hàm số 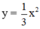
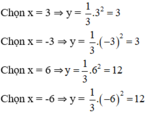
⇒ Parabol đi qua các điểm (3; 3); (-3; 3); (-6; 12); (6; 12); (0; 0).
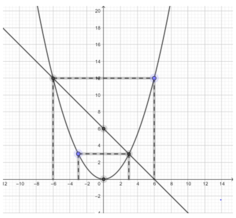
b)Xét phương trình hoành độ giao điểm


\(b,\text{PT hoành độ giao điểm: }2x=-2x+4\Leftrightarrow x=1\Leftrightarrow y=2\Leftrightarrow A\left(1;2\right)\)

b: Tọa độ giao điểm là:
\(\left\{{}\begin{matrix}-2x+3=x+2\\y=x+2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{1}{3}\\y=\dfrac{7}{3}\end{matrix}\right.\)
Lời giải:
a.
Đồ thị xanh lá: $y=2x+1$
Đồ thị xanh dương: $y=x-3$
b.
PT hoành độ giao điểm:
$y=2x+1=x-3$
$\Leftrightarrow x=-4$
$y=x-3=(-4)-3=-7$
Vậy tọa độ điểm $M$ là $(-4;-7)$