Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Lời giải:
a)
\(x(2x+1)-x^2(x+3)+x^3-x+3=2x^2+x-x^3-2x^2+x^3-x+3\)
\(=3\) không phụ thuộc vào biến (đpcm)
b)
\(4(x-6)-x^2(2+3x)+x(5x-4)+3x^2(x-1)\)
\(=4x-24-2x^2-3x^3+5x^2-4x+3x^3-3x^2\)
\(=(4x-4x)-24+(-2x^2+5x^2-3x^2)+(-3x^3+3x^3)\)
\(=-24\) không phụ thuộc vào biến.
c)
\((x^2+2x+3)(3x^2-2x+1)-3x^2(x^2+2)-4x(x^2-1)\)
\(=(3x^4-2x^3+x^2+6x^3-4x^2+2x+9x^2-6x+3)-(3x^4+6x^2)-(4x^3-4x)\)
\(=(3x^4-3x^4)+(-2x^3+6x^3-4x^3)+(x^2-4x^2+9x^2-6x^2)+(2x-6x+4x)+3\)
\(=3\) không phụ thuộc vào biến (đpcm)
a: \(=\dfrac{x^4-6x^3+12x^2-14x+3}{x^2-4x+1}\)
\(=\dfrac{x^4-4x^3+x^2-2x^3+8x^2-2x+3x^2-12x+3}{x^2-4x+1}\)
\(=x^2-2x+3\)
b: \(=\dfrac{x^5-3x^4+5x^3-x^2+3x-5}{x^2-3x+5}=x^2-1\)
c: \(=\dfrac{2x^4-5x^3+2x^2+2x-1}{x^2-x-1}\)
\(=\dfrac{2x^4-2x^3-2x^2-3x^3+3x^2+3x+x^2-x-1}{x^2-x-1}\)
\(=2x^2-3x+1\)
`@` `\text {Ans}`
`\downarrow`
`P(x)+Q(x)-R(x)`
`= 5x^2 + 5x - 4 +2x^2 - 3x + 1 - (4x^2 - x + 3)`
`= 5x^2 + 5x - 4 + 2x^2 - 3x + 1 - 4x^2 + x - 3`
`= (5x^2 + 2x^2 - 4x^2) + (5x - 3x + x) + (-4 + 1 - 3)`
`= 3x^2 + 3x - 6`
Thay `x=-1/2`
`3*(-1/2)^2 + 3*(-1/2) - 6`
`= 3*1/4 - 3/2 - 6`
`= 3/4 - 3/2 - 6`
`= -3/4 - 6 = -27/4`
Vậy, khi `x=-1/2` thì GTr của đa thức là `-27/4`
P(x)+Q(x)-R(x)
=5x^2+5x-4+2x^2-3x+1-4x^2+x-3
=2x^2+3x-6(1)
Khi x=-1/2 thì (1) sẽ là 2*1/4+3*(-1/2)-6=1/2-3/2-6=-7
uuuuuuuuuuuuuuuuuuuuuuuuuuuuuu
55555555555555555
666666666666666666666666666
88888888888888888888
a) \(\left(1+x\right)^2+\left(1-x\right)^2\)
\(=1+2x+x^2+1-2x+x^2\)
\(=2x^2+2\)
b) \(\left(x+2\right)^2+\left(1+x\right)\left(1-x\right)\)
\(=x^2+4x+4+1-x^2\)
\(=4x+5\)
c) \(\left(x-3\right)^2+3\left(x+1\right)^2\)
\(=x^2-6x+9+3x^2+6x+3\)
\(=4x^2+12\)
d)\(\left(2+3x\right)\left(3x-2\right)-\left(3x+1\right)^2\)
\(=9x^2-4-9x^2-6x-1\)
\(=-6x-5\)
e) \(\left(x+5\right)\left(x-2\right)-\left(x+2\right)^2\)
\(=x^2-2x+5x-10-x^2-4x-4\)
\(=-x-14\)
f) \(\left(x+3\right)\left(2x-5\right)-2\left(1+x\right)^2\)
\(=2x^2-5x+6x-15-2-4x-2x^2\)
\(=-3x-17\)
g) \(\left(4x-1\right)\left(4x+1\right)-4\left(1-2x\right)^2\)
\(=16x^2-1-4+16x-16x^2\)
\(=16x-5\)
#Học tốt!
â) viết lại biểu thức bên trái = (x2+5x-3)(x2-2x-4)+(14+a)x+b-12
Để là phép chia hết thì số dư =0
Số dư chính là (14+a)x+b-12=0 => a+14=0 và b-12=0 <=>a=-14 và b=12
b) làm tương tự phân tích vế trái thành (x3-2x2+4)(x2+9x+18)+(a+32)x2+(b-36)x
số dư là (a+32)x2+(b-36)x=0 =>a=-32 và b=36
c) Tương tự (x2-1)4x+(a+4)x+b
số dư là (a+4)x+b =2x-3 =>a+4=2 và b=-3 <=>a=-2 và b=-3
a: \(=6x^4-9x^3+3x^2-4x^3+6x^2-2x+10x^2-15x+5\)
\(=6x^4-13x^3+19x^2-17x+5\)
b: \(=6x^4-\dfrac{9}{4}x^3-\dfrac{9}{2}x^2-\dfrac{8}{3}x^3+x^2+2x-\dfrac{20}{3}x^2+\dfrac{5}{2}x+5\)
\(=6x^4-\dfrac{59}{12}x^3-\dfrac{67}{6}x^2+\dfrac{9}{2}x+5\)
c: \(=3x^4-\dfrac{9}{8}x^3-\dfrac{3}{4}x^2+8x^3-3x^2-6x-\dfrac{4}{3}x^2+\dfrac{1}{2}x+1\)
\(=3x^4-\dfrac{55}{8}x^3-\dfrac{25}{12}x^2-\dfrac{11}{2}x+1\)
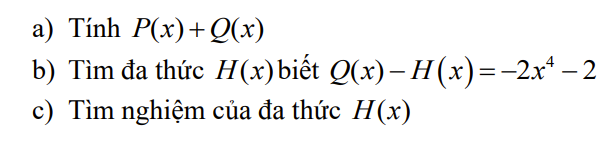
`a)P(x)+Q(x)=3x^4-x^3+4x^2+2x+1-2x^4-x^2+x-2`
`=x^4-x^3+3x^2+3x-1`
`b)Q(x)-H(x)=-2x^4-2`
`=>H(x)=Q(x)-(-2x^4-2)`
`=>H(x)=-2x^4-x^2+x-2+2x^4+2`
`=>H(x)=-x^2+x`
`c)` Cho `H(x)=0`
`=>-x^2+x=0`
`=>-x(x-1)=0`
`@TH1:-x=0=>x=0`
`@TH2:x-1=0=>x=1`
\(a,P\left(x\right)+Q\left(x\right)=x^4-x^3+3x^2+3x-1\)
\(b,H\left(x\right)=Q\left(x\right)+2x^4+2=-2x^4-x^2+x-2+2x^4+2=-x^2+x\)
\(c,H\left(x\right)=-x^2+x=x\left(1-x\right)=0\Leftrightarrow\left[{}\begin{matrix}x=0\\x=1\end{matrix}\right.\)