Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a) ĐKXĐ: \(x\ne3;x\ne\pm2\)
\(C=\frac{2a-a^2}{a+3}\cdot\left(\frac{a-2}{a+2}-\frac{a+2}{a-2}+\frac{4a^2}{4-a^2}\right)\)
\(C=\frac{-a^2+2a}{a+3}\cdot\left(-\frac{4a}{a-2}\right)\)
\(C=-\frac{2a-a^2}{a+3}\cdot\frac{4a}{a-2}\)
\(C=-\frac{\left(2a-a^2\right)\cdot4a}{\left(a+3\right)\left(a-2\right)}\)
\(C=\frac{4a^2}{a+3}\)
b) \(C=\frac{4.4^2}{4+3}=\frac{46}{7}\)
c) \(\frac{4a^2}{a+3}=1\)
<=> 4a2 = a + 3
<=> 4a2 - a - 3 = 0
<=> 4a2 - 3a - 4a - 3 = 0
<=> a(4a + 3) - (4a + 3) = 0
<=> (4a + 3)(a - 1) = 0
<=> 4a + 3 = 0 hoặc a - 1 = 0
<=> a = -3/4 hoặc a = 1

a, Để B xác định
\(\Leftrightarrow\left\{{}\begin{matrix}x-2\ne0\\x+2\ne0\\4-x^2\ne0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x\ne2\\x\ne-2\end{matrix}\right.\)
\(b,B=\dfrac{3}{x-2}+\dfrac{-2}{x+2}-\dfrac{x-14}{4-x^2}\)
\(=\dfrac{3\left(x+2\right)}{\left(x+2\right)\left(x-2\right)}+\dfrac{-2\left(x-2\right)}{\left(x+2\right)\left(x-2\right)}+\dfrac{x-14}{\left(x-2\right)\left(x+2\right)}\)
\(=\dfrac{3x+6-2x+4+x-14}{\left(x+2\right)\left(x-2\right)}\)
\(=\dfrac{2x-4}{\left(x-2\right)\left(x+2\right)}=\dfrac{2\left(x-2\right)}{\left(x-2\right)\left(x+2\right)}=\dfrac{2}{x+2}\)
c, Đẻ B có giá trị nguyên
\(\Leftrightarrow2⋮x+2\Leftrightarrow x+2\inƯ\left(2\right)=\left\{1;-1;2;-2\right\}\)
Ta có bẳng sau:
| \(x+2\) | 1 | -1 | 2 | -2 |
| 2 | -1 | -3 | 0 | -4 |
Vậy \(x\in\left\{-1;-3;0;-4\right\}\) thì B có giá trị nguyên

Lời giải của bạn Nhật Linh đúng rồi, tuy nhiên cần thêm điều kiện để A có nghĩa: \(x\ne\pm2\)

Bài 1 rút gọn bc tự làm :
\(B=\dfrac{3y^3-7y^2+5y-1}{2y^3-y^2-4y+3}\)
\(B=\dfrac{3x^3-3y^2-4y^2+4y+y-1}{2y^3-2y^2+y^2-y+3y-3}\)
\(B=\dfrac{3y^2\left(y-1\right)-4y\left(y-1\right)+\left(y-1\right)}{2y^2\left(y-1\right)+y\left(y-1\right)-3\left(y-1\right)}\)
\(B=\dfrac{\left(3y^2-4y+1\right)\left(y-1\right)}{\left(2y^2+y-3\right)\left(y-1\right)}\)
\(B=\dfrac{3y^2-3y-y+1}{2y^2-2y+3y-3}=\dfrac{3y\left(y-1\right)-\left(y-1\right)}{2y\left(y-1\right)+3\left(y-1\right)}\)
\(B=\dfrac{\left(3y-1\right)\left(y-1\right)}{\left(3y+2\right)\left(y-1\right)}=\dfrac{3y-1}{3y+2}\)
Bài 2 )
a ) \(x+\dfrac{1}{x}=3\)
\(\Leftrightarrow x^2+2x\dfrac{1}{x}+\dfrac{1}{x^2}=9\)
\(\Leftrightarrow x^2+\dfrac{1}{x^2}=1\)
b ) \(\left(x+\dfrac{1}{x}\right)^3=27\)
\(\Leftrightarrow x^3+\dfrac{1}{x^3}+\dfrac{3}{x}+3x=27\)
\(\Leftrightarrow x^3+\dfrac{1}{x^3}+3\left(\dfrac{1}{x}+x\right)=27\)
\(\Leftrightarrow x^3+\dfrac{1}{x^3}=18\)

Trả lời
a,- Rút gọn A như sau:
A= \(\dfrac{4}{x+2}+\dfrac{2}{x-2}-\dfrac{5x-6}{x^2-4}\)
A= \(\dfrac{4}{x+2}+\dfrac{2}{x-2}-\dfrac{5x-6}{\left(x-2\right)\left(x+2\right)}\)
A= \(\text{}\text{}\dfrac{4\left(x-2\right)}{\left(x-2\right)\left(x+2\right)}+\dfrac{2\left(x+2\right)}{\left(x-2\right)\left(x+2\right)}-\dfrac{5x-6}{\left(x-2\right)\left(x+2\right)}\)
A= \(\dfrac{4x-8}{\left(x-2\right)\left(x+2\right)}+\dfrac{2x+4}{\left(x-2\right)\left(x+2\right)}-\dfrac{5x-6}{\left(x-2\right)\left(x+2\right)}\)
A= \(\dfrac{4x-8+2x+4-5x+6}{\left(x-2\right)\left(x+2\right)}\)
A= \(\dfrac{x-2}{\left(x-2\right)\left(x+2\right)}\) A= \(\dfrac{1}{x+2}\) -Thay x = \(\dfrac{7}{3}\)vào biểu thức A ta có: A= \(\dfrac{1}{\dfrac{7}{3}+2}\) A=\(\dfrac{3}{13}\) Vậy khi x= \(\dfrac{7}{3}\)thì A có giá trị bằng \(\dfrac{3}{13}\)

a: ĐKXĐ: x<>2; x<>-2; x<>0
b: \(A=\dfrac{2x+4-4}{\left(x+2\right)^2}:\dfrac{2-x-2}{\left(x+2\right)\left(x-2\right)}\)
\(=\dfrac{2x}{\left(x+2\right)^2}\cdot\dfrac{\left(x+2\right)\left(x-2\right)}{-x}=\dfrac{-2\left(x-2\right)}{x+2}\)
c: Khi x=2 thì A ko xác định
Khi x=3/4 thì \(A=\dfrac{-2\left(\dfrac{3}{4}-2\right)}{\dfrac{3}{4}+2}=\dfrac{10}{11}\)
d: Để A=0 thì x-2=0
=>x=2(loại)
Để A=-2/3 thì \(\dfrac{-2\left(x-2\right)}{x+2}=\dfrac{-2}{3}\)
=>x-2/x+2=1/3
=>3x-6=x+2
=>2x=8
=>x=4

\(Câu\text{ }1:\)
\(\text{ a) }A=\dfrac{4}{x^2+2}+\dfrac{3}{2-x^2}-\dfrac{12}{4-x^4}\\ A=\dfrac{4\left(2-x^2\right)}{\left(x^2+2\right)\left(2-x^2\right)}+\dfrac{3\left(2+x^2\right)}{\left(2-x^2\right)\left(2+x^2\right)}-\dfrac{12}{\left(2+x^2\right)\left(2-x^2\right)}\\ A=\dfrac{4\left(2-x^2\right)+3\left(2+x^2\right)-12}{\left(x^2+2\right)\left(2-x^2\right)}\\ A=\dfrac{8-4x^2+6+3x^2-12}{\left(x^2+2\right)\left(2-x^2\right)}\\ A=\dfrac{-x^2-2}{\left(x^2+2\right)\left(2-x^2\right)}\\ A=\dfrac{-\left(x^2+2\right)}{\left(x^2+2\right)\left(2-x^2\right)}\\ A=\dfrac{-1}{2-x^2}\)
\(\text{b) }Để\text{ }A=-3\\ thì\Rightarrow\dfrac{-1}{2-x^2}=-3\\ \Leftrightarrow2-x^2=3\\ \Leftrightarrow x^2=-1\\ \Leftrightarrow x\text{ }không\text{ }có\text{ }giá\text{ }trị\left(vì\text{ }x^2\ge0\forall x\right)\\ \text{ }Vậy\text{ }để\text{ }A=-3\text{ }thì\text{ }x\text{ }không\text{ }có\text{ }giá\text{ }trị.\)
\(\text{c) }Ta\text{ }có:\text{ }A=\dfrac{-1}{2-x^2}\\ A=\dfrac{1}{x^2-2}\\ x^2\ge0\forall x\\ \Rightarrow x^2-2\ge-2\forall x\\ \Rightarrow A=\dfrac{1}{x^2-2}\le-\dfrac{1}{2}\\ Dấu\text{ }"="\text{ }xảy\text{ }khi:\\ x^2=0\\ \Leftrightarrow x=0\\\text{ }Vậy\text{ }A_{\left(Max\right)}=-\dfrac{1}{2}\text{ }khi\text{ }x=0\)
\(Câu\text{ }2:\)
\(\text{a) }B=\dfrac{1}{x}+\dfrac{1}{x+5}+\dfrac{x-5}{x\left(x+5\right)}\\ B=\dfrac{x+5}{x\left(x+5\right)}+\dfrac{x}{\left(x+5\right)x}+\dfrac{x-5}{x\left(x+5\right)}\\ B=\dfrac{x+5+x+x-5}{x\left(x+5\right)}\\ B=\dfrac{3x}{x\left(x+5\right)}\\ B=\dfrac{3}{x+5}\left(\text{*}\right)\)
\(\text{b) }Ta\text{ }có:\text{ }\left|x-1\right|=6\\ \Leftrightarrow\left[{}\begin{matrix}x-1=6\\x-1=-6\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=7\\x=-5\end{matrix}\right.\\ Ta\text{ }lại\text{ }có:\text{ }B=\dfrac{3}{x+5}\\ \RightarrowĐKCĐ:x+5\ne0\\ \Rightarrow x\ne-5\\ \Rightarrow x=7\text{ }thỏa\text{ }mãn\text{ }với\text{ }điều\text{ }kiện\text{ }của\text{ }biến.\\ x=-5\text{ }không\text{ }thỏa\text{ }mãn\text{ }với\text{ }điều\text{ }kiện\text{ }của\text{ }biến.\\ Thay\text{ }x=7\text{ }vào\text{ }\left(\text{*}\right),ta\text{ }được:\text{ }B=\dfrac{3}{7+5}=\dfrac{3}{12}=\dfrac{1}{4}\\ \text{ }Vậy\text{ }với\text{ }x=7\text{ }thì\text{ }B=\dfrac{1}{4}\\ với\text{ }x=-5\text{ }thì\text{ }B\text{ }không\text{ }có\text{ }giá\text{ }trị.\)
\(\text{c) }Ta\text{ }có:B=\dfrac{3}{x+5}\\ \RightarrowĐể\text{ }B\in Z\\ thì\Rightarrow3⋮x+5\\ \Rightarrow x+5\inƯ_{\left(3\right)}\\ Mà\text{ }Ư_{\left(3\right)}=\left\{\pm1;\pm3\right\}\\ Ta\text{ }lập\text{ }bảng\text{ }xét\text{ }giá\text{ }trị:\)
| \(x+5\) | \(-3\) | \(-1\) | \(1\) | \(3\) |
| \(x\) | \(-8\) | \(-6\) | \(-4\) | \(-2\) |
\(\Rightarrow x\in\left\{-8;-6;-4;-2\right\}\\ Vậy\text{ }để\text{ }B\in Z\\ thì x\in\left\{-8;-6;-4;-2\right\}\)


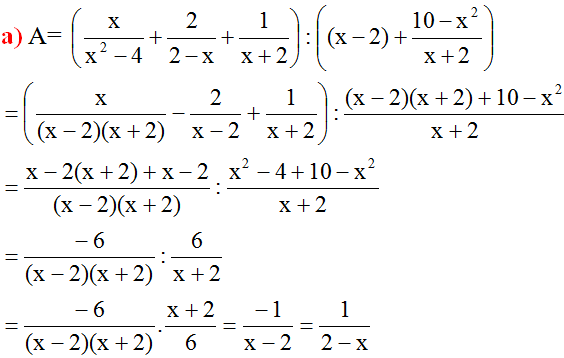

Lời giải:
a. ĐKXĐ: $a\neq \pm 2$
\(M=\frac{(2+a)^2}{(2-a)(2+a)}+\frac{4a^2}{(2-a)(2+a)}-\frac{(2-a)^2}{(2+a)(2-a)}\)
\(=\frac{(2+a)^2+4a^2-(2-a)^2}{(2-a)(2+a)}=\frac{4a(a+2)}{(2-a)(2+a)}=\frac{4a}{2-a}\)
b.
$|a+1|=3\Rightarrow a+1=\pm 3\Rightarrow a=-2$ hoặc $a=-4$
Vì $a\neq \pm 2$ nên $a=-4$
Khi đó: $M=\frac{4a}{2-a}=\frac{4(-4)}{2-(-4)}=\frac{-8}{3}$
c.
Trước tiên cần tìm $a$ để $M$ nguyên đã.
$M=\frac{4a}{2-a}=\frac{8-4(2-a)}{2-a}=\frac{8}{2-a}-4$ nguyên khi $\frac{8}{2-a}$ nguyên
$\Rightarrow 2-a\in\left\{\pm 1; \pm 2; \pm 4; \pm 8\right\}$
$\Rightarrow a\in\left\{1; 3; 0; 4; -2; 6; 10; -6\right\}$.
Thử lại thấy $a\in\left\{1; 3; 0; 4\right\}$ thỏa mãn $M$ là số nguyên chia hết cho $4$