Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Chọn D.
Để f(x) ≤ 0 thì (x + 5)(3 - x) < 0
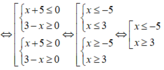
Vậy x ∈ (- ∞ ;-5] ∪ [3;+ ∞ ).

\(f\left(x\right)=\frac{2-x}{x+1}+2=\frac{x+4}{x+1}< 0\)
\(\Leftrightarrow-4< x< -1\)

1/ \(f\left(x\right)\ge0\Leftrightarrow2x-4\ge0\Leftrightarrow x\ge2\)
2/ \(f\left(x\right)\le0\Leftrightarrow\left(x+5\right)\left(3-x\right)\le0\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x\ge-5\\x\ge3\end{matrix}\right.\\\left\{{}\begin{matrix}x\le-5\\x\le3\end{matrix}\right.\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x\ge3\\x\le-5\end{matrix}\right.\)
6/ ĐKXĐ: \(x\ne2\)
\(f\left(x\right)=\frac{1}{3x-6}\le0\Leftrightarrow3x-6< 0\Leftrightarrow x< 2\)

\(f\left(x\right)=\frac{4\left(x-3\right)}{x\left(x-4\right)}\le0\) \(\Rightarrow\left[{}\begin{matrix}x< 0\\3\le x< 4\end{matrix}\right.\)
Hay: \(\left(-\infty;0\right)\cup[3;4)\)