K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
H
2 tháng 1 2023
\(a,đk\left(B\right):x\ne\pm3\\ B=\dfrac{3}{x-3}-\dfrac{6x}{9-x^2}+\dfrac{x}{x+3}\\ =\dfrac{3}{x-3}+\dfrac{6x}{x^2-9}+\dfrac{x}{x+3}\\ =\dfrac{3\left(x+3\right)+6x+x\left(x-3\right)}{x^2-9}\\ =\dfrac{3x+9+6x+x^2-3x}{x^2-9}\\ =\dfrac{x^2+6x+9}{x^2-9}\\ =\dfrac{\left(x+3\right)^2}{x^2-9}\\ =\dfrac{x+3}{x-3}\)
\(b,P=A.B\\ =\dfrac{x+1}{x+3}\times\dfrac{x+3}{x-3}\\ =\dfrac{x+1}{x-3}\)
\(c,\) Để P nguyên
\(\dfrac{x+1}{x-3}=1+\dfrac{4}{x-3}\)
=> \(x-3\inƯ\left(4\right)\)
\(Ư\left(4\right)=\left\{-1;1;2;-2;4;-4\right\}\)
\(=>x=\left\{2;4;5;1;7;-1\right\}\)
6 tháng 1 2022
a: Thay x=-4 vào B, ta được:
\(B=\dfrac{-4+3}{-4}=\dfrac{-1}{-4}=\dfrac{1}{4}\)
b: \(P=A\cdot B=\dfrac{x^2-3x+2x-9+3x+9}{\left(x-3\right)\left(x+3\right)}\cdot\dfrac{x+3}{x}\)
\(=\dfrac{x^2+2x}{\left(x-3\right)}\cdot\dfrac{1}{x}=\dfrac{x+2}{x-3}\)
c: Để P nguyên thì \(x-3\in\left\{1;-1;5;-5\right\}\)
hay \(x\in\left\{4;2;8;-2\right\}\)
YN
21 tháng 12 2021
Answer:
a) \(\frac{5x}{2x+2}+1=\frac{6}{x+1}\)
\(\Rightarrow\frac{5x}{2\left(x+1\right)}+\frac{2\left(x+1\right)}{2\left(x+1\right)}=\frac{12}{2\left(x+1\right)}\)
\(\Rightarrow5x+2x+2-12=0\)
\(\Rightarrow7x-10=0\)
\(\Rightarrow x=\frac{10}{7}\)
b) \(\frac{x^2-6}{x}=x+\frac{3}{2}\left(ĐK:x\ne0\right)\)
\(\Rightarrow x^2-6=x^2+\frac{3}{2}x\)
\(\Rightarrow\frac{3}{2}x=-6\)
\(\Rightarrow x=-4\)
c) \(\frac{3x-2}{4}\ge\frac{3x+3}{6}\)
\(\Rightarrow\frac{3\left(3x-2\right)-2\left(3x+3\right)}{12}\ge0\)
\(\Rightarrow9x-6-6x-6\ge0\)
\(\Rightarrow3x-12\ge0\)
\(\Rightarrow x\ge4\)
d) \(\left(x+1\right)^2< \left(x-1\right)^2\)
\(\Rightarrow x^2+2x+1< x^2-2x+1\)
\(\Rightarrow4x< 0\)
\(\Rightarrow x< 0\)
e) \(\frac{2x-3}{35}+\frac{x\left(x-2\right)}{7}\le\frac{x^2}{7}-\frac{2x-3}{5}\)
\(\Rightarrow\frac{2x-3+5\left(x^2-2x\right)}{35}\le\frac{5x^2-7\left(2x-3\right)}{35}\)
\(\Rightarrow2x-3+5x^2-10x\le5x^2-14x+21\)
\(\Rightarrow6x\le24\)
\(\Rightarrow x\le4\)
f) \(\frac{3x-2}{4}\le\frac{3x+3}{6}\)
\(\Rightarrow\frac{3\left(3x-2\right)-2\left(3x+3\right)}{12}\le0\)
\(\Rightarrow9x-6-6x-6\le0\)
\(\Rightarrow3x\le12\)
\(\Rightarrow x\le4\)
2 tháng 11 2018
Bài 1:
a) \(A=5\left(x-3\right)\left(x+3\right)+\left(2x+3\right)^2\)
\(A=5\left(x^2-3^2\right)+\left(4x^2+12x+9\right)\)
\(A=5x^2-45+4x^2+12x+9\)
\(A=9x^2+12x-36\)
b) Thay x = 1/3 vào A ta có :
\(A=9\cdot\frac{1}{9}+\frac{12}{3}-36\)
\(A=1+4-36\)
\(A=-31\)
NT
a) cho x+y=1. Tính giá trị biểu thức x^3+ y^3+ 3xy
b) cho x-y=1. Tính giá trị biểu thức x^3- y^3- 3xy
2
BT
30 tháng 6 2018
x^3+ y^3+ 3xy
=(x+y)(x^2 -xy + y^2 ) + 3xy
=x^2 -xy + y^2 + 3xy
=x^2 + 2xy + y^2
=(x+y)^2 =1
=> x^3+ y^3+ 3xy=1
HQ
Hà Quang Minh
Giáo viên
21 tháng 7 2023
a, \(A=3x^3\left(x^5-y^5\right)+y^5\left(3x^3-y^3\right)\\ =3x^8-3x^3y^5+3x^3y^5-y^8\\ =3x^8-y^8\)
b, Có \(y^4=x^4\sqrt{3}\Rightarrow y^8=3x^8\)
Thay vào A, ta được: \(A=3x^8-3x^8=0.\)
KK
2 tháng 4 2018
a) do gt của bt 3-4x lớn hơn gt của bt 2(3-x)
=> 3-4x>2(3-x)
⇔ 3-4x>6-2x
⇔ 3-6>4x-2x
⇔-3>2x
⇔ 2x<-3
⇔ x< \(\dfrac{-3}{2}\)
vậy x< \(\dfrac{-3}{2}\)
b vì gt của bt \(\dfrac{3-1}{2}\) ko lớn hơn gt của bt x+2
=> \(\dfrac{3-1}{2}\ge x+2\)
⇔ 1>x+2
⇔ -1>x
vậy x<-1
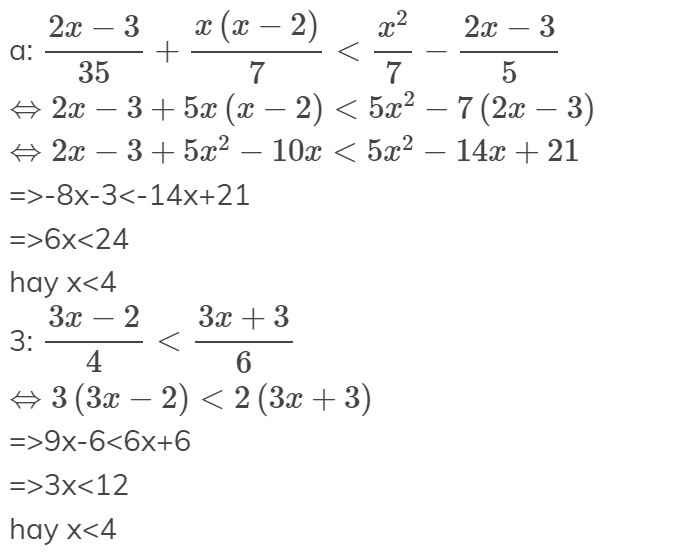
3/3-x=0
3/3-3x/3=0
3-3x=0
-3x=-3
x=-3/-3
x=1
tìm x nguyên để bth nguyên hả bạn ?
\(3-x\inƯ\left(3\right)=\left\{\pm1;\pm3\right\}\)