Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(P=\frac{\sqrt{a}}{\sqrt{a}-1}-\frac{4-6\sqrt{a}}{1-a}-\frac{-3}{\sqrt{a}+1}\)
ĐK : \(\hept{\begin{cases}a\ge0\\a\ne1\end{cases}}\)
a) \(P=\frac{\sqrt{a}}{\sqrt{a}-1}+\frac{4-6\sqrt{a}}{a-1}+\frac{3}{\sqrt{a}+1}\)
\(=\frac{\sqrt{a}}{\sqrt{a}-1}+\frac{4-6\sqrt{a}}{\left(\sqrt{a}-1\right)\left(\sqrt{a}+1\right)}+\frac{3}{\sqrt{a}+1}\)
\(=\frac{\sqrt{a}\left(\sqrt{a}+1\right)}{\left(\sqrt{a}-1\right)\left(\sqrt{a}+1\right)}+\frac{4-6\sqrt{a}}{\left(\sqrt{a}-1\right)\left(\sqrt{a}+1\right)}+\frac{3\left(\sqrt{a}-1\right)}{\left(\sqrt{a}-1\right)\left(\sqrt{a}+1\right)}\)
\(=\frac{a+\sqrt{a}}{\left(\sqrt{a}-1\right)\left(\sqrt{a}+1\right)}+\frac{4-6\sqrt{a}}{\left(\sqrt{a}-1\right)\left(\sqrt{a}+1\right)}+\frac{3\sqrt{a}-3}{\left(\sqrt{a}-1\right)\left(\sqrt{a}+1\right)}\)
\(=\frac{a+\sqrt{a}+4-6\sqrt{a}+3\sqrt{a}-3}{\left(\sqrt{a}-1\right)\left(\sqrt{a}+1\right)}\)
\(=\frac{a-2\sqrt{a}+1}{\left(\sqrt{a}-1\right)\left(\sqrt{a}+1\right)}=\frac{\left(\sqrt{a}-1\right)^2}{\left(\sqrt{a}-1\right)\left(\sqrt{a}+1\right)}=\frac{\sqrt{a}-1}{\sqrt{a}+1}\)
Với \(a=4-2\sqrt{3}\)( tmđk \(\hept{\begin{cases}a\ge0\\a\ne1\end{cases}}\))
\(P=\frac{\sqrt{4-2\sqrt{3}}-1}{\sqrt{4-2\sqrt{3}}+1}\)
\(=\frac{\sqrt{3-2\sqrt{3}+1}-1}{\sqrt{3-2\sqrt{3}+1}+1}\)
\(=\frac{\sqrt{\left(\sqrt{3}\right)^2-2\sqrt{3}+1^2}-1}{\sqrt{\left(\sqrt{3}\right)^2-2\sqrt{3}+1^2}+1}\)
\(=\frac{\sqrt{\left(\sqrt{3}-1\right)^2}-1}{\sqrt{\left(\sqrt{3}-1\right)^2}+1}\)
\(=\frac{\left|\sqrt{3}-1\right|-1}{\left|\sqrt{3}-1\right|+1}\)
\(=\frac{\sqrt{3}-1-1}{\sqrt{3}-1+1}=\frac{\sqrt{3}-2}{\sqrt{3}}\)
b) \(P=\frac{\sqrt{a}-1}{\sqrt{a}+1}=\frac{\sqrt{a}+1-2}{\sqrt{a}+1}=1-\frac{2}{\sqrt{a}+1}\)( ĐK \(\hept{\begin{cases}a\ge0\\a\ne1\end{cases}}\))
Để P đạt giá trị nguyên => \(\frac{2}{\sqrt{a}+1}\)nguyên
=> \(2⋮\sqrt{a}+1\)
=> \(\sqrt{a}+1\inƯ\left(2\right)=\left\{\pm1;\pm2\right\}\)
=> \(\sqrt{a}\in\left\{0;1\right\}\)< đã loại hai trường hợp âm >
=> \(a\in\left\{0\right\}\)< loại trường hợp a = 1 >
Vậy với a = 0 thì P có giá trị nguyên

a) \(\frac{b-16}{4-\sqrt{b}}\left(b\ge0,b\ne16\right)\)
\(=\frac{\left(\sqrt{b}-4\right)\left(\sqrt{b}+4\right)}{4-\sqrt{b}}\)
\(=-\sqrt{b}-4\)
b) \(\frac{a-4\sqrt{a}+4}{a-4}\left(a\ge0;a\ne4\right)\)
\(=\frac{a-2.\sqrt{a}.2+4}{\left(\sqrt{a}-2\right)\left(\sqrt{a}+2\right)}\)
\(=\frac{\left(\sqrt{a}-2\right)^2}{\left(\sqrt{a}+2\right)\left(\sqrt{a}-2\right)}=\frac{\sqrt{a}-2}{\sqrt{a}+2}\)
c) \(2x+\sqrt{1+4x^2-4x}\) với \(x\le\frac{1}{2}\)
\(=2x+\sqrt{\left(1-2x\right)^2}\)
\(=2x+\left|1-2x\right|=2x+1-2x=1\)
d) \(\frac{4a-4b}{\sqrt{a}-\sqrt{b}}\left(a,b\ge0;a\ne b\right)\)
\(=\frac{4\left(a-b\right)}{\sqrt{a}-\sqrt{b}}=\frac{4\left(\sqrt{a}-\sqrt{b}\right)\left(\sqrt{a}+\sqrt{b}\right)}{\sqrt{a}-\sqrt{b}}\)
\(=4\left(\sqrt{a}+\sqrt{b}\right)\)

đk : \(a\ge0;b\ge0;a\ne b\)
a) \(\dfrac{\sqrt{a}+\sqrt{b}}{\sqrt{a}-\sqrt{b}}+\dfrac{\sqrt{a}-\sqrt{b}}{\sqrt{a}+\sqrt{b}}\) = \(\dfrac{\left(\sqrt{a}+\sqrt{b}\right)^2+\left(\sqrt{a}-\sqrt{b}\right)^2}{\left(\sqrt{a}+\sqrt{b}\right)\left(\sqrt{a}-\sqrt{b}\right)}\)
= \(\dfrac{a+2\sqrt{ab}+b+a-2\sqrt{ab}+b}{a-b}\) = \(\dfrac{2\left(a+b\right)}{a-b}\)
b) đk : \(a\ge0;b\ge0;a\ne b\)
\(\dfrac{a-b}{\sqrt{a}-\sqrt{b}}-\dfrac{\sqrt{a^3}-\sqrt{b^3}}{a-b}\)
= \(\dfrac{\left(\sqrt{a}+\sqrt{b}\right)\left(\sqrt{a}-\sqrt{b}\right)}{\sqrt{a}-\sqrt{b}}-\dfrac{\left(\sqrt{a}-\sqrt{b}\right)\left(a+\sqrt{ab}+b\right)}{\left(\sqrt{a}+\sqrt{b}\right)\left(\sqrt{a}-\sqrt{b}\right)}\)
= \(\dfrac{\sqrt{a}+\sqrt{b}}{1}-\dfrac{a+\sqrt{ab}+b}{\sqrt{a}+\sqrt{b}}\) = \(\dfrac{\left(\sqrt{a}+\sqrt{b}\right)^2-\left(a+\sqrt{ab}+b\right)}{\sqrt{a}+\sqrt{b}}\)
= \(\dfrac{a+2\sqrt{ab}+b-a-\sqrt{ab}-b}{\sqrt{a}+\sqrt{b}}\) = \(\dfrac{\sqrt{ab}}{\sqrt{a}+\sqrt{b}}=\dfrac{\sqrt{ab}\left(\sqrt{a}-\sqrt{b}\right)}{a+b}\)

a, \(\sqrt{75}+\sqrt{48}-\sqrt{300}\)
\(=5\sqrt{3}+4\sqrt{3}-10\sqrt{3}\)
\(=-\sqrt{3}\)
b, \(\sqrt{81a}-\sqrt{36a}+\sqrt{144a}\)
\(=9\sqrt{a}-6\sqrt{a}+12\sqrt{a}\)
\(=15\sqrt{a}\)
c, \(\dfrac{4}{\sqrt{5}-2}-\dfrac{4}{\sqrt{5}+2}\)
\(=\dfrac{4\sqrt{5}+8-4\sqrt{5}+8}{\left(\sqrt{5}-2\right)\left(\sqrt{5}+2\right)}\)
\(=\dfrac{16}{5-4}=16\)
d, \(\dfrac{a\sqrt{b}-b\sqrt{a}}{\sqrt{a}-\sqrt{b}}=\dfrac{\sqrt{ab}\left(\sqrt{a}-\sqrt{b}\right)}{\sqrt{a}-\sqrt{b}}=\sqrt{ab}\)
Nguyễn Huy Tú anh sinh năm 2004 là lên lớp 8 mà sao lại tl được bài lớp 9

Ta có : \(\left(\dfrac{a\sqrt{a}+b\sqrt{b}}{\sqrt{a}+\sqrt{b}}+\sqrt{ab}\right)\)\(\left(\dfrac{\sqrt{a}+\sqrt{b}}{a-b}\right)^2\)=1
⇌ \(\left(\dfrac{\sqrt{a}^3+\sqrt{b}^3}{\sqrt{a}+\sqrt{b}}+\sqrt{ab}\right)\)\(\left(\dfrac{\sqrt{a}+\sqrt{b}}{\left(\sqrt{a}+\sqrt{b}\right)\left(\sqrt{a}-\sqrt{b}\right)}\right)^2\)=1
⇌ \(\left(\dfrac{\left(\sqrt{a}+\sqrt{b}\right)\left(a-\sqrt{ab}+b\right)}{\sqrt{a}+\sqrt{b}}+\sqrt{ab}\right)\)\(\dfrac{1}{\left(\sqrt{a}-\sqrt{b}\right)^2}\)=1
⇌ \(\left(a+b\right)\)\(\dfrac{1}{\left(\sqrt{a}-\sqrt{b}\right)^2}\)=1
⇌ \(\dfrac{a+b}{\left(\sqrt{a}-\sqrt{b}\right)^2}-1=0\)
⇌ \(\dfrac{a+b-a+\sqrt{ab}-b}{\left(\sqrt{a}-\sqrt{b}\right)^2}=0\)
⇌ \(\sqrt{ab}=0\)
⇌\(\left[{}\begin{matrix}a=0\\b=0\end{matrix}\right.\)(thỏa mãn điều kiện)
Vậy a=0;b=0

1/ Rút gọn: \(a)3\sqrt{2a}-\sqrt{18a^3}+4\sqrt{\dfrac{a}{2}}-\dfrac{1}{4}\sqrt{128a}\left(a\ge0\right)=3\sqrt{2a}-3a\sqrt{2a}+2\sqrt{2a}-2\sqrt{2a}=3\sqrt{2a}\left(1-a\right)\)b)\(\dfrac{\sqrt{2}-1}{\sqrt{2}+2}-\dfrac{2}{2+\sqrt{2}}+\dfrac{\sqrt{2}+1}{\sqrt{2}}=\dfrac{\sqrt{2}-1-2}{\sqrt{2}+2}+\dfrac{\sqrt{2}+1}{\sqrt{2}}=\dfrac{\sqrt{2}-3}{\sqrt{2}+2}+\dfrac{\sqrt{2}+1}{\sqrt{2}}=\dfrac{\sqrt{2}-3+2+1+2\sqrt{2}}{\sqrt{2}\left(1+\sqrt{2}\right)}=\dfrac{3\sqrt{2}}{\sqrt{2}\left(1+\sqrt{2}\right)}=\dfrac{3}{1+\sqrt{2}}\)c)\(\dfrac{2+\sqrt{5}}{\sqrt{2}+\sqrt{3+\sqrt{5}}}+\dfrac{2-\sqrt{5}}{\sqrt{2}-\sqrt{3-\sqrt{5}}}=\dfrac{\sqrt{2}\left(2+\sqrt{5}\right)}{\left(\sqrt{2}+\sqrt{3+\sqrt{5}}\right)\sqrt{2}}+\dfrac{\sqrt{2}\left(2-\sqrt{5}\right)}{\sqrt{2}\left(\sqrt{2}-\sqrt{3-\sqrt{5}}\right)}=\dfrac{2\sqrt{2}+\sqrt{10}}{2+\sqrt{6+2\sqrt{5}}}+\dfrac{2\sqrt{2}-\sqrt{10}}{2-\sqrt{6-2\sqrt{5}}}=\dfrac{2\sqrt{2}+\sqrt{10}}{2+\sqrt{\left(\sqrt{5}+1\right)^2}}+\dfrac{2\sqrt{2}-\sqrt{10}}{2-\sqrt{\left(\sqrt{5}-1\right)^2}}=\dfrac{\sqrt{2}\left(2+\sqrt{5}\right)}{2+\sqrt{5}+1}+\dfrac{\sqrt{2}\left(2-\sqrt{5}\right)}{2-\sqrt{5}+1}=\dfrac{\sqrt{2}\left(2+\sqrt{5}\right)}{3+\sqrt{5}}+\dfrac{\sqrt{2}\left(2-\sqrt{5}\right)}{3-\sqrt{5}}=\dfrac{\sqrt{2}\left(2+\sqrt{5}\right)\left(3-\sqrt{5}\right)+\sqrt{2}\left(2-\sqrt{5}\right)\left(3+\sqrt{5}\right)}{\left(3+\sqrt{5}\right)\left(3-\sqrt{5}\right)}=\dfrac{\sqrt{2}\left(6-2\sqrt{5}+3\sqrt{5}-5+6+2\sqrt{5}-3\sqrt{5}-5\right)}{9-5}=\dfrac{2\sqrt{2}}{4}=\dfrac{1}{\sqrt{2}}\)
Làm nốt nè :3
\(2.a.P=\left(\dfrac{1}{x-\sqrt{x}}+\dfrac{1}{\sqrt{x}-1}\right):\dfrac{\sqrt{x}}{x-2\sqrt{x}+1}=\dfrac{\sqrt{x}+1}{\sqrt{x}\left(\sqrt{x}-1\right)}.\dfrac{\left(\sqrt{x}-1\right)^2}{\sqrt{x}}=\dfrac{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}{x}=\dfrac{x-1}{x}\left(x>0;x\ne1\right)\)\(b.P>\dfrac{1}{2}\Leftrightarrow\dfrac{x-1}{x}-\dfrac{1}{2}>0\)
\(\Leftrightarrow\dfrac{x-2}{2x}>0\)
\(\Leftrightarrow x-2>0\left(do:x>0\right)\)
\(\Leftrightarrow x>2\)
\(3.a.A=\left(\dfrac{\sqrt{a}}{\sqrt{a}-1}-\dfrac{\sqrt{a}}{a-\sqrt{a}}\right):\dfrac{\sqrt{a}+1}{a-1}=\dfrac{\sqrt{a}-1}{\sqrt{a}-1}.\dfrac{\left(\sqrt{a}-1\right)\left(\sqrt{a}+1\right)}{\sqrt{a}+1}=\sqrt{a}-1\left(a>0;a\ne1\right)\)
\(b.Để:A< 0\Leftrightarrow\sqrt{a}-1< 0\Leftrightarrow a< 1\)
Kết hợp với DKXĐ : \(0< a< 1\)

Đặt x=\(\sqrt{a}\), y=\(\sqrt{b}\) (x,y>0) .Khi đó biểu thức đã cho có dạng:
\(\frac{x^3-y^3}{x-y}=\frac{\left(x-y\right)\left(x^2+xy+y^2\right)}{x-y}=x^2+xy+y^2=a+\sqrt{ab}+b\)

a: \(=2\sqrt{2}+30\sqrt{2}-3\sqrt{2}+6\sqrt{2}=26\sqrt{2}\)
b: \(=\dfrac{1}{2}\cdot4\sqrt{3}-2\cdot5\sqrt{3}+\sqrt{3}+\dfrac{5}{2}\sqrt{3}=-\dfrac{9}{2}\sqrt{3}\)
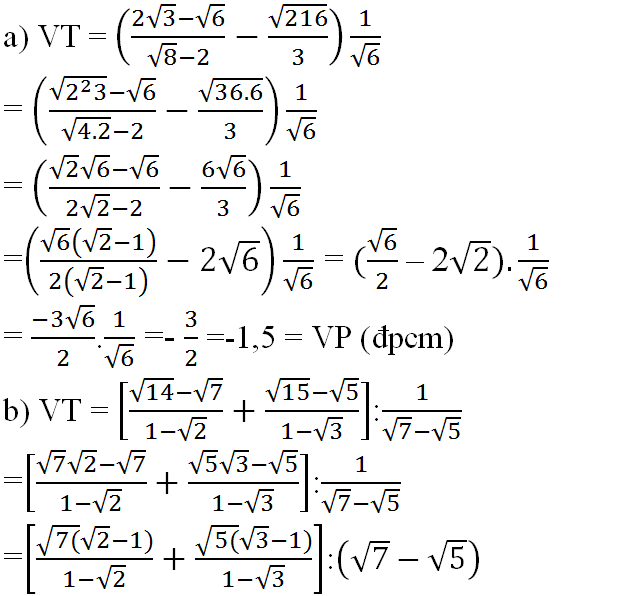
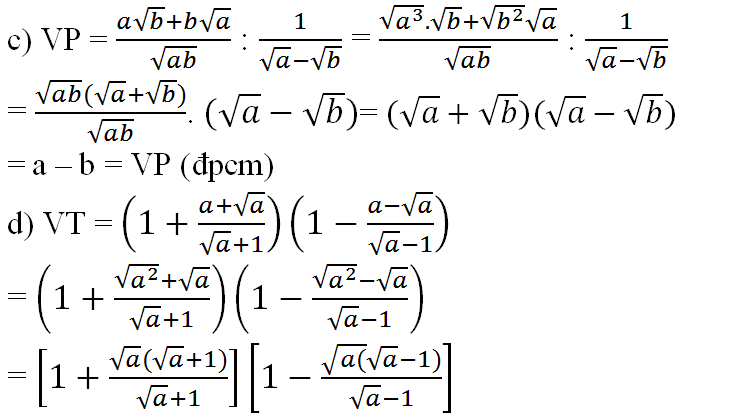
Ta có: \(a=2007-2\sqrt{2006}=\left(\sqrt{2007}-1\right)^2\)
\(\Rightarrow\sqrt{a}=\left|\sqrt{2017}-1\right|=\sqrt{2017}-1\)
Thay \(\sqrt{a}=\sqrt{2017}-1\) vào \(A=\dfrac{a+\sqrt{a}+1}{\sqrt{a}-1}\) ta có:
\(A=\dfrac{2018-2\sqrt{2017}+1+\sqrt{2017}-1}{\sqrt{2017}-1-1}=\dfrac{2018-\sqrt{2017}}{\sqrt{2017}-2}\)
cày nhìn đã mắt ghe:<