S7
3
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
17 tháng 9 2023
\(P(x).A(x) = Q(x) \Rightarrow A(x) = Q(x):P(x)\)
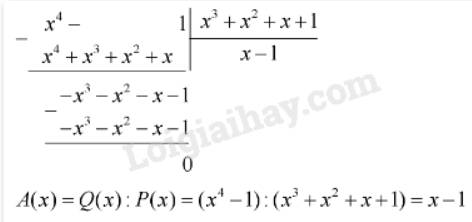
Vậy \(A(x) = x - 1\).
9 tháng 4 2016
1/a, f(x) - g(x) + h(x) = x3 - 2x2 + 3x +1 - x3 - x + 1 +2x2 - 1
=(x3 - x3) + (-2x2 + 2x2) + (3x - x) + (1 + 1 - 1)
=2x + 1
b, f(x) - g(x) + h(x) = 0
<=> 2x + 1 = 0
<=> 2x = -1
<=> x = -1/2
Vậy x = -1/2 là nghiệm của đa thức f(x) - g(x) + h(x)
2/ a, 5x + 3(3x + 7)-35 = 0
<=> 5x + 9x + 21 - 35 = 0
<=> 14x - 14 = 0
<=> 14(x - 1) = 0
<=> x-1 = 0
<=> x = 1
Vậy 1 là nghiệm của đa thức 5x + 3(3x + 7) -35
b, x2 + 8x - (x2 + 7x +8) -9 =0
<=> x2 + 8x - x2 - 7x - 8 - 9 =0
<=> (x2 - x2) + (8x - 7x) + (-8 -9)
<=> x - 17 = 0
<=> x =17
Vậy 17 là nghiệm của đa thức x2 + 8x -(x2 + 7x +8) -9
3/ f(x) = g (x) <=> x3 +4x2 - 3x + 2 = x2(x + 4) + x -5
<=> x3 +4x2 - 3x + 2 = x3 + 4x2 + x - 5
<=> -3x + 2 = x - 5
<=> -3x = x - 5 - 2
<=> -3x = x - 7
<=>2x = 7
<=> x = 7/2
Vậy f(x) = g(x) <=> x = 7/2
4/ có k(-2) = m(-2)2 - 2(-2) +4 = 0
=> 4m + 4 + 4 = 0
=> 4m + 8 = 0
=> 4m = -8
=> m = -2
LD
1
T
11 tháng 9 2018
a)\(\frac{x+2}{x+5}< \frac{x+1}{x+4}\)
\(\Leftrightarrow\frac{x+5-3}{x+5}< \frac{x+4-3}{x+4}\)
\(\Leftrightarrow1-\frac{3}{x+5}< 1-\frac{3}{x+4}\)
\(\Leftrightarrow\frac{3}{x+5}>\frac{3}{x+4}\)
\(\Leftrightarrow x+5< x+4\)
Vì \(x+5\)luôn lớn hơn x+4 với mọi x
nên không có giá trị x thỏa mãn
b) \(\frac{x-1}{x-2}< \frac{x+4}{x+3}\)
\(\Leftrightarrow\frac{x-2+1}{x-2}< \frac{x+3+1}{x+3}\)
\(\Leftrightarrow1+\frac{1}{x-2}< 1+\frac{1}{x+3}\)
\(\Leftrightarrow\frac{1}{x-2}< \frac{1}{x+3}\)
\(\Leftrightarrow x-2>x+3\)
Vì \(x+3>x-2\)với mọi x
nên không có giá trị x thỏa mãn
NH
1
7 tháng 4 2023
a: Q(x)=3x^4+x^3+2x^2+x+1-2x^4+x^2-x+2
=x^4+x^2+3x^2+3
b: H(x)=2x^4-x^2+x-2-x^4+x^3-x^2+2
=x^4+x^3-2x^2+x
c: R(x)=2x^3+x^2+1+2x^4-x^2+x-2
=2x^4+2x^3+x-1
Ta có: A=(x-4)-3(x+1)
=x-4-3x-3
=-2x-7=4
=>-2x=11 => x=-11/2
Vậy x=-11/2
\(A=\left(x-4\right)-3.\left(x+1\right)\)
\(\Rightarrow A=x-4-3x+3\)
\(\Rightarrow A=-2x-1\)
Mà A = 4
=> 4 = -2x - 1
=> 2x = -5
=> x = \(-\frac{5}{2}\)