Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a: Để hai đường song song thì
\(\left\{{}\begin{matrix}2m^2-m=1\\m^2+m< >2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\left(m-1\right)\left(2m+1\right)=0\\\left(m+2\right)\left(m-1\right)< >0\end{matrix}\right.\Leftrightarrow m=-\dfrac{1}{2}\)
b: Thay x=2 vào (d1), ta đc:
\(y=2+2=4\)
Vì (d3) vuông góc với (d1) nên (d3): y=-x+b
Thay x=2 và y=4 vào (d3), ta được:
b-2=4
=>b=6

\(\Delta=9-4m\ge0\Rightarrow m\le\frac{9}{4}\)
Theo Viet: \(\left\{{}\begin{matrix}x_1+x_2=-3\\x_1x_2=m\end{matrix}\right.\)
a/ \(\left\{{}\begin{matrix}x_1-x_2=6\\x_1+x_2=-3\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x_1=\frac{3}{2}\\x_2=-\frac{9}{2}\end{matrix}\right.\)
\(x_1x_2=m\Rightarrow m=\frac{3}{2}.\left(-\frac{9}{2}\right)=-\frac{27}{4}\)
b/ \(\left\{{}\begin{matrix}3x_1+2x_2=20\\x_1+x_2=-3\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x_1=26\\x_2=-29\end{matrix}\right.\)
\(\Rightarrow m=x_1x_2=-29.26=-754\)
c/ \(\left\{{}\begin{matrix}\left(x_1-x_2\right)\left(x_1+x_2\right)=34\\x_1+x_2=-3\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x_1-x_2=-\frac{34}{3}\\x_1+x_2=-3\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x_1=-\frac{43}{6}\\x_2=\frac{25}{6}\end{matrix}\right.\) \(\Rightarrow m=-\frac{1075}{36}\)
d/ \(\left\{{}\begin{matrix}x_1=2x_2\\x_1+x_2=-3\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x_2=-1\\x_1=-2\end{matrix}\right.\) \(\Rightarrow m=2\)
e/ Giống câu c, bạn tự giải

a: Để hai đường song song thì 3m^2+1=4m và m^2-9<>-m-5
=>(m-1)(3m-1)=0 và m^2+m-4<>0
=>m=1 hoặc m=1/3
b: Để hai đường cắt nhau thì 3m^2+1<>4m
=>m<>1 và m<>1/3
Khi m=2 thì (d1): \(y=8x-7\)
(d2): y=13x-5
Toa độ giao điểm là:
8x-7=13x-5 và y=8x-7
=>-5x=-5+7=2 và y=8x-7
=>x=-2/5 và y=-16/5-7=-16/5-35/5=-51/5

Lời giải:
Để pt có hai nghiệm phân biệt thì \(\Delta'=(m-1)^2-(m^2-3)>0\)
\(\Leftrightarrow 4-2m>0\Leftrightarrow m< 2\)
Khi đó áp dụng định lý Viete về pt bậc 2: \(\left\{\begin{matrix} x_1+x_2=2(m-1)\\ x_1x_2=m^2-3\end{matrix}\right.(*)\)
a) \(x_1-x_2=2\Rightarrow (x_1-x_2)^2=4\)
\(\Leftrightarrow x_1^2+x_2^2-2x_1x_2=4\Leftrightarrow x_1^2+x_2^2+2x_1x_2-4x_1x_2=4\)
\(\Leftrightarrow (x_1+x_2)^2-4x_1x_2=4\)
\(\Leftrightarrow 4(m-1)^2-4(m^2-3)=4\)
\(\Leftrightarrow 8m=12\Leftrightarrow m=\frac{3}{2}\) (thỏa mãn)
b) \(x_1x_2-x_1-x_2=11\)
\(\Leftrightarrow x_1x_2-(x_1+x_2)=11\)
\(\Leftrightarrow m^2-3-2(m-1)=11\)
\(\Leftrightarrow m^2-2m-12=0\Leftrightarrow \left[\begin{matrix} m=1+\sqrt{13}\\ m=1-\sqrt{13}\end{matrix}\right.\)
Vì \(m<2\Rightarrow m=1-\sqrt{13}\)
c)Ta có: \(\left\{\begin{matrix} x_1+x_2=2(m-1)\\ x_1x_2=m^2-3\end{matrix}\right.\)
\(\Rightarrow \left\{\begin{matrix} x_1+x_2+2=2m\\ x_1x_2+3=m^2\end{matrix}\right.\)
Suy ra \( (x_1+x_2+2)^2=4(x_1x_2+3)(=4m^2)\)
\(\Leftrightarrow x_1^2+x_2^2+4+2x_1x_2+4(x_1+x_2)=4x_1x_2+12\)
\(\Leftrightarrow x_1^2+x_2^2-2x_1x_2+4(x_1+x_2)-8=0\)
Đây chính là biểu thức (không phụ thuộc m) cần tìm.

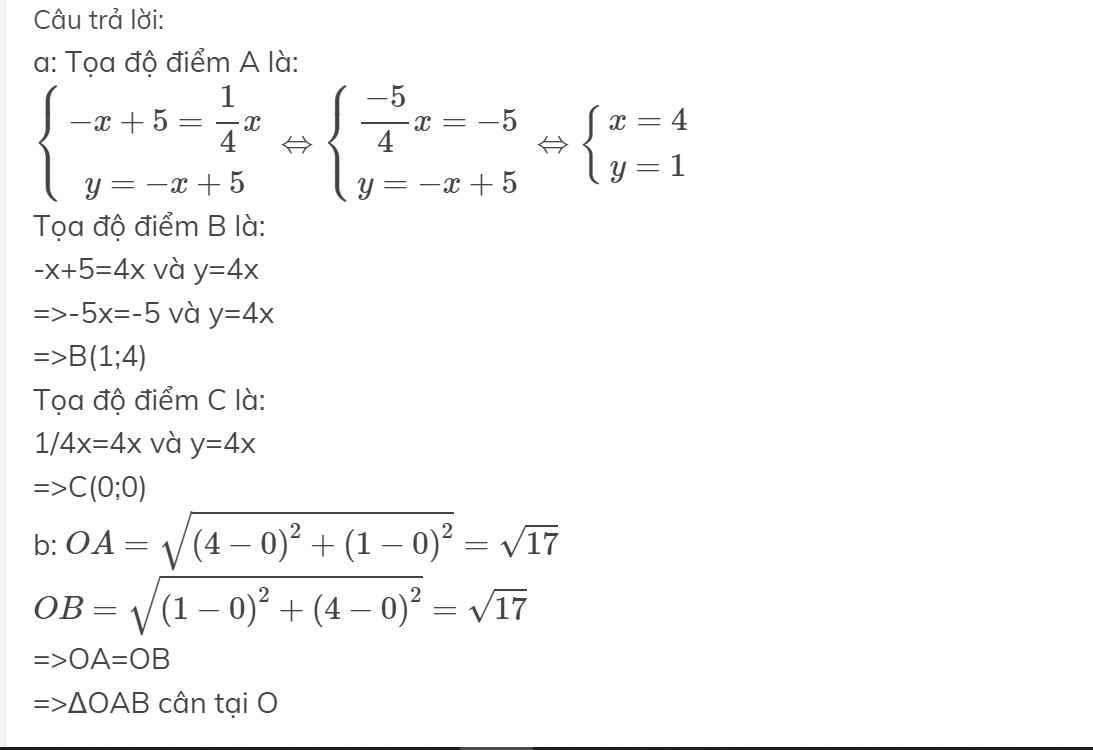
a: Tọa độ A là:
\(\left\{{}\begin{matrix}-x+5=\dfrac{1}{4}x\\y=\dfrac{1}{4}x\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-\dfrac{5}{4}x=-5\\y=\dfrac{1}{4}x\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=4\\y=1\end{matrix}\right.\)
Tọa độ B là:
-x+5=4x và y=4x
=>-5x=-5 và y=4x
=>B(1;4)
Tọa độ C là:
1/4x=4x và y=4x
=>C(0;0)
b: A(4;1); B(1;4); O(0;0)
\(OA=\sqrt{4^2+1^2}=\sqrt{17}\)
\(OB=\sqrt{4^2+1^2}=\sqrt{17}\)
=>OA=OB
=>ΔOAB cân tại O
1: \(O_2D=O_2A+CD=\dfrac{AC}{2}+\dfrac{BC}{2}=\dfrac{AB}{2}=R_1\)
góc O2MD=góc O2MC+góc CMD
=1/2*sđ cung CM+góc MCA
=90 độ
=>DM là tiếp tuyến của (O2)
PD^2=BD*DA=DC*BA=DM^2=O2D-R2^2
=>PD^2=R1^2-R2^2
2: Xet ΔD1BD vuông tại D1 và ΔD4BD vuông tại D4 có
BD chung
góc D1BD=góc D4BD
=>ΔD1BD=ΔD4BD
=>D1=D4
CM tương tự, ta được: DD2=DD3, BP=BQ, PA=PB
=>D1D+D2D+D3D+D4D<=1/2(BP+PA+AQ+QB)
=>2*(D1D+D2D)<=PA+PB
PB^2=BD^2+DP^2>=2*DB*DP
=>\(PB>=\dfrac{2\cdot DB\cdot DP}{PB}=2\cdot D_1D\)
Chứng minh tương tự,ta được: \(AP>=\dfrac{2\cdot DA\cdot DP}{PA}=2\cdot D_2D\)
=>ĐPCM