
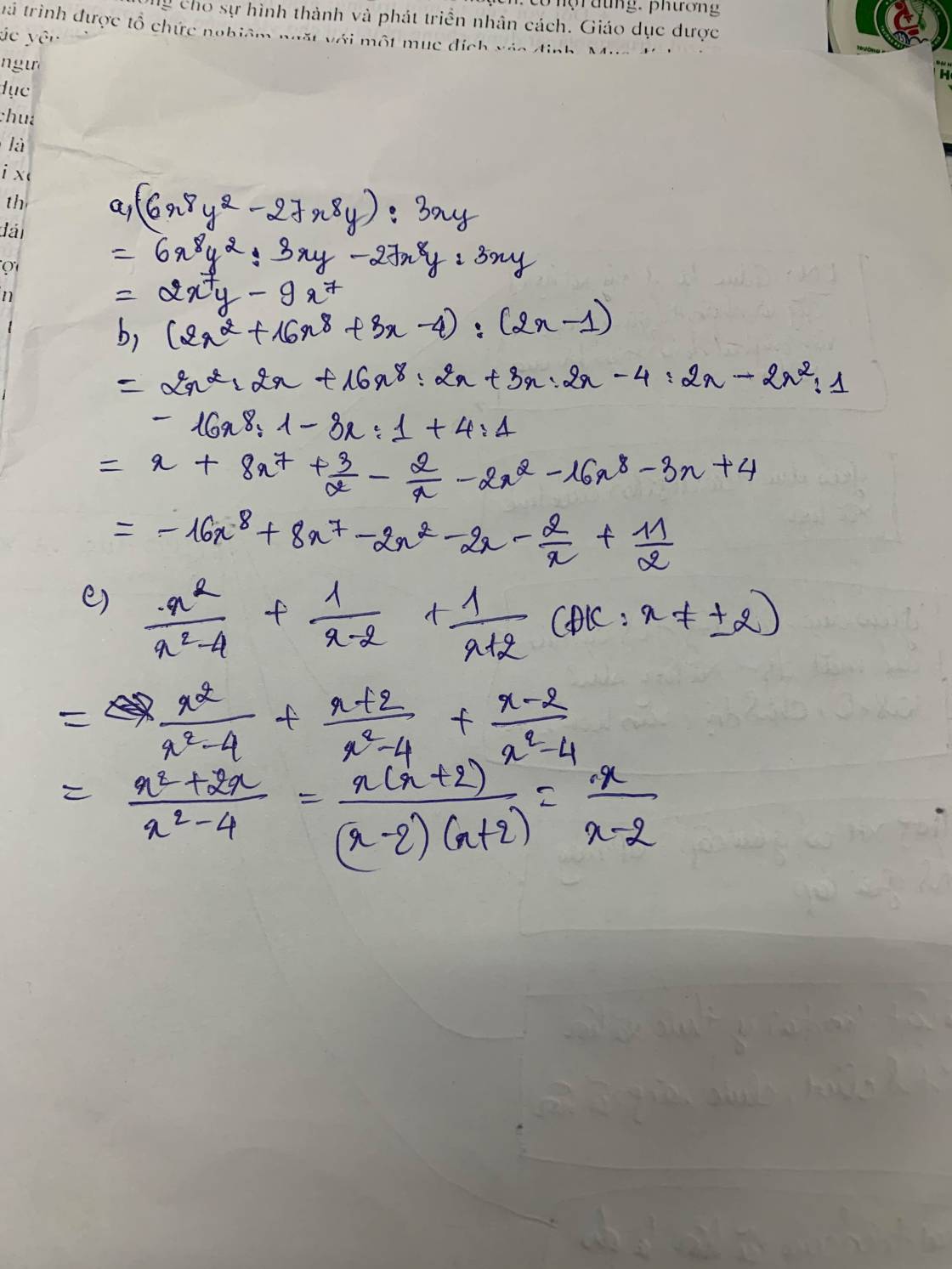
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

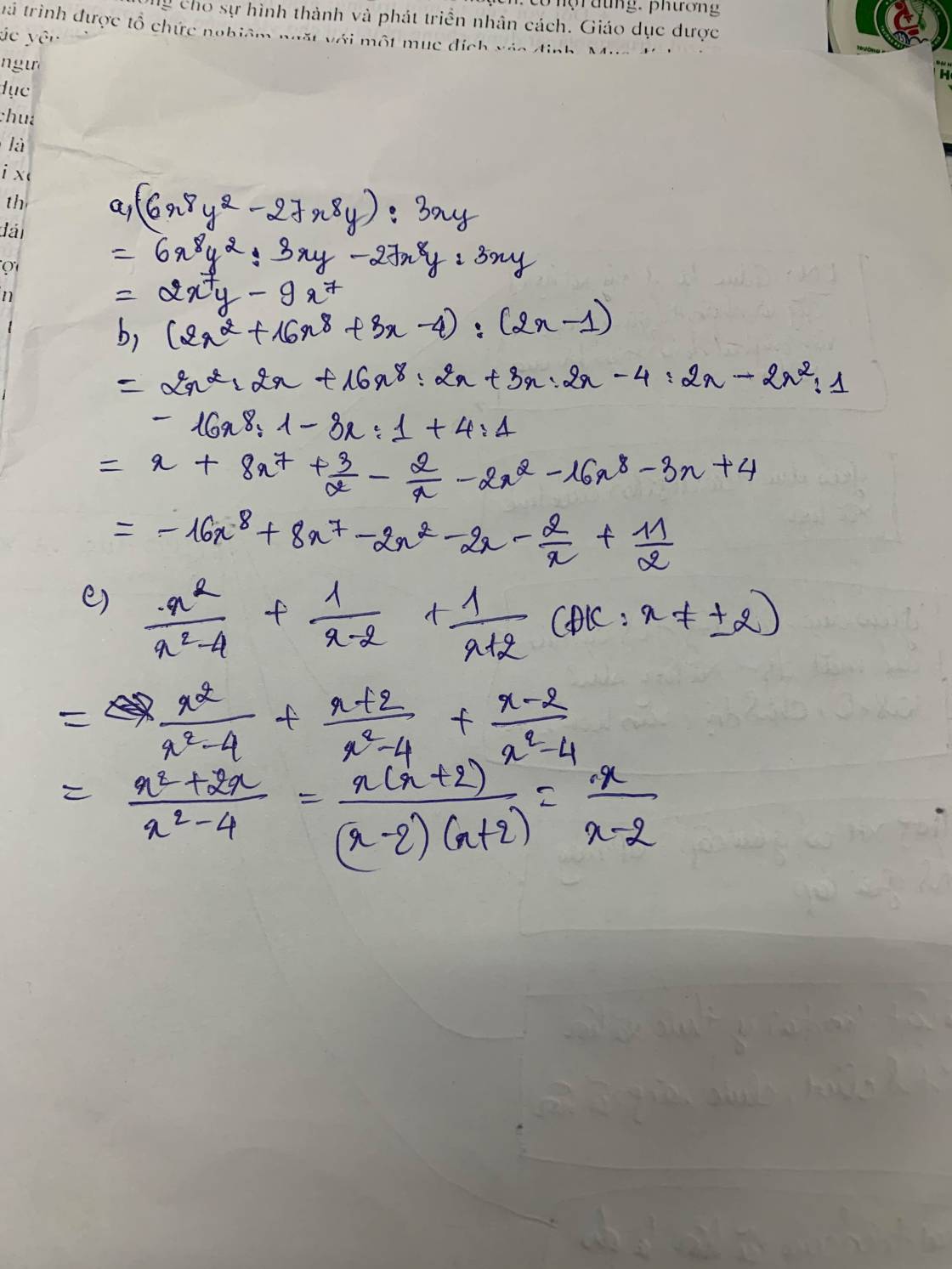



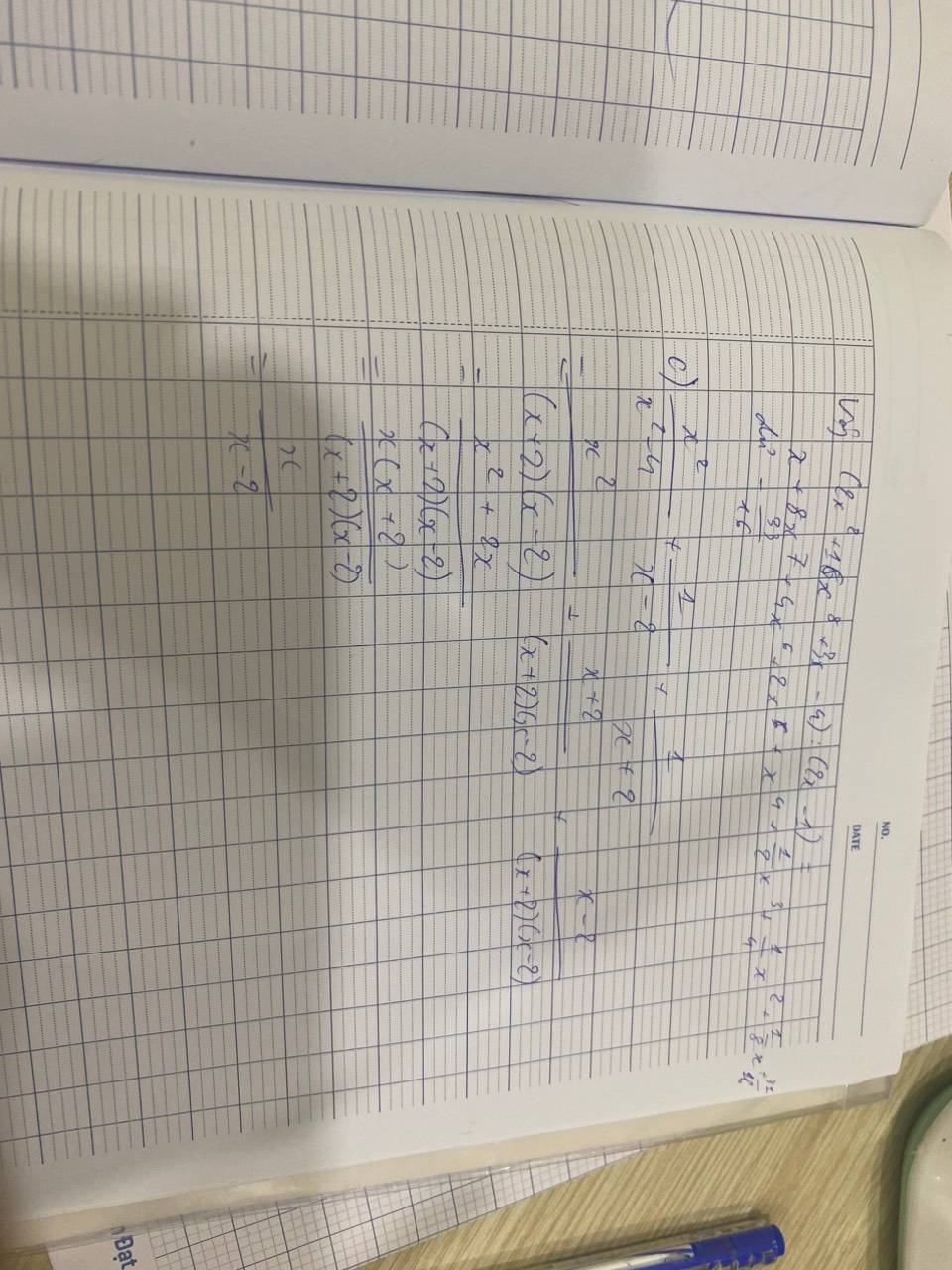
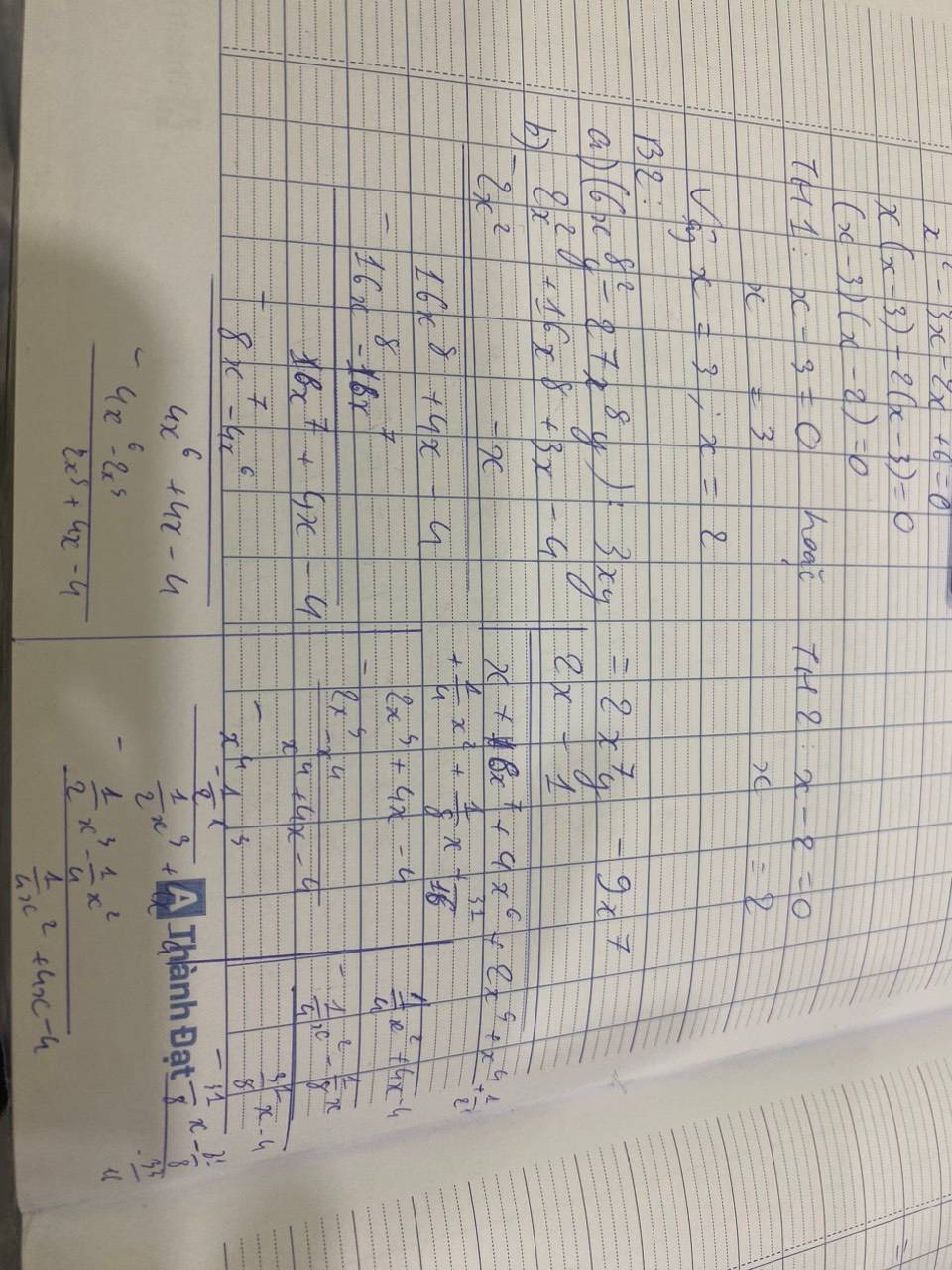
a) \(\left(\right. 6 x^{3} y^{2} - 27 x^{3} y \left.\right) : 3 x y = 2 x^{2} y - 9 x^{2}\).
b) \(\left(\right. \frac{2}{3^{2}} x^{4} \left.\right) . \left(\right. 3 y x^{5} \left.\right) = \left(\right. \frac{2}{9} . 3 \left.\right) \left(\right. x^{4} \cdot x^{5} \left.\right) y = \frac{2}{3} x^{9} y\).
c) \(\frac{x^{2}}{x^{2} - 4} + \frac{1}{x - 2} + \frac{1}{x + 2} = \frac{x^{2}}{\left(\right. x - 2 \left.\right) \left(\right. x + 2 \left.\right)} + \frac{x + 2}{\left(\right. x - 2 \left.\right) \left(\right. x + 2 \left.\right)} + \frac{x - 2}{\left(\right. x - 2 \left.\right) \left(\right. x + 2 \left.\right)} = \frac{x^{2} + x + 2 + x - 2}{\left(\right. x - 2 \left.\right) \left(\right. x + 2 \left.\right)} = \frac{x^{2} + 2 x}{\left(\right. x - 2 \left.\right) \left(\right. x + 2 \left.\right)} = \frac{x \left(\right. x + 2 \left.\right)}{\left(\right. x - 2 \left.\right) \left(\right. x + 2 \left.\right)} = \frac{x}{x - 2}\).
d) \(\frac{2}{x - y} - \left(\right. \frac{x}{x - 1} - \frac{2}{y - x} \left.\right) - \left(\right. \frac{- 2}{x + y} - \frac{x}{x - 1} \left.\right) = \frac{2}{x - y} - \frac{x}{x - 1} + \frac{2}{y - x} + \frac{2}{x + y} + \frac{x}{x - 1} = \left(\right. \frac{2}{x - y} + \frac{2}{y - x} \left.\right) + \left(\right. - \frac{x}{x - 1} + \frac{x}{x - 1} \left.\right) + \frac{2}{x + y} = \frac{2}{x + y}\).

\(a,=\dfrac{5x}{4y^3}\times\left(\dfrac{-20y}{x^4}\right)=\dfrac{-100xy}{4x^4y^3}=\dfrac{-25}{x^3y^2}\\ b,=\dfrac{\left(x-4\right)\left(x+4\right)}{\left(x+4\right)}\times\dfrac{x}{2\left(x-4\right)}=\dfrac{x}{2}\)
\(c,=\dfrac{2\left(x+3\right)}{\left(x-2\right)\left(x^2+2x+4\right)}\times\dfrac{2\left(x-2\right)}{\left(x+3\right)^3}=\dfrac{4}{\left(x+3\right)^2.\left(x^2+2x+4\right)}\)
a) \(\dfrac{5x}{4y^3}:\left(-\dfrac{x^4}{20y}\right)=\dfrac{5x}{4y^3}\cdot\left(-\dfrac{20y}{x^4}\right)=\dfrac{5\cdot-5}{y^2\cdot x^3}=\dfrac{-25}{x^3y^2}\)
b) \(\dfrac{x^2-16}{x+4}:\dfrac{2x-8}{x}=\left(x-4\right)\cdot\dfrac{x}{2\left(x-4\right)}=\dfrac{x}{2}\)
c) \(\dfrac{2x+6}{x^3-8}:\dfrac{\left(x+3\right)^3}{2x-4}=\dfrac{2\left(x+3\right)}{\left(x-2\right)\left(x^2+2x+4\right)}\cdot\dfrac{2\left(x-2\right)}{\left(x+3\right)^3}=\dfrac{4}{\left(x^2+2x+4\right)\left(x+3\right)^2}\)

\(a)\dfrac{{3{\rm{x}} + 6}}{{4{\rm{x}} - 8}}.\dfrac{{2{\rm{x}} - 4}}{{x + 2}} = \dfrac{{3\left( {x + 2} \right).2\left( {x - 2} \right)}}{{4.\left( {x - 2} \right).\left( {x + 2} \right)}} = \dfrac{3}{2}\)
\(b)\dfrac{{{x^2} - 36}}{{2{\rm{x}} + 10}}.\dfrac{{x + 5}}{{6 - x}} = \dfrac{{\left( {x - 6} \right)\left( {x + 6} \right)\left( {x + 5} \right)}}{{2\left( {x + 5} \right).\left( { - 1} \right)\left( {x - 6} \right)}} = \dfrac{{x + 6}}{{ - 2}} = \dfrac{{-x- 6}}{{ 2}}\)
\(c)\dfrac{{1 - {y^3}}}{{y + 1}}.\dfrac{{5y + 5}}{{{y^2} + y + 1}} = \dfrac{{\left( {1 - y} \right)\left( {1 + y + {y^2}} \right).5\left( {y + 1} \right)}}{{\left( {y + 1} \right).\left( {{y^2} + y + 1} \right)}} = 5\left( {1 - y} \right)\)
\(d)\dfrac{{x + 2y}}{{4{{\rm{x}}^2} - 4{\rm{x}}y + {y^2}}}.\left( {2{\rm{x}} - y} \right) = \dfrac{{\left( {x + 2y} \right).\left( {2{\rm{x}} - y} \right)}}{{{{\left( {2{\rm{x}} - y} \right)}^2}}} = \dfrac{{x + 2y}}{{2{\rm{x}} - y}}\)

a)
\(\begin{array}{l}A = 0,2\left( {5{\rm{x}} - 1} \right) - \dfrac{1}{2}\left( {\dfrac{2}{3}x + 4} \right) + \dfrac{2}{3}\left( {3 - x} \right)\\A = x - 0,2 - \dfrac{1}{3}x - 2 + 2 - \dfrac{2}{3}x\\ = \left( {x - \dfrac{1}{3}x - \dfrac{2}{3}x} \right) + \left( {\dfrac{{ - 1}}{2} - 2 + 2} \right)\\ = - \dfrac{1}{2}\end{array}\)
Vậy \(A = - \dfrac{1}{2}\) không phụ thuộc vào biến x
b)
\(\begin{array}{l}B = \left( {x - 2y} \right)\left( {{x^2} + 2{\rm{x}}y + 4{y^2}} \right) - \left( {{x^3} - 8{y^3} + 10} \right)\\B = \left[ {x - {{\left( {2y} \right)}^3}} \right] - {x^3} + 8{y^3} - 10\\B = {x^3} - 8{y^3} - {x^3} + 8{y^3} - 10 = - 10\end{array}\)
Vậy B = -10 không phụ thuộc vào biến x, y.
c)
\(\begin{array}{l}C = 4{\left( {x + 1} \right)^2} + {\left( {2{\rm{x}} - 1} \right)^2} - 8\left( {x - 1} \right)\left( {x + 1} \right) - 4{\rm{x}}\\{\rm{C = 4}}\left( {{x^2} + 2{\rm{x}} + 1} \right) + \left( {4{{\rm{x}}^2} - 4{\rm{x}} + 1} \right) - 8\left( {{x^2} - 1} \right) - 4{\rm{x}}\\C = 4{{\rm{x}}^2} + 8{\rm{x}} + 4 + 4{{\rm{x}}^2} - 4{\rm{x}} + 1 - 8{{\rm{x}}^2} + 8 - 4{\rm{x}}\\C = \left( {4{{\rm{x}}^2} + 4{{\rm{x}}^2} - 8{{\rm{x}}^2}} \right) + \left( {8{\rm{x}} - 4{\rm{x}} - 4{\rm{x}}} \right) + \left( {4 + 1 + 8} \right)\\C = 13\end{array}\)
Vậy C = 13 không phụ thuộc vào biến x

d: \(=\dfrac{x-1}{x^3}-\dfrac{x+1}{x^2\left(x-1\right)}+\dfrac{3}{x\left(x-1\right)^2}\)
\(=\dfrac{\left(x-1\right)^2-x\left(x+1\right)\left(x-1\right)+3x^2}{x^3\left(x-1\right)^2}\)
\(=\dfrac{x^2-2x+1-x^3+x+3x^2}{x^3\left(x-1\right)^2}=\dfrac{-x^3+4x^2-3x+1}{x^3\left(x-1\right)^2}\)
a: \(=\dfrac{x+1}{x+2}:\dfrac{\left(x+2\right)\left(x+1\right)}{\left(x+3\right)^2}\)
\(=\dfrac{x+1}{x+2}\cdot\dfrac{\left(x+3\right)^2}{\left(x+2\right)\left(x+1\right)}=\dfrac{\left(x+3\right)^2}{\left(x+2\right)^2}\)
b: \(=\dfrac{8}{\left(x^2+3\right)\left(x-1\right)\left(x+1\right)}+\dfrac{2\left(x^2-1\right)}{\left(x^2+3\right)\left(x^2-1\right)}+\dfrac{\left(x-1\right)\left(x^2+3\right)}{\left(x-1\right)\left(x+1\right)\left(x^2+3\right)}\)
\(=\dfrac{8+2x^2-2+x^3+3x-x^2-3}{\left(x^2+3\right)\left(x-1\right)\left(x+1\right)}\)
\(=\dfrac{x^3+x^2+3x+3}{\left(x^2+3\right)\left(x-1\right)\left(x+1\right)}=\dfrac{\left(x^2+3\right)\left(x+1\right)}{\left(x^2+3\right)\left(x-1\right)\left(x+1\right)}=\dfrac{1}{x-1}\)
c: \(=\dfrac{x^2+2xy+y^2-x^2+2xy-y^2+4y^2}{2\left(x-y\right)\left(x+y\right)}=\dfrac{4y^2+4xy}{2\left(x-y\right)\left(x+y\right)}\)
\(=\dfrac{4y\left(x+y\right)}{2\left(x-y\right)\left(x+y\right)}=\dfrac{2y}{\left(x-y\right)}\)

\(\dfrac{3}{x-5}-\dfrac{x+1}{x\left(x-5\right)}\left(dkxd:x\ne0,x\ne5\right)\\ =\dfrac{3x-x-1}{x\left(x-5\right)}=\dfrac{2x-1}{x^2-5x}\)
----------------------------------------
\(\dfrac{8\left(y+2\right)}{3x^2}.\dfrac{15x^5}{4\left(y+2\right)^2}\left(dkxd:x\ne0,y\ne-2\right)\\ =\dfrac{8}{4}.\dfrac{15x^2.x^3}{3x^2}=10x^3\)
------------------------------------------
\(\dfrac{8\left(y-1\right)}{3x^2-3}:\dfrac{4\left(y-1\right)^3}{x^2-2x+1}\left(dkxd:x\ne1,x\ne-1\right)\\ =\dfrac{8\left(y-1\right)}{3\left(x-1\right)\left(x+1\right)}.\dfrac{\left(x-1\right)^2}{4\left(y-1\right)^3}\\ =\dfrac{2\left(x-1\right)}{3\left(x+1\right)\left(y-1\right)^2}\)

\(\begin{array}{l}a)\dfrac{{y + 6}}{{{x^2} - 4{\rm{x}} + 4}}.\dfrac{{{x^2} - 4}}{{x + 1}}.\dfrac{{x - 2}}{{y + 6}}\\ = \dfrac{{y + 6}}{{{x^2} - 4{\rm{x}} + 4}}.\dfrac{{x - 2}}{{y + 6}}.\dfrac{{{x^2} - 4}}{{x + 1}}\\ = \dfrac{{\left( {y + 6} \right).\left( {x - 2} \right).\left( {{x^2} - 4} \right)}}{{\left( {{x^2} - 4{\rm{x}} + 4} \right).\left( {y + 6} \right).\left( {x + 1} \right)}}\\ = \dfrac{{\left( {y + 6} \right).\left( {x - 2} \right).\left( {x - 2} \right)\left( {x + 2} \right)}}{{{{\left( {x - 2} \right)}^2}.\left( {y + 6} \right).\left( {x + 1} \right)}} = \dfrac{{x + 2}}{{x + 1}}\end{array}\)
\(\begin{array}{l}b)\left(\frac{2x+1}{{x - 3}} + \frac{2x+1}{x+3}\right ) .\dfrac{{x^2 - 9}}{{2{\rm{x}} + 1}} \\ = (2x+1) \left ( \frac {1}{x-3} + \frac {1}{x+3} \right ) . \frac {(x-3)(x+3)}{2x + 1} \\ = (2x+1) \frac {x+3 + x - 3}{(x-3)(x+3)} . \frac {(x-3)(x+3)}{2x + 1} \\ = \frac {2x(2x+1)}{(x-3)(x+3)} . \frac {(x-3)(x+3)}{2x +1} \\= 2x \end{array}\)