Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.



Bài 8:
a: Thay x=16 vào P, ta được:
\(P=\dfrac{4+2}{4-1}=\dfrac{6}{3}=2\)
b: Thay \(x=3+2\sqrt{2}\) vào P, ta được:
\(P=\dfrac{\sqrt{2}+1+2}{\sqrt{2}+1-1}=\dfrac{3+\sqrt{2}}{\sqrt{2}}=3\sqrt{2}+2\)


a: Ta có: \(A=\dfrac{2x-3\sqrt{x}-14}{x-7\sqrt{x}+12}-\dfrac{\sqrt{x}+4}{\sqrt{x}-3}-\dfrac{\sqrt{x}-1}{\sqrt{x}-4}\)
\(=\dfrac{2x-3\sqrt{x}-14-x+16-x+4\sqrt{x}-3}{\left(\sqrt{x}-3\right)\left(\sqrt{x}-4\right)}\)
\(=\dfrac{\sqrt{x}-1}{\left(\sqrt{x}-3\right)\left(\sqrt{x}-4\right)}\)
Ta có: \(B=\dfrac{x-2\sqrt{x}+1}{x-4\sqrt{x}+3}\)
\(=\dfrac{\left(\sqrt{x}-1\right)^2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}-3\right)}\)
\(=\dfrac{\sqrt{x}-1}{\sqrt{x}-3}\)
b: Ta có: M=A:B
\(=\dfrac{\sqrt{x}-1}{\left(\sqrt{x}-3\right)\left(\sqrt{x}-4\right)}\cdot\dfrac{\sqrt{x}-3}{\sqrt{x}-1}\)
\(=\dfrac{1}{\sqrt{x}-4}\)

Câu 2:
1: \(y=\sqrt{3}+5\)
=>\(\left(\sqrt{3}-1\right)x+4=\sqrt{3}+5\)
=>\(\left(\sqrt{3}-1\right)\cdot x=\sqrt{3}+5-4=\sqrt{3}+1\)
=>\(x=\dfrac{\sqrt{3}+1}{\sqrt{3}-1}=\dfrac{\left(\sqrt{3}+1\right)^2}{3-1}=\dfrac{4+2\sqrt{3}}{2}=2+\sqrt{3}\)
2: \(x^2-2\left(1-m\right)x-2m-5=0\)
=>\(x^2+\left(2m-2\right)x-2m-5=0\)
a: \(\Delta=\left(2m-2\right)^2-4\left(-2m-5\right)\)
\(=4m^2-8m+4+8m+20\)
\(=4m^2+24>=24>0\forall m\)
=>Phương trình luôn có hai nghiệm phân biệt
Câu 1:
2: Thay x=2 và y=-1 vào hệ phương trình, ta được:
\(\left\{{}\begin{matrix}2a-\left(-1\right)=5\\b\cdot2+a\cdot\left(-1\right)=4\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}2a=5+\left(-1\right)=4\\2b-a=4\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}a=2\\2b=a+4=6\end{matrix}\right.\)
=>a=2 và b=3
2: Gọi phân số cần tìm có dạng là \(\dfrac{a}{b}\left(b\ne0\right)\)
Khi tăng mẫu số thêm 4 đơn vị thì phân số đó bằng 1/3 nên ta có:
\(\dfrac{a}{b+4}=\dfrac{1}{3}\)
=>3a=b+4
=>3a-b=4(1)
Khi giảm mẫu số đi 2 đơn vị thì phân số bằng với 2/3 nên ta có:
\(\dfrac{a}{b-2}=\dfrac{2}{3}\)
=>3a=2(b-2)
=>3a=2b-4
=>3a-2b=-4(2)
Từ (1) và (2) ta có hệ phương trình:
\(\left\{{}\begin{matrix}3a-b=4\\3a-2b=-4\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}b=8\\3a-b=4\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}b=8\\3a=b+4=12\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}a=4\\b=8\end{matrix}\right.\)(nhận)
Vậy: Phân số cần tìm là \(\dfrac{4}{8}\)


Bài 1:
Gọi vận tốc và thời gian dự định là a,b
Theo đề, ta có hệ phương trình:
\(\left\{{}\begin{matrix}\left(a+3\right)\left(b-2\right)=ab\\\left(a-3\right)\left(b+3\right)=ab\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-2a+3b=6\\3a-3b=9\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}a=15\\b=6\end{matrix}\right.\)
Vậy: Chiều dài khúc sông là 90km


Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=3\\x_1x_2=1\end{matrix}\right.\)
Gọi \(x_3;x_4\) là các nghiệm của pt cần tìm, ta có:
\(\left\{{}\begin{matrix}x_3+x_4=2x_1-x_2^2+2x_2-x_1^2\\x_3x_4=\left(2x_1-x_2^2\right)\left(2x_2-x_1^2\right)\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}x_3+x_4=2\left(x_1+x_2\right)-\left(x_1+x_2\right)^2+2x_1x_2\\x_3x_4=4x_1x_2-2x_1^3-2x_2^3+\left(x_1x_2\right)^2\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}x_3+x_4=2\left(x_1+x_2\right)-\left(x_1+x_2\right)^2+2x_1x_2\\x_3x_4=4x_1x_2-2\left[\left(x_1+x_2\right)^3-3x_1x_2\left(x_1+x_2\right)\right]+\left(x_1x_2\right)^2\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}x_3+x_4=2.3-3^2+2.1=-1\\x_3x_4=4.1-2\left(3^3-3.1.3\right)+1^2=-31\end{matrix}\right.\)
Theo định lý Viet đảo, pt cần tìm có dạng:
\(x^2+x-31=0\)
 cần giải bài gấp mn ơi giúp với bài 2 với bài 3 thuii
cần giải bài gấp mn ơi giúp với bài 2 với bài 3 thuii 





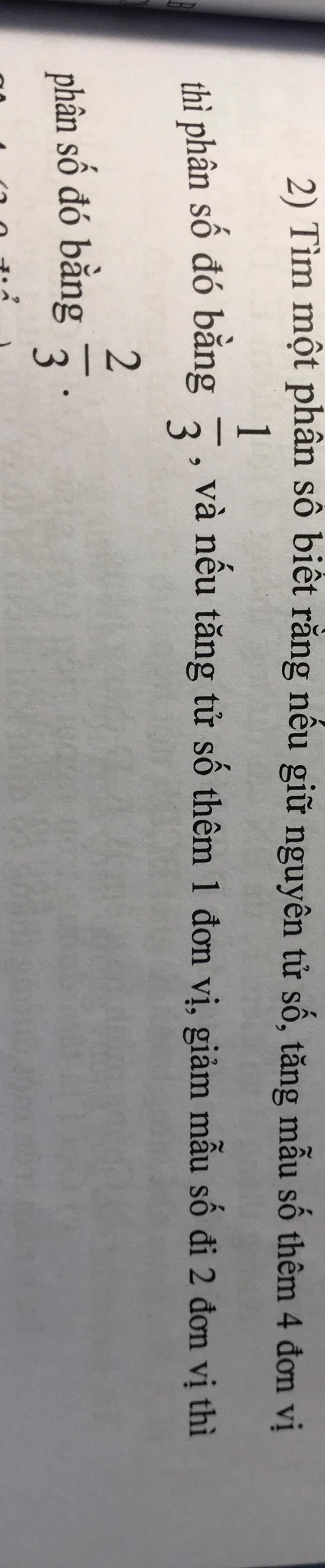

 mn giúp em với em cần gấp bài 2 nha thank you
mn giúp em với em cần gấp bài 2 nha thank you  Mn giúp mình bài 3 với ạ. Mình đang cần gấp lắm. Mình cảm ơn nhiều !
Mn giúp mình bài 3 với ạ. Mình đang cần gấp lắm. Mình cảm ơn nhiều !