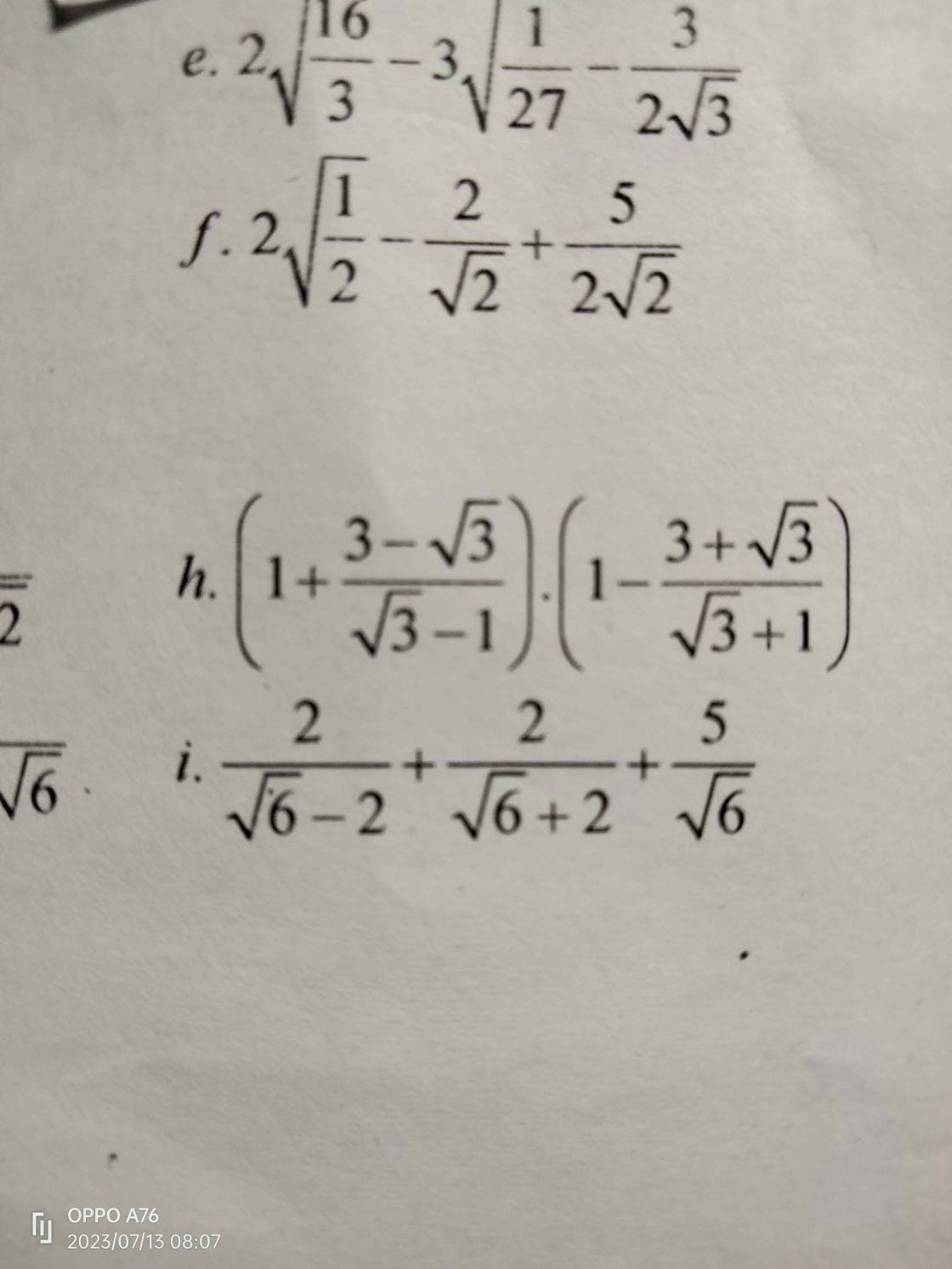K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
13 tháng 7 2023
2\(\sqrt{\dfrac{16}{3}}\) - 3\(\sqrt{\dfrac{1}{27}}\) - \(\dfrac{3}{2\sqrt{3}}\)
= \(\dfrac{8}{\sqrt{3}}\) - \(\dfrac{3}{3\sqrt{3}}\) - \(\dfrac{3}{2\sqrt{3}}\)
= \(\dfrac{8}{\sqrt{3}}\) - \(\dfrac{1}{\sqrt{3}}\) - \(\dfrac{3}{2\sqrt{3}}\)
= \(\dfrac{16}{2\sqrt{3}}\) - \(\dfrac{2}{2\sqrt{3}}\) - \(\dfrac{3}{2\sqrt{3}}\)
= \(\dfrac{11}{2\sqrt{3}}\)
= \(\dfrac{11\sqrt{3}}{6}\)
f, 2\(\sqrt{\dfrac{1}{2}}\)- \(\dfrac{2}{\sqrt{2}}\) + \(\dfrac{5}{2\sqrt{2}}\)
= \(\dfrac{2}{\sqrt{2}}\) - \(\dfrac{2}{\sqrt{2}}\) + \(\dfrac{5}{2\sqrt{2}}\)
= \(\dfrac{5}{2\sqrt{2}}\)
= \(\dfrac{5\sqrt{2}}{4}\)
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
13 tháng 7 2023
(1 + \(\dfrac{3-\sqrt{3}}{\sqrt{3}-1}\)).(1- \(\dfrac{3+\sqrt{3}}{\sqrt{3}+1}\))
= \(\dfrac{\sqrt{3}-1+3-\sqrt{3}}{\sqrt{3}-1}\).\(\dfrac{\sqrt{3}+1-3+\sqrt{3}}{\sqrt{3}+1}\)
= \(\dfrac{2}{\sqrt{3}-1}\).\(\dfrac{-2}{\sqrt{3}+1}\)
= \(\dfrac{-4}{3-1}\)
= \(\dfrac{-4}{2}\)
= -2
NV
Nguyễn Việt Lâm
Giáo viên
10 tháng 1 2022
\(P=\dfrac{x}{1-x^2}+\dfrac{y}{1-y^2}+\dfrac{z}{1-z^2}\)
Ta có: \(2x^2.\left(1-x^2\right)\left(1-x^2\right)\le\dfrac{1}{27}\left(2x^2+1-x^2+1-x^2\right)^3=\dfrac{8}{27}\)
\(\Rightarrow x^2\left(1-x^2\right)^2\le\dfrac{4}{27}\)
\(\Rightarrow x\left(1-x^2\right)\le\dfrac{2}{3\sqrt{3}}\)
\(\Rightarrow\dfrac{x}{1-x^2}\ge\dfrac{3\sqrt{3}}{2}x^2\)
Tương tự và cộng lại:
\(P\ge\dfrac{3\sqrt{3}}{2}\left(x^2+y^2+z^2\right)=...\)
5 tháng 11 2021
\(ĐK:x\ge\dfrac{1}{2}\\ PT\Leftrightarrow\left(5x-5\right)-\left(2\sqrt{2x^2+5x-3}-4\right)-\left(x\sqrt{2x-1}-x\right)+\left(2x\sqrt{x+3}-4x\right)=0\\ \Leftrightarrow5\left(x-1\right)-\dfrac{2\left(2x+7\right)\left(x-1\right)}{\sqrt{2x^2+5x-3}+2}-\dfrac{x\left(2x-2\right)}{\sqrt{2x-1}+1}+\dfrac{2x\left(x-1\right)}{\sqrt{x+3}+2}=0\\ \Leftrightarrow\left(x-1\right)\left(5-\dfrac{2\left(2x+7\right)}{\sqrt{2x^2+5x-3}+2}-\dfrac{x}{\sqrt{2x-1}+1}+\dfrac{2x}{\sqrt{x+3}+2}\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=1\left(tm\right)\\5-\dfrac{2\left(2x+7\right)}{\sqrt{2x^2+5x-3}+2}-\dfrac{x}{\sqrt{2x-1}+1}+\dfrac{2x}{\sqrt{x+3}+2}=0\left(1\right)\end{matrix}\right.\)
Với \(x\ge\dfrac{1}{2}\Leftrightarrow\left(1\right)< 0\)
Do đó PT có nghiệm x=1
5 tháng 11 2021
ĐKXĐ: \(x\ge\dfrac{1}{2}\)
\(2x-2\sqrt{\left(2x-1\right)\left(x+3\right)}-\left(1+x\sqrt{2x-1}-2x\sqrt{x+3}\right)=0\)
\(\Leftrightarrow\left(2x-1\right)-2\sqrt{\left(2x-1\right)\left(x+3\right)}-x\sqrt{2x-1}+2x\sqrt{x+3}=0\)
\(\Leftrightarrow\sqrt{2x-1}\left(\sqrt{2x-1}-x\right)-2\sqrt{x+3}\left(\sqrt{2x-1}-x\right)=0\)
\(\Leftrightarrow\left(\sqrt{2x-1}-x\right)\left(\sqrt{2x-1}-2\sqrt{x+3}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{2x-1}=x\\\sqrt{2x-1}=2\sqrt{x+3}\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}2x-1=x^2\\2x-1=4x+12\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\left(x-1\right)^2=0\\2x=-13\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}x=1\left(tm\right)\\x=-\dfrac{13}{2}\left(ktm\right)\end{matrix}\right.\)
21 tháng 5 2021
Vì \(x=\sqrt{\dfrac{1}{2\sqrt{3}-2}-\dfrac{3}{2\sqrt{3}+2}}=\dfrac{\sqrt{3}-1}{2}\)
\(\Rightarrow x=\dfrac{\sqrt{3}-1}{2}\) là nghiệm của đa thức \(2x^2+2x-1\)
\(\Rightarrow B=\dfrac{2x^{2017}\left(2x^2+2x-1\right)+2x+1}{\left(2x^2+2x-1\right)+x+1}=\dfrac{2x+1}{x+1}=3-\sqrt{3}\)
31 tháng 5 2021
1)Thay x=4 vào biểu thức B ta được:
\(B=\left(\dfrac{x+1}{2}-\sqrt{x}\right)=\left(\dfrac{4+1}{2}-\sqrt{4}\right)=\dfrac{1}{2}\)
2)\(M=\left(\dfrac{1}{\sqrt{x}-1}-\dfrac{1}{\sqrt{x}+1}\right)\left(\dfrac{x+1}{2}-\sqrt{x}\right)\) (đk:\(x\ge0;x\ne1\))
\(=\dfrac{\sqrt{x}+1-\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}.\dfrac{x+1-2\sqrt{x}}{2}\)
\(=\dfrac{2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}.\dfrac{\left(\sqrt{x}-1\right)^2}{2}\)
\(=\dfrac{\sqrt{x}-1}{\sqrt{x}+1}\)
3) \(M=\dfrac{\sqrt{x}}{6}\)
=> \(\dfrac{\sqrt{x}-1}{\sqrt{x}+1}=\dfrac{\sqrt{x}}{6}\) \(\Leftrightarrow6\left(\sqrt{x}-1\right)=\sqrt{x}\left(\sqrt{x}+1\right)\)
\(\Leftrightarrow x-5\sqrt{x}+6=0\) \(\Leftrightarrow\)\(\left[{}\begin{matrix}\sqrt{x}=3\\\sqrt{x}=2\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}x=9\\x=4\end{matrix}\right.\) (thỏa)
Vậy...
L
31 tháng 5 2021
a) \(x=4\rightarrow\sqrt{x}=2\) (TMĐK)
Thay \(\sqrt{x}=2\) vào A ta có :
\(A=\left(\dfrac{1}{2-1}-\dfrac{1}{2+1}\right)=\left(1-\dfrac{1}{3}\right)=\dfrac{2}{3}\)
b) M=A.B
\(\rightarrow M=\left(\dfrac{1}{\sqrt{x}-1}-\dfrac{1}{\sqrt{x}+1}\right).\left(\dfrac{x+1}{2}-\sqrt{x}\right)\)
\(\rightarrow M=\left(\dfrac{\sqrt{x}+1-\sqrt{x}+1}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}\right).\left(\dfrac{x+1-2\sqrt{x}}{2\sqrt{x}}\right)\)
\(\rightarrow M=\dfrac{2}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}.\dfrac{\left(\sqrt{x}-1\right)^2}{2\sqrt{x}}\)
\(\rightarrow M=\dfrac{\sqrt{x}-1}{\sqrt{x}\left(\sqrt{x}+1\right)}\)
\(M=\dfrac{\sqrt{x}}{6}\)
\(\rightarrow\dfrac{\sqrt{x}-1}{\sqrt{x}\left(\sqrt{x}+1\right)}=\dfrac{\sqrt{x}}{6}\)
\(\rightarrow6\left(\sqrt{x}-1\right)=\sqrt{x}+1\)
\(\rightarrow6\sqrt{x}-6-\sqrt{x}-1=0\)
\(\rightarrow5\sqrt{x}-7=0\)
\(\rightarrow\sqrt{x}=\dfrac{7}{5}\)
\(\rightarrow x=\pm\dfrac{5\sqrt{7}}{5}\)
\(\rightarrow x=\dfrac{5\sqrt{7}}{7}\) (TMĐK)
AH
Akai Haruma
Giáo viên
23 tháng 7 2021
Lời giải:
a. Số tiền phạt cho $20$ kg hành lý quá cước là:
$T=\frac{4}{5}.20+20=36$ (USD)
b.
$651980$ VNĐ = $\frac{651980}{23285}=28$ USD
Ta có: $28=\frac{4}{5}M+20$
$8=\frac{4}{5}M$
$M=10$ (kg)
Vậy khối lượng hành lý quá cước là $10$ kg.
17 tháng 8 2021
c: Phương trình hoành độ giao điểm của \(\left(d1\right),\left(d2\right)\) là:
x-2=3x-4
\(\Leftrightarrow x-3x=-4+2\)
\(\Leftrightarrow-2x=-2\)
hay x=1
Thay x=1 vào y=x-2, ta được:
y=1-2=-1
Thay x=1 và y=-1 vào \(\left(d\right)\), ta được:
\(m^2-3m+1+m-1=-1\)
\(\Leftrightarrow m^2-2m+1=0\)
\(\Leftrightarrow m-1=0\)
hay m=1