Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(x^{2^{ }}+2\left(m-1\right)x-6m-7=0\left(1\right)\)
a) \(Dental=\left[2\left(m-1\right)\right]^2-4\cdot1\cdot\left(-6m-7\right)\)
\(< =>4\cdot\left(m^2-2m+1\right)+24m+28\)
\(< =>4m^2-8m+4+24m+28\)
\(< =>4m^2+16m+32\)
\(< =>\left(2m+4\right)^2+16>0\) với mọi m
Vậy phương (1) luôn có 2 nghiệm phân biệt với mọi m
b) Theo định lí vi ét ta có:
x1+x2= \(\dfrac{-2\left(m-1\right)}{1}=-2m+1\)
x1x2= \(-6m-7\)
quy đồng
khử mẫu
tách sao cho có tích và tổng
thay x1x2 x1+x2
kết luận
mặt xấu vl . . .![]()

PT có 2 nghiệm `x_1,x_2`
`<=>\Delta>0`
`<=>(2m+3)^2-4m>0`
`<=>4m^2+12m+9-4m>0`
`<=>4m^2+8m+9>0``
`<=>(2m+2)^2+5>0`(luôn đúng)
Áp dụng vi-ét:$\begin{cases}x_1+x_2=2m+3\\x_1.x_2=m\end{cases}$
$x_1^2+x_2^2\\=(x_1+x_2)^2-2x_1.x_2\\=(2m+3)^2-2m\\=4m^2+12m+9-2m\\=4m^2+10m+9\\=(2m+\dfrac52)^2+\dfrac{11}{4} \geq \dfrac{11}{4}$
Dấu "=" `<=>2m=-5/2<=>m=-5/4`

Δ=(2m-2)^2-4(2m-5)
=4m^2-8m+4-8m+20
=4m^2-16m+24
=4m^2-16m+16+8=(2m-4)^2+8>=8>0 với mọi m
=>Phương trình luôn có hai nghiệm phân biệt
\(B=\dfrac{x_1^2}{x^2_2}+\dfrac{x_2^2}{x_1^2}\)
\(=\dfrac{x_1^4+x_2^4}{\left(x_1\cdot x_2\right)^2}=\dfrac{\left(x_1^2+x_2^2\right)^2-2\left(x_1\cdot x_2\right)^2}{\left(x_1\cdot x_2\right)^2}\)
\(=\dfrac{\left[\left(2m-2\right)^2-2\left(2m-5\right)\right]^2-2\left(2m-5\right)^2}{\left(2m-5\right)^2}\)
\(=\dfrac{\left(4m^2-8m+4-4m+10\right)^2}{\left(2m-5\right)^2}-2\)
\(=\left(\dfrac{4m^2-12m+14}{2m-5}\right)^2-2\)
\(=\left(\dfrac{4m^2-10m-2m+5+9}{2m-5}\right)^2-2\)
\(=\left(2m-1+\dfrac{9}{2m-5}\right)^2-2\)
Để B nguyên thì \(2m-5\in\left\{1;-1;3;-3;9;-9\right\}\)
=>\(m\in\left\{3;2;4;1;7\right\}\)

a) Phương trình có hai nghiệm phân biệt khi:
\(\Delta=9-4\left(m+1\right)>0\) \(\Leftrightarrow m< \dfrac{5}{4}\)
Vậy \(\ m< \dfrac{5}{4}\) thì pt có hai nghiệm phân biệt.
b) Áp dụng hệ thức viet có:
\(\left\{{}\begin{matrix}x_1+x_2=-3\\x_1.x_2=m+1\end{matrix}\right.\)
\(P=\left(x_1+x_2\right)^2-4x_1.x_2+7m+5.x_1x_2\)
\(=9-4\left(m+1\right)+7m+5\left(m+1\right)\)
\(=8m+10\)
Không tồn tại giá trị lớn nhất. Em xem lại đề
Trên đó em ko hề có ghi là tìm m để pt có 2 nghiệm phân biệt. Vậy nên phải là m \(\le\dfrac{5}{4}\). KQ: Giá trị lớn nhất của P = 20 khi m = \(\dfrac{5}{4}\)

a. + Với m = − 1 2 phương trình (1) trở thành x 2 − 4 x = 0 ⇔ x = 0 x = 4 .
+ Vậy khi m = − 1 2 phương trình có hai nghiệm x= 0 và x= 4.
b. + Phương trình có hai nghiệm dương phân biệt khi
Δ = 2 m + 5 2 − 4 2 m + 1 > 0 x 1 + x 2 = 2 m + 5 > 0 x 1 . x 2 = 2 m + 1 > 0
+ Ta có Δ = 2 m + 5 2 − 4 2 m + 1 = 4 m 2 + 12 m + 21 = 2 m + 3 2 + 12 > 0 , ∀ m ∈ R
+ Giải được điều kiện m > − 1 2 (*).
+ Do P>0 nên P đạt nhỏ nhất khi P 2 nhỏ nhất.
+ Ta có P 2 = x 1 + x 2 − 2 x 1 x 2 = 2 m + 5 − 2 2 m + 1 = 2 m + 1 − 1 2 + 3 ≥ 3 ( ∀ m > − 1 2 ) ⇒ P ≥ 3 ( ∀ m > − 1 2 ) .
và P = 3 khi m= 0 (thoả mãn (*)).
+ Vậy giá trị nhỏ nhất P = 3 khi m= 0.

a)
\(x=-2\) là nghiệm của phương trình
\(\Rightarrow\left(-2\right)^2-\left(-2\right).\left(m-1\right).\left(-2\right)-3=0\)
\(\Leftrightarrow4+4\left(m-1\right)-3=0\)
\(\Leftrightarrow4\left(m-1\right)=-1\)
\(\Leftrightarrow m-1=-\dfrac{1}{4}\)
\(\Leftrightarrow m=\dfrac{3}{4}\)
\(x^2-2\left(m-1\right)x-3=0\)
\(\Leftrightarrow x^2+\dfrac{1}{2}x-3=0\)
\(\Leftrightarrow2x^2+x-6=0\)
\(\Leftrightarrow\left(x+2\right)\left(2x-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x+2=0\\2x-3=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-2\\x=\dfrac{3}{2}\end{matrix}\right.\)
b)
\(\Delta'=\left(m-1\right)^2+12x>0\forall m\)
\(\Rightarrow\) Phương trình luôn có 2 nghiệm phân biệt
Theo Viet: \(\left\{{}\begin{matrix}x_1+x_2=2\left(m-1\right)\\x_1x_2=-3\end{matrix}\right.\)
Có:
\(Q=x_1^3x_2+x_1x_2^3-5x_1x_2\)
\(=x_1x_2.\left[\left(x_1+x_2\right)^2-2x_1x_2\right]-5x_1x_2\)
\(=-3\left[4\left(m-1\right)^2+6\right]+15\)
\(=-12\left(m-1\right)^2-3\)
Mà \(-12\left(m-1\right)^2\le0\)
\(\Rightarrow-12\left(m-1\right)^2-3\le-3\)
\(Max_Q=-3\Leftrightarrow m-1=0\Leftrightarrow m=1\).
`a)` Thay `x=-2` vào ptr có:
`(-2)^2-2(m-1).(-2)-3=0<=>m=3/4`
Thay `m=3/4` vào ptr có: `x^2-2(3/4-1)x-3=0<=>x^2+1/2x-3=0`
`<=>2x^2+x-6=0<=>(x+2)(2x-3)=0<=>[(x=-2),(x=3/2):}`
`b)` Ptr có nghiệm `<=>\Delta' >= 0`
`<=>[-(m-1)]^2+3 >= 0<=>(m-1)^2+3 >= 0` (LĐ `AA m`)
`=>` Áp dụng Viét có: `{(x_1+x_2=[-b]/a=2m-2),(x_1 .x_2=c/a=-3):}`
Có:`Q=x_1 ^3 x_2+x_1 x_2 ^3 -5x_1 x_2`
`<=>Q=x_1 x_2(x_1 ^2+x_2 ^2)-5x_1 x_2`
`<=>Q=x_1 x_2[(x_1+x_2)^2-2x_1 x_2]-5x_1 x_2`
`<=>Q=-3[(2m-2)^2-2.(-3)]-5.(-3)`
`<=>Q=-3(2m-2)^2-18+15`
`<=>Q=-3(2m-2)^2-3`
Vì `-3(2m-2)^2 <= 0<=>-3(2m-2)^2-3 <= -3 AA m`
`=>Q <= -3 AA m`
Dấu "`=`" xảy ra `<=>2m-2=0<=>m=1`
Vậy GTLN của `Q` là `-3` khi `m=1`
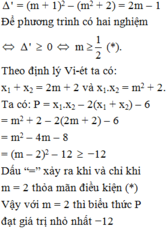

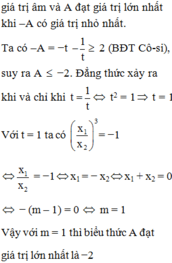
\(x^2-2\left(m-3\right)x-6m-7\\\Delta'=\left(m-3\right)^2-\left(-6m-7\right)=m^2-6m+9+6m+7\\ =m^2+16>0\forall m\)
=> pt luôn có 2 no pb
theo viet \(\Rightarrow\left\{{}\begin{matrix}x_1+x_2=2\left(m-3\right)\\x_1.x_2=-6m-7\end{matrix}\right.\)
\(C=\left(x_1+x_2\right)^2+8x_1x_2\\ =\left[2\left(m-3\right)\right]^2+8\left(-6m-7\right)\\ =4\left(m-3\right)^2-48m-56\\ =4\left(m^2-6m+9\right)-48m-56\\ =4m^2-72m-20\\ =\left(2m\right)^2-2.2m.18+18^2-344\\ =\left(2m-18\right)^2-344\)
có \(\left(2m-18\right)^2\ge0\forall m\\ \Rightarrow\left(2m-18\right)^2-344\ge-344\)
vậy..
\(C=\left(x_1+x_2\right)^2+8x_1x_2\)
\(=\left(2m-6\right)^2+8\left(-6m-7\right)\)
\(=4m^2-24m+36-48m-56\)
\(=4m^2-72m-20\)
\(=4m^2-72m+324-344\)
\(=\left(2m-18\right)^2-344\ge-344\forall x\)
Dấu '=' xảy ra khi m=9