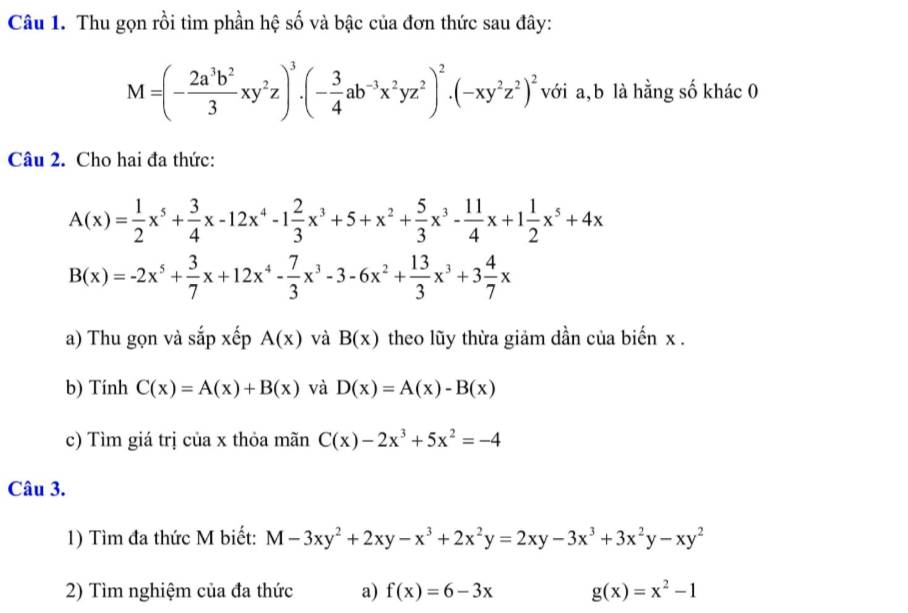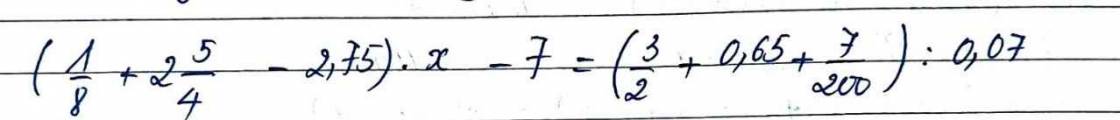Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.



1:
a: f(x)=3x^2-15x+7x^3-2x^2-4x=7x^3+x^2-19x
Bậc là 3
g(x)=x^2-6x+9+7+3x^2-x^3=-x^3+4x^2-6x+16
Bậc là 3
b: f(x)+g(x)
=7x^3+x^2-19x-x^3+4x^2-6x+16
=6x^3+5x^2-25x+16
f(x)-g(x)
=7x^3+x^2-19x+x^3-4x^2+6x-16
=8x^3-3x^2-13x-16
c: f(-1)=-7+1+19=13
g(-2/3)=8/27+4*4/9-6*(-2/3)+16=596/27
2:
a: f(x)=4x^3-12x^2-10x-14
g(x)=4x^3-24x^2-7x^2+15x^4=15x^4+4x^3-31x^2
Bậc của f(x) là 3
Bậc của g(x) là 4
b: f(x)+g(x)
=4x^3-12x^2-10x-14+15x^4+4x^3-31x^2
=15x^4+8x^3-43x^2-10x-14
f(x)-g(x)
=4x^3-12x^2-10x-14-15x^4-4x^3+31x^2
=-15x^4+19x^2-10x-14
c: f(-1)=-4-12+10=-6
g(-2/3)=15*16/81+4*(-8/27)-31*(-2/3)^2
=-12

Câu 1.
\(M=\left(-\dfrac{2a^3b^2}{3}xy^2z\right)^3.\left(-\dfrac{3}{4}ab^{-3}x^2yz^2\right)^2.\left(-xy^2z^2\right)^2\)
\(=\left(-\dfrac{8}{27}a^9b^6x^3y^6z^3\right).\left(\dfrac{9}{16}a^2b^{-6}x^4y^2z^4\right).\left(x^2y^4z^4\right)\)
\(=-\dfrac{8}{27}.\dfrac{9}{16}.a^{11}x^9y^{12}z^{11}\)
\(=-\dfrac{1}{6}a^{11}x^9y^{12}z^{11}\)
Hệ số: \(-\dfrac{1}{6}\)
Bậc: \(43\)
Câu 2.
a) \(A\left(x\right)=\dfrac{1}{2}x^5+\dfrac{3}{4}x-12x^4-1\dfrac{2}{3}x^3+5+x^2+\dfrac{5}{3}x^3-\dfrac{11}{4}x+1\dfrac{1}{2}x^5+4x\)
\(=\left(\dfrac{1}{2}x^5+\dfrac{3}{2}x^5\right)+\left(-12x^4\right)+\left(-\dfrac{5}{3}x^3+\dfrac{5}{3}x^3\right)+x^2+\left(\dfrac{3}{4}x-\dfrac{11}{4}x+4x\right)+5\)
\(=2x^5-12x^4+x^2+2x+5\)
\(B\left(x\right)=-2x^5+\dfrac{3}{7}x+12x^4-\dfrac{7}{3}x^3-3-6x^2+\dfrac{13}{3}x^3+3\dfrac{4}{7}x\)
\(=\left(-2x^5\right)+12x^4+\left(-\dfrac{7}{3}x^3+\dfrac{13}{3}x^3\right)-6x^2+\left(\dfrac{3}{7}x+\dfrac{25}{7}x\right)-3\)
\(=-2x^5+12x^4+2x^3-6x^2+4x-3\)
b) \(C\left(x\right)=A\left(x\right)+B\left(x\right)=\left(2x^5-12x^4+x^2+2x+5\right)+\left(-2x^5+12x^4+2x^3-6x^2+4x-3\right)\)
\(=\left(2x^5-2x^5\right)+\left(-12x^4+12x^4\right)+2x^3+\left(x^2-6x^2\right)+\left(2x+4x\right)+\left(5-3\right)\)
\(=2x^3-5x^2+6x+2\)
\(D\left(x\right)=A\left(x\right)-B\left(x\right)=\left(2x^5-12x^4+x^2+2x+5\right)-\left(-2x^5+12x^4+2x^3-6x^2+4x-3\right)\)
\(=\left(2x^5+2x^5\right)+\left(-12x^4-12x^4\right)-2x^3+\left(x^2+6x^2\right)+\left(2x-4x\right)+\left(5+3\right)\)
\(=4x^5-24x^4-2x^3+7x^2-2x+8\)
c) \(2x^3-5x^2+6x+2-2x^3+5x^2=-4\)
\(\Rightarrow\left(2x^3-2x^3\right)+\left(-5x^2+5x^2\right)+6x+2\)
\(\Rightarrow6x+2=-4\)
\(\Rightarrow6x=-6\)
\(\Rightarrow x=-1\)
Câu 3.
1) \(M-3xy^2+2xy-x^3+2x^2y=2xy-3x^3+3x^2y-xy^2\)
\(\Rightarrow M=\left(3xy^2+2xy-x^3+2x^2y\right)+\left(2xy-3x^3+3x^2y-xy^2\right)\)
\(=\left(3xy^2-xy^2\right)+\left(2xy+2xy\right)+\left(-x^3-3x^3\right)+\left(2x^2y+3x^2y\right)\)
\(=2xy^2+4xy-4x^3+5x^2y\)
2)
Để cho \(f\left(x\right)\) có nghiệm thì \(6-3x=0\)
\(\Rightarrow3x=6\)
\(\Rightarrow x=2\)
Để cho \(g\left(x\right)\) có nghiệm thì \(x^2-1=0\)
\(\Rightarrow x^2=1\)
\(\Rightarrow\left[{}\begin{matrix}x=1\\x=-1\end{matrix}\right.\)

3n + 3 + 3n + 1 + 2n + 3 + 2n + 2
= 3n.33 + 3n.3 + 2n.23 + 2n.22
= 3n.(27 + 3) + 2n.(8 + 4)
= 3n.30 + 2n.12
= 3n.5.6 + 2n.2.6
= 6.(3n.5 + 2n.2) \(⋮\) 6


Cộng trừ hai số hữu tỉ
B6:
a, \(\frac{-1}{21}+\frac{-1}{28}\) = \(\frac{-1}{12}\)
\(b,\frac{-8}{18}-\frac{15}{27}\) = -1
\(c,\frac{-5}{12}+0,75=\frac{1}{3}\)
\(d,3,5-\left(\frac{-2}{7}\right)=\frac{53}{14}\)
\(B8:\)
\(a,\frac{3}{7}+\left(\frac{-5}{2}\right)+\left(\frac{-3}{5}\right)\)
= \(\frac{3}{7}-\frac{5}{2}-\frac{3}{5}\)
\(=\frac{-187}{70}\)
\(b,\left(\frac{-4}{3}\right)+\left(\frac{-2}{5}\right)+\left(\frac{-3}{2}\right)\)
\(=\frac{-4}{3}-\frac{2}{5}-\frac{3}{2}\)
\(=\frac{-97}{30}\)
\(c,\frac{4}{5}-\left(\frac{2}{7}\right)-\frac{7}{10}\)
\(=\frac{4}{5}+\frac{2}{7}-\frac{7}{10}\)
\(=\left(\frac{4}{5}-\frac{7}{1}\right)+\frac{2}{7}\)
\(=\frac{1}{10}+\frac{2}{7}\)
\(=\frac{27}{70}\)
\(d,\frac{2}{3}-\left[\left(\frac{-7}{4}\right)-\left(\frac{1}{2}+\frac{3}{8}\right)\right]\)
\(=\frac{2}{3}-\left(\frac{-7}{4}-\frac{7}{8}\right)\)
\(=\frac{2}{3}-\left(\frac{-21}{8}\right)\)
\(=\frac{79}{24}\)
Làm từng bài một nha![]()
Chẳng biết đúng hay sai nữa ![]()

2\(\dfrac{5}{4}\) là một hỗn số hả em, như vậy không hợp lý vì phần phân số phải bé hơn 1