Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a) <=>
Miền nghiệm của hệ bất phương trình là miền không bị gạch sọc ở hình bên (không kể các điểm).
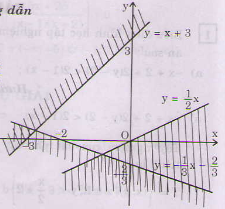
b) <=>
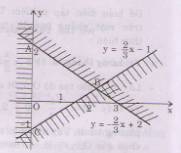
Miền nghiệm của hệ bất phương trình là miền tam giác ABC bao gồm cả các điểm trên cạnh AC và cạnh BC (không kể các điểm của cạnh AB).

1. \(\left\{{}\begin{matrix}x+y+\dfrac{1}{x}+\dfrac{1}{y}=5\\x^2+y^2+\dfrac{1}{x^2}+\dfrac{1}{y^2}=9\end{matrix}\right.\) ĐKXĐ : \(\left\{{}\begin{matrix}x>0\\y>0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x^2y+xy^2+x+y=5xy\\x^4y^2+x^2y^4+x^2+y^2=9x^2y^2\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x^4y^2+x^2y^4+x^2+y^2=25x^2y^2\\x^4y^2+x^2y^4+x^2+y^2=9x^2y^2\end{matrix}\right.\)\(\Leftrightarrow0=16x^2y^2\)
\(\Rightarrow\) phương trình vô nghiệm

a: \(\left\{{}\begin{matrix}\dfrac{2}{x}+\dfrac{3}{y}=5\\\dfrac{1}{x}-\dfrac{4}{y}=-3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\dfrac{2}{x}+\dfrac{3}{y}=5\\\dfrac{2}{x}-\dfrac{8}{y}=-6\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\dfrac{11}{y}=11\\\dfrac{1}{x}-\dfrac{4}{y}=-3\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}y=1\\\dfrac{1}{x}=-3+\dfrac{4}{y}=-3+4=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=1\end{matrix}\right.\)
b: \(\left\{{}\begin{matrix}\dfrac{12}{x-3}-\dfrac{5}{y+2}=63\\\dfrac{8}{x-3}+\dfrac{15}{y+2}=-13\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\dfrac{36}{x-3}-\dfrac{15}{y+2}=189\\\dfrac{8}{x-3}+\dfrac{15}{y+2}=-13\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{44}{x-3}=176\\\dfrac{8}{x-3}+\dfrac{15}{y+2}=-13\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x-3=\dfrac{1}{4}\\\dfrac{15}{y+2}=-13-\dfrac{8}{x-3}=-13-32=-45\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{13}{4}\\y=-\dfrac{1}{3}-2=-\dfrac{7}{3}\end{matrix}\right.\)

Bài 1:
Khi $m=1$ thì HPT trở thành:
\(\left\{\begin{matrix} x-2y=-1\\ 2x+y=2\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} 2x-4y=-2\\ 2x+y=2\end{matrix}\right.\)
\(\Rightarrow (2x+y)-(2x-4y)=2-(-2)\)
\(\Leftrightarrow 5y=4\Rightarrow y=\frac{4}{5}\)
\(x=\frac{2-y}{2}=\frac{2-\frac{4}{5}}{2}=\frac{3}{5}\)
Vậy ...........
b)
HPT \(\Leftrightarrow \left\{\begin{matrix} mx-2y=m-2\\ y=m+1-2x\end{matrix}\right.\Rightarrow mx-2(m+1-2x)=m-2\)
\(\Leftrightarrow x(m+4)=3m(*)\)
Để HPT ban đầu có bộ nghiệm (x,y) duy nhất thì PT $(*)$ phải có nghiệm $x$ duy nhất. Điều này xảy ra khi $m+4\neq 0$ hay $m\neq -4$
Bài 2:
a)
Khi $m=2$ thì hệ trở thành:
\(\left\{\begin{matrix}
x+2y=1\\
2x+y=1\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix}
2x+4y=2\\
2x+y=1\end{matrix}\right.\)
\(\Rightarrow (2x+4y)-(2x+y)=2-1\)
\(\Leftrightarrow 3y=1\Rightarrow y=\frac{1}{3}\)
Khi đó: \(x=1-2y=1-2.\frac{1}{3}=\frac{1}{3}\)
Vậy HPT có bộ nghiệm duy nhất $(x,y)=(\frac{1}{3}, \frac{1}{3})$
b)
HPT \(\Leftrightarrow \left\{\begin{matrix} x=1-my\\ mx+y=1\end{matrix}\right.\Rightarrow m(1-my)+y=1\)
\(\Leftrightarrow y(1-m^2)=1-m(*)\)
Để HPT ban đầu có nghiệm duy nhất thì PT $(*)$ cũng phải có nghiệm duy nhất. Điều này xảy ra khi \(1-m^2\neq 0\Leftrightarrow m\neq \pm 1\)
Khi đó:
\(y=\frac{1-m}{1-m^2}=\frac{1}{1+m}\)
\(x=1-my=1-\frac{m}{m+1}=\frac{1}{m+1}\)
Vậy HPT có nghiệm \((x,y)=(\frac{1}{m+1}, \frac{1}{m+1})\)
Để \(x,y>0\Leftrightarrow \frac{1}{m+1}>0\Leftrightarrow m>-1\)
Kết hợp những điều vừa tìm được suy ra $m>-1$ và $m\neq 1$ thì thỏa mãn.

c. ĐKXĐ: ...
\(x^2+y^2+2xy-2xy+\dfrac{2xy}{x+y}-1=0\)
\(\Leftrightarrow\left(x+y\right)^2-1-2xy\left(1-\dfrac{1}{x+y}\right)=0\)
\(\Leftrightarrow\left(x+y-1\right)\left(x+y+1\right)-\dfrac{2xy\left(x+y-1\right)}{x+y}=0\)
\(\Leftrightarrow\left(x+y-1\right)\left(x+y+1-\dfrac{2xy}{x+y}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x+y=1\\x^2+y^2+x+y=0\left(vô-nghiệm\right)\end{matrix}\right.\)
Thế \(y=1-x\) xuống pt dưới:
\(\sqrt{x+1-x}=x^2-\left(1-x\right)\)
\(\Leftrightarrow x^2+x-2=0\Rightarrow\left[{}\begin{matrix}x=1\Rightarrow y=0\\x=-2\Rightarrow y=3\end{matrix}\right.\)
d.
ĐKXĐ: \(x>-2;y>1;x+y>0\)
\(\left\{{}\begin{matrix}\sqrt{\dfrac{x+y}{x+2}}+\sqrt{\dfrac{x+y}{y-1}}=2\\2\left(x+y\right)^2=\left(x+2\right)^2+\left(y-1\right)^2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\sqrt{\dfrac{x+y}{x+2}}+\sqrt{\dfrac{x+y}{y-1}}=2\\\left(\dfrac{x+2}{x+y}\right)^2+\left(\dfrac{y-1}{x+y}\right)^2=2\end{matrix}\right.\)
Đặt \(\left\{{}\begin{matrix}\sqrt{\dfrac{x+y}{x+2}}=a>0\\\sqrt{\dfrac{x+y}{y-1}}=b>0\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}a+b=2\\\dfrac{1}{a^4}+\dfrac{1}{b^4}=2\end{matrix}\right.\)
Ta có: \(\dfrac{1}{a^4}+\dfrac{1}{b^4}\ge\dfrac{1}{8}\left(\dfrac{1}{a}+\dfrac{1}{b}\right)^4\ge\dfrac{1}{8}\left(\dfrac{4}{a+b}\right)^4=\dfrac{1}{8}.\left(\dfrac{4}{2}\right)^4=2\)
Dấu "=" xảy ra khi và chỉ khi \(a=b=1\)
\(\Rightarrow\left\{{}\begin{matrix}\dfrac{x+y}{x+2}=1\\\dfrac{x+y}{y-1}=1\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x=-1\\y=2\end{matrix}\right.\)

a)\(\left\{{}\begin{matrix}2x-3y=1\\x+2y=3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}2\cdot\left(3-2y\right)-3y=1\\x=3-2y\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}6-7y=1\\x=3-2y\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=\dfrac{5}{7}\\x=3-2\cdot\dfrac{5}{7}\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=\dfrac{5}{7}\\x=\dfrac{11}{7}\end{matrix}\right.\)b) Biểu diễn lại một biến theo một biến như pt trên rồi giải, ta có :
\(\left\{{}\begin{matrix}2x+4y=5\\4x-2y=2\end{matrix}\right.\Rightarrow\left\{{}\begin{matrix}x=\dfrac{9}{10}\\y=\dfrac{4}{5}\end{matrix}\right.\)
c) Cách làm tương tự như pt a ta có :
\(\left\{{}\begin{matrix}\dfrac{2}{3}x+\dfrac{1}{2}y=\dfrac{2}{3}\\\dfrac{1}{3}x-\dfrac{3}{4}y=\dfrac{1}{2}\end{matrix}\right.\Rightarrow\left\{{}\begin{matrix}x=\dfrac{9}{8}\\y=-\dfrac{1}{6}\end{matrix}\right.\)
d) Tương tự ta có :
\(\left\{{}\begin{matrix}0,3x-0,2y=0,5\\0,5x+0,4y=1,2\end{matrix}\right.\Rightarrow\left\{{}\begin{matrix}x=2\\y=\dfrac{1}{2}\end{matrix}\right.\)

5,\(hpt\Leftrightarrow\left\{{}\begin{matrix}x\left(x+y\right)\left(x+2\right)=0\\2\sqrt{x^2-2y-1}+\sqrt[3]{y^3-14}=x-2\end{matrix}\right.\)
Thay từng TH rồi làm nha bạn
3,\(hpt\Leftrightarrow\left\{{}\begin{matrix}x-y=\frac{1}{x}-\frac{1}{y}=\frac{y-x}{xy}\\2y=x^3+1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\left(x-y\right)\left(1+\frac{1}{xy}\right)=0\\2y=x^3+1\end{matrix}\right.\)
thay nhá
Bài 1:ĐKXĐ: \(2x\ge y;4\ge5x;2x-y+9\ge0\)\(\Rightarrow2x\ge y;x\le\frac{4}{5}\Rightarrow y\le\frac{8}{5}\)
PT(1) \(\Leftrightarrow\left(x-y-1\right)\left(2x-y+3\right)=0\)
+) Với y = x - 1 thay vào pt (2):
\(\frac{2}{3+\sqrt{x+1}}+\frac{2}{3+\sqrt{4-5x}}=\frac{9}{x+10}\) (ĐK: \(-1\le x\le\frac{4}{5}\))
Anh quy đồng lên đê, chắc cần vài con trâu đó:))
+) Với y = 2x + 3...
Bài 1:
Gọi thời gian làm riêng của đội 2 là x
Thời gian làm riêng của đội 1 là x+5
Trong 1 ngày, đội 2 làm được 1/x(công việc)
Trong 1 ngày, đội 1 làm được 1/(x+5)(công việc)
Theo đề, ta có phương trình:
\(\dfrac{1}{x}+\dfrac{1}{x+5}=\dfrac{1}{6}\)
\(\Leftrightarrow6\left(x+5\right)+6x=x\left(x+5\right)\)
\(\Leftrightarrow x^2+5x=12x+30\)
\(\Leftrightarrow x^2-7x-30=0\)
=>(x-10)(x+3)=0
=>x=10
Vậy: Thời gian làm riêng của đội 2 là 10 ngày
Thời gian làm riêng của đội 1 là 15 ngày