
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


ĐKXĐ: \(\left\{{}\begin{matrix}2x-3>=0\\x+1>=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x>=\dfrac{3}{2}\\x>=-1\end{matrix}\right.\)
=>\(x>=\dfrac{3}{2}\)
\(\sqrt{2x-3}-\sqrt{x+1}=x-4\)
=>\(\dfrac{2x-3-x-1}{\sqrt{2x-3}+\sqrt{x+1}}-\left(x-4\right)=0\)
=>\(\left(x-4\right)\left(\dfrac{1}{\sqrt{2x-3}+\sqrt{x+1}}-1\right)=0\)
=>x-4=0
=>x=4(nhận)

b) \(\sqrt{x^2}=\left|-8\right|\)
\(\Rightarrow\left|x\right|=8\)
\(\Rightarrow\left[{}\begin{matrix}x=8\\x=-8\end{matrix}\right.\)
d) \(\sqrt{9x^2}=\left|-12\right|\)
\(\Rightarrow\sqrt{\left(3x\right)^2}=12\)
\(\Rightarrow\left|3x\right|=12\)
\(\Rightarrow\left[{}\begin{matrix}3x=12\\3x=-12\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=\dfrac{12}{3}\\x=-\dfrac{12}{3}\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=4\\x=-4\end{matrix}\right.\)

Mình không thấy câu nào cả thì giúp kiểu gì lỗi ảnh hay sao ý

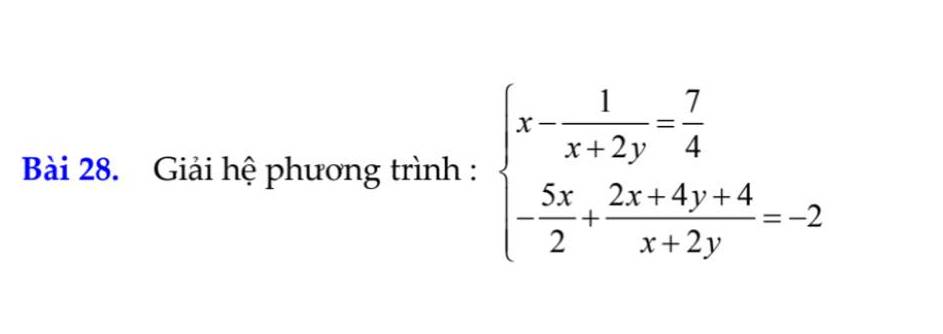
ĐKXĐ: \(x+2y\ne0\)
\(\left\{{}\begin{matrix}x-\dfrac{1}{x+2y}=\dfrac{7}{4}\\-\dfrac{5}{2}x+2+\dfrac{4}{x+2y}=-2\end{matrix}\right.\)
Đặt \(\dfrac{1}{x+2y}=z\) ta được hệ:
\(\left\{{}\begin{matrix}x-z=\dfrac{7}{4}\\-\dfrac{5}{2}x+4z=-4\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x=2\\z=\dfrac{1}{4}\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}x=2\\\dfrac{1}{x+2y}=\dfrac{1}{4}\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x=2\\x+2y=4\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}x=2\\y=1\end{matrix}\right.\)

Xét một phân số trong tổng:
\(\frac{1}{\sqrt{k} + \sqrt{k + 1}}\)
Nhân cả tử và mẫu với \(\sqrt{k + 1} - \sqrt{k}\), ta được:
\(\frac{1}{\sqrt{k} + \sqrt{k + 1}} = \frac{\sqrt{k + 1} - \sqrt{k}}{\left(\right. \sqrt{k} + \sqrt{k + 1} \left.\right) \left(\right. \sqrt{k + 1} - \sqrt{k} \left.\right)} = \sqrt{k + 1} - \sqrt{k}\)
Vậy:
\(A=\left(\right.\sqrt{2}-\sqrt{1}\left.\right)+\left(\right.\sqrt{3}-\sqrt{2}\left.\right)+\cdots+\left(\right.\sqrt{n + 1}-\sqrt{n}\left.\right)\)
Cộng các hạng tử lại, ta thấy \(\sqrt{2}\) ở số hạng đầu bị trừ đi ở số hạng sau, \(\sqrt{3}\) cũng vậy,… chỉ còn:
\(A = \sqrt{n + 1} - \sqrt{1} = \sqrt{n + 1} - 1\)
Đáp số: \(\sqrt{n + 1} - 1\)
Tham khảo

Gọi số xe dự định tham gia chở hàng là x (xe) với x>4, x nguyên dương
Mỗi xe dự định chở khối lượng hàng là: \(\dfrac{20}{x}\) (tấn)
Số xe thực tế tham gia chở hàng là: \(x-4\) (xe)
Thực tế mỗi xe phải chở số hàng là: \(\dfrac{20}{x-4}\) (tấn)
Do thực tế mỗi xe phải chở nhiều hơn dự định là 5/6 tấn hàng nên ta có pt:
\(\dfrac{20}{x-4}-\dfrac{20}{x}=\dfrac{5}{6}\)
\(\Rightarrow24x-24\left(x-4\right)=x\left(x-4\right)\)
\(\Leftrightarrow x^2-4x-96=0\)
\(\Rightarrow\left[{}\begin{matrix}x=12\\x=-8\left(loại\right)\end{matrix}\right.\)
Vậy thực tế có \(12-4=8\) xe tham gia vận chuyển


a) \(P=\left(\frac{\sqrt{x}}{\sqrt{x}-2}+\frac{1}{\sqrt{x}+2}-\frac{2}{4-x}\right):\frac{\sqrt{x}+3}{\sqrt{x}-2}\left(ĐK:x\ge0;x\ne4\right)\)
\(=\frac{\sqrt{x}\left(\sqrt{x}+2\right)+\sqrt{x}-2+2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}\cdot\frac{\sqrt{x}-2}{\sqrt{x}+3}\)
\(=\frac{x+2\sqrt{x}+\sqrt{x}}{\sqrt{x}+2}\cdot\frac{1}{\sqrt{x}+3}\)
\(=\frac{\sqrt{x}\left(\sqrt{x}+3\right)}{\sqrt{x}+2}\cdot\frac{1}{\sqrt{x}+3}=\frac{\sqrt{x}}{\sqrt{x}+2}\)
b) Vì: \(\sqrt{x}+4>0,\forall x\inĐK\)
=> \(2\sqrt{x}+4>\sqrt{x}\)
=> \(\frac{\sqrt{x}}{2\sqrt{x}+4}< 0\)
=> \(\frac{\sqrt{x}}{\sqrt{x}+2}< 2\)
=>đpcm
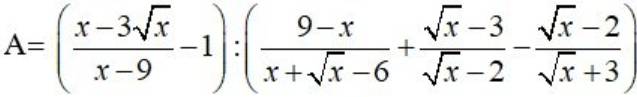






a: ĐKXĐ: \(\left\{{}\begin{matrix}x>=0\\x\notin\left\{4;9\right\}\end{matrix}\right.\)
\(A=\left(\dfrac{x-3\sqrt{x}}{x-9}-1\right):\left(\dfrac{9-x}{x+\sqrt{x}-6}+\dfrac{\sqrt{x}-3}{\sqrt{x}-2}-\dfrac{\sqrt{x}-2}{\sqrt{x}+3}\right)\)
\(=\left(\dfrac{\sqrt{x}\left(\sqrt{x}-3\right)}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}-1\right):\left(\dfrac{\left(3-\sqrt{x}\right)\left(3+\sqrt{x}\right)}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-2\right)}+\dfrac{\sqrt{x}-3}{\sqrt{x}-2}-\dfrac{\sqrt{x}-2}{\sqrt{x}+3}\right)\)
\(=\left(\dfrac{\sqrt{x}}{\sqrt{x}+3}-1\right):\left(\dfrac{3-x+\sqrt{x}-3}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-2\right)}+\dfrac{-\left(\sqrt{x}-2\right)}{\sqrt{x}+3}\right)\)
\(=\dfrac{\sqrt{x}-\sqrt{x}-3}{\sqrt{x}+3}:\dfrac{-\left(\sqrt{x}-2\right)}{\sqrt{x}+3}\)
\(=\dfrac{-3}{\sqrt{x}+3}\cdot\dfrac{\sqrt{x}+3}{-\left(\sqrt{x}-2\right)}=\dfrac{3}{\sqrt{x}-2}\)
b: A<1
=>A-1<0
=>\(\dfrac{3-\sqrt{x}+2}{\sqrt{x}-2}< 0\)
=>\(\dfrac{\sqrt{x}-5}{\sqrt{x}-2}>0\)
TH1: \(\left\{{}\begin{matrix}\sqrt{x}-5>0\\\sqrt{x}-2>0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}\sqrt{x}>5\\\sqrt{x}>2\end{matrix}\right.\Leftrightarrow\sqrt{x}>5\)
=>x>25
TH2: \(\left\{{}\begin{matrix}\sqrt{x}-5< 0\\\sqrt{x}-2< 0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}\sqrt{x}< 2\\\sqrt{x}< 5\end{matrix}\right.\)
=>\(\sqrt{x}< 2\)
=>0<=x<4