Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Lời giải của bạn Nhật Linh đúng rồi, tuy nhiên cần thêm điều kiện để A có nghĩa: \(x\ne\pm2\)

Bài 1:
a: ĐKXĐ: \(x\notin\left\{0;2;-2;3\right\}\)
\(A+\left(\dfrac{4x}{x+2}-\dfrac{8x^2}{\left(x+2\right)\left(x-2\right)}\right):\left(\dfrac{x-1}{x\left(x-2\right)}-\dfrac{2}{x}\right)\)
\(=\dfrac{4x^2-8x-8x^2}{\left(x+2\right)\left(x-2\right)}:\dfrac{x-1-2x+4}{x\left(x-2\right)}\)
\(=\dfrac{-4x\left(x+2\right)}{\left(x+2\right)\left(x-2\right)}\cdot\dfrac{x\left(x-2\right)}{-x+3}\)
\(=\dfrac{-4x}{-x+3}=\dfrac{4x}{x-3}\)
b: Để A<0 thi x/x-3<0
=>0<x<3

Bài 1:
a: \(A=\dfrac{x^4+x^3+x+1}{x^4-x^3+2x^2-x+1}=\dfrac{x^3\left(x+1\right)+\left(x+1\right)}{x^4-x^3+x^2+x^2-x+1}\)
\(=\dfrac{\left(x+1\right)\left(x^3+1\right)}{\left(x^2-x+1\right)\left(x^2+1\right)}=\dfrac{\left(x+1\right)^2}{x^2+1}\)
Để A=0 thì x+1=0
hay x=-1
b: \(B=\dfrac{x^4-5x^2+4}{x^4-10x^2+9}=\dfrac{\left(x^2-1\right)\left(x^2-4\right)}{\left(x^2-1\right)\left(x^2-9\right)}=\dfrac{x^2-4}{x^2-9}\)
Để B=0 thi (x-2)(x+2)=0
=>x=2 hoặc x=-2

B3;a,ĐKXĐ:\(x\ne\pm4\)
A=\(\left(\dfrac{4}{x-4}-\dfrac{4}{x+4}\right)\dfrac{x^2+8x+16}{32}=\left(\dfrac{4x+16}{x^2-16}-\dfrac{4x-16}{x^2-16}\right)\dfrac{x^2+2.4x+4^2}{32}=\left(\dfrac{4x+16-4x+16}{x^2-16}\right)\dfrac{\left(x+4\right)^2}{32}=\left(\dfrac{32}{x^2-16}\right)\dfrac{\left(x+4\right)^2}{32}=\dfrac{32\left(x+4\right)^2}{32.\left(x-4\right)\left(x+4\right)}=\dfrac{x+4}{x-4}\\ \\ \\ \\ \\ \\ b,Tacó\dfrac{x+4}{x-4}=\dfrac{1}{3}\Leftrightarrow3x+12=x-4\Leftrightarrow x=-8\left(TM\right)c,TAcó\dfrac{x+4}{x-4}=3\Leftrightarrow x+4=3x-12\Leftrightarrow x=8\left(TM\right)\)

b)\(\dfrac{x+14}{86}+\dfrac{x+15}{85}+\dfrac{x+16}{84}+\dfrac{x+17}{83}+\dfrac{x+116}{4}=0\)
\(\Leftrightarrow\dfrac{x+14}{86}+1+\dfrac{x+15}{85}+1+\dfrac{x+16}{84}+1+\dfrac{x+17}{83}+1+\dfrac{x+116}{4}-4=0\)
\(\Leftrightarrow\dfrac{x+100}{86}+\dfrac{x+100}{85}+\dfrac{x+100}{84}+\dfrac{x+100}{83}+\dfrac{x+100}{4}=0\)
\(\Leftrightarrow\left(x+100\right)\left(\dfrac{1}{86}+\dfrac{1}{85}+\dfrac{1}{84}+\dfrac{1}{83}+\dfrac{1}{4}\right)=0\)
\(\Leftrightarrow x+100=0\).Do \(\dfrac{1}{86}+\dfrac{1}{85}+\dfrac{1}{84}+\dfrac{1}{83}+\dfrac{1}{4}\ne0\)
\(\Leftrightarrow x=-100\)
c)\(\dfrac{1}{\left(x^2+5\right)\left(x^2+4\right)}+\dfrac{1}{\left(x^2+4\right)\left(x^2+3\right)}+\dfrac{1}{\left(x^2+3\right)\left(x^2+2\right)}+\dfrac{1}{\left(x^2+2\right)\left(x^2+1\right)}=-1\)
\(\Leftrightarrow\dfrac{1}{\left(x^2+1\right)\left(x^2+2\right)}+\dfrac{1}{\left(x^2+2\right)\left(x^2+3\right)}+...+\dfrac{1}{\left(x^2+4\right)\left(x^2+5\right)}=-1\)
\(\Leftrightarrow\dfrac{1}{x^2+1}-\dfrac{1}{x^2+2}+\dfrac{1}{x^2+2}-\dfrac{1}{x^2+3}+...+\dfrac{1}{x^2+4}-\dfrac{1}{x^2+5}=-1\)
\(\Leftrightarrow\dfrac{1}{x^2+1}-\dfrac{1}{x^2+5}=-1\)\(\Leftrightarrow\dfrac{4}{x^4+6x^2+5}=-1\)
\(\Leftrightarrow\dfrac{x^4+6x^2+9}{x^4+6x^2+5}=0\Leftrightarrow x^4+6x^2+9=0\)
\(\Leftrightarrow\left(x^2+3\right)^2>0\forall x\) (vô nghiệm)

a)Ta có: \(\left(\dfrac{1}{x-2}+\dfrac{2x}{x^2-4}+\dfrac{1}{x+2}\right)\left(\dfrac{2}{x}-1\right)\)
\(=\left(\dfrac{1}{x-2}+\dfrac{2x}{\left(x-2\right)\left(x+2\right)}+\dfrac{1}{x+2}\right)\left(\dfrac{2-x}{x}\right)\)
\(=\left(\dfrac{-\left(x-2\right)}{x\left(x-2\right)}+\dfrac{-2x\left(x-2\right)}{x\left(x-2\right)\left(x+2\right)}+\dfrac{2-x}{x\left(x+2\right)}\right)\)
\(=\left(-\dfrac{1}{x}+\dfrac{-2}{x+2}+\dfrac{2-x}{x\left(x+2\right)}\right)\)
\(=\left(\dfrac{-x-2-2x+2-x}{x\left(x+2\right)}\right)=\dfrac{-4}{x+2}\)
b) Ta có ĐKXĐ của A là \(x\ne\pm2\)
Lại có \(A=-\dfrac{4}{x+2}=1\)
\(\Rightarrow-4=x+2\Rightarrow x=-6\)
Vậy x=-6 thì A=1

A= \(\left(\dfrac{x}{x^2-4}+\dfrac{2}{2-x}+\dfrac{1}{x+2}\right):\left(x-2+\dfrac{10-x^2}{x+2}\right)\)
\(=\dfrac{-6}{\left(x+2\right)\left(x-2\right)}:\dfrac{6}{x+2}\)
\(=\dfrac{-1}{x-2}\)
b) \(\left|x\right|=\dfrac{1}{2}\)
\(\Rightarrow x=\dfrac{1}{2};x=\dfrac{-1}{2}\)
Thay \(x=\dfrac{1}{2}\) (Thỏa mãn ĐKXĐ \(x\ne2;x\ne-2\) )
\(A=\dfrac{-1}{\dfrac{1}{2}-2}=\dfrac{2}{3}\)
Thay \(x=\dfrac{-1}{2}\) (Thỏa mãn ĐKXĐ \(x\ne2;x\ne-2\) )
\(A=\dfrac{-1}{\dfrac{-1}{2}-2}=\dfrac{2}{5}\)
c) \(\dfrac{-1}{x-2}< 0\)
\(\Rightarrow x-2>0\)
\(\Leftrightarrow x>2\)
Vậy x > 2 để A < 0
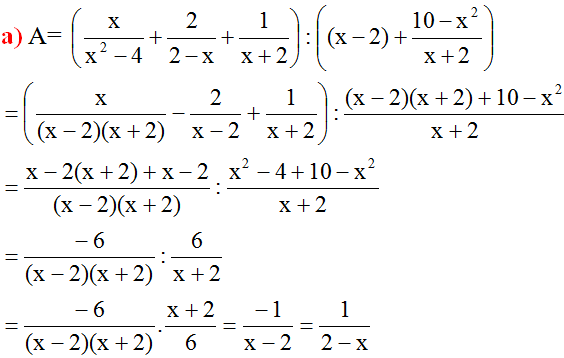

ĐKXĐ: x\(\ne\pm2\)
a, A=\(\left(\dfrac{x}{x^2-4}+\dfrac{2}{2-x}+\dfrac{1}{x+2}\right):\left(x-2+\dfrac{10-x^2}{x+2}\right)\)
= \(\left(\dfrac{x}{\left(x-2\right)\left(x+2\right)}-\dfrac{2\left(x+2\right)}{\left(x-2\right)\left(x+2\right)}+\dfrac{x-2}{\left(x-2\right)\left(x+2\right)}\right):\left(\dfrac{\left(x-2\right)\left(x+2\right)+10-x^2}{x+2}\right)\)
= \(\dfrac{x-2x-4+x-2}{\left(x-2\right)\left(x+2\right)}.\dfrac{x+2}{x^2-4+10-x^2}\)
= \(\dfrac{-6}{\left(x-2\right)\left(x+2\right)}.\dfrac{x+2}{6}\)
= \(\dfrac{-1}{x-2}\)
Vậy A=\(\dfrac{-1}{x-2}\) với x\(\ne\pm2\)
b, Để A<0 thì \(\dfrac{-1}{x-2}\)<0
<=> x-2>0 <=> x>2 (thỏa mãn ĐKXĐ)
Vậy để A<0 thì x>2