K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
NT
0
3 tháng 12 2018
Đợi nghĩ ra cách ngắn hơn nhá :))
\(1)\)\(B=x^{15}-8x^{14}+8x^{13}-8x^{12}+...-8x^2+8x-5\)
\(B=-7x^{15}+\left(8x^{15}-8x^{14}\right)+\left(8x^{13}-8x^{12}\right)+...+\left(8x^3-8x^2\right)+\left(8x-8\right)+3\)
\(B=-7x^{15}+8x^{14}\left(x-1\right)+8x^{12}\left(x-1\right)+...+8x^2\left(x-1\right)+8\left(x-1\right)+3\)
\(B=-7x^{15}+8\left(x-1\right)\left(x^{14}+x^{12}+...+x^2+1\right)+3\)
\(B=-7x^{15}+8\left(x-1\right)\left[x^{12}\left(x^2+1\right)+x^8\left(x^2+1\right)+...+\left(x^2+1\right)\right]+3\)
\(B=-7x^{15}+8\left(x-1\right)\left(x^2+1\right)\left(x^{12}+x^8+...+1\right)+3\)
\(B=-7x^{15}+8\left(x-1\right)\left(x^2+1\right)\left[x^8\left(x^4+1\right)+\left(x^4+1\right)\right]+3\)
\(x=7\)\(\Rightarrow\)\(x+1=8\)
\(B=-7x^{15}+\left(x+1\right)\left(x-1\right)\left(x^2+1\right)\left(x^4+1\right)\left(x^8+1\right)+3\)
\(B=-7x^{15}+\left(x^2-1\right)\left(x^2+1\right)\left(x^4+1\right)\left(x^8+1\right)\)
\(B=-7x^{15}+\left(x^4-1\right)\left(x^4+1\right)\left(x^8+1\right)\)
\(B=-7x^{15}+\left(x^8-1\right)\left(x^8+1\right)=-7x^{15}+x^{16}-1=x^{15}\left(x-7\right)-1=-1\)
...
19 tháng 4 2018
Bài 1:
ta có: x=7 => x+ 1 =8
thay vào biểu thức B
\(\Rightarrow B=x^{15}-\left(x+1\right)x^{14}+\left(x+1\right)x^{13}-\left(x+1\right)x^{12}+...-\left(x+1\right)x^2+\left(x+1\right)x-5\) \(B=x^{15}-x^{15}-x^{14}+x^{14}+x^{13}-x^{13}-x^{12}+...-x^3-x^2+x^2+x-5\)
\(B=x-5\)
\(B=7-5\)
\(B=2\)
Bài 2:
bn tham khảo link dưới nha:
https://olm.vn/hoi-dap/question/982834.html
Bài 3: Bn xem lại giúp mk nha!!! ( Chỗ nếu: thì....)
5 tháng 9 2018
\(B=x^{15}-8x^{14}+8x^{13}-8x^{12}+...+8x-5\)
\(=x^{15}-\left(x+1\right)x^{14}+\left(x+1\right)x^{13}-\left(x+1\right)x^{12}+...+\left(x+1\right)x-x+2\)
\(=x^{15}-x^{15}-x^{14}+x^{14}+x^{13}-x^{13}-x^{12}+...+x^2+x-x+2\)
\(=2\)
SM
19 tháng 9 2018
Bài 1 : Thay 8 = x + 1 vào B
=> B = x15 - ( x + 1 ) x14 + ( x + 1 ) x13 - ( x + 1 ) x12 ......+ ( x + 1 ) x - 5
= x15 - x15 - x14 + x14 + x13 - x13 ...... - x2 + x2 + x - 5
= x - 5
Mà x = 7
=> B = 7 - 5 = 2
Vậy B = 1
2 ) Gọi ba số cần tìm là a; a+1; a+2
Vì tích hai số đầu nhỏ hơn tích hai số sau là 50
=> a ( a + 1 ) = ( a + 1 ) ( a + 2 ) - 50
=> a2 + a = a2 + 3a + 2 - 50
=> a = 3a - 48
=> 2a = 48
=> a = 24
Vậy 3 số phải tìm là 24; 25; 26
Bài 3 đề bài chưa rõ nếu cái gì ? Bạn sửa lại đi, mình sẽ giải
19 tháng 9 2020
1.B = x15 - 8x14 + 8x13 - 8x2 + ... - 8x2 + 8x - 5
B = x^15 - 7x^14 -x^14+7x^13+x^13-7x^12-...-x^2+7x+x-5
B = x^14(x-7) - x^14(x-7) +...+x^2(x-7)-x(x-7)+x-5
B = 7-5=2
2.Gọi 3 số cần tìm là theo thứ tự a,b,c
Ta có:
b.c - a.b = 50
=> b.(c-a) = 50
Vì là 3 số tự nhiên liên tiếp
=> khoảng cách giữa a và c là 2
Ta có: 50 = 2 . 25
=> b = 25
=> a = 25 - 1 = 24
=> c = 25 + 1 = 26
Vậy 3 số đó là: 24;25;26 cần tìm
3.(a+b)^2=2(a^2+b^2)
<=> a^2+b^2+2ab=2a^2+2b^2
<=> a^2+b^2=2ab
<=>a^2+b^2-2ab=0
<=>(a-b)^2=0
=> a-b=0
<=>a=b(đpcm)
19 tháng 9 2020
Câu 3 thiếu đề nhé, đề đúng là: CMR nếu: \(\frac{x}{a}=\frac{y}{b}=\frac{z}{c}\)thì \(\left(x^2+y^2+z^2\right)\left(a^2+b^2+c^2\right)=\left(ax+by+cz\right)^2\)
Giải
Ta có: \(\left(x^2+y^2+z^2\right)\left(a^2+b^2+c^2\right)-\left(ax+by+cz\right)^2\)
\(=x^2a^2+x^2b^2+x^2c^2+y^2a^2+y^2b^2+y^2c^2+z^2a^2+z^2b^2+z^2c^2\)\(-a^2x^2-b^2y^2-c^2z^2-2axby-2bycz-2axcz\)
\(=x^2b^2+x^2c^2+y^2a^2+y^2c^2+z^2a^2+z^2b^2-2axby-2bycz-2axcz\)
\(=x^2b^2-2xbay+a^2y^2+y^2c^2-2ycbz+z^2b^2+x^2c^2-2cxaz+a^2z^2\)
\(=\left(xb-ay\right)^2+\left(yc-zb\right)^2+\left(xc-az\right)^2\)
Lại có: \(\frac{x}{a}=\frac{y}{b}=\frac{z}{c}\Rightarrow\hept{\begin{cases}bx=ay\\yc=bz\\cx=az\end{cases}}\)
\(\Rightarrow\left(bx-ay\right)^2+\left(yc-bz\right)^2+\left(cx-az\right)^2=\left(ay-ay\right)^2+\left(bz-bz\right)^2+\left(az-az\right)^2=0\)
\(\Rightarrow\left(x^2+y^2+z^2\right)\left(a^2+b^2+c^2\right)-\left(ax+by+cz\right)^2=0\)
\(\Rightarrow\left(x^2+y^2+z^2\right)\left(a^2+b^2+c^2\right)=\left(ax+by+cz\right)^2\)
=> Đpcm
P/s: Nãy đánh xoq rồi tự nhiên olm lỗi, không gửi được, giờ đánh lại T.T Tức~
NC
28 tháng 8 2020
a) Ta có: \(\left(3x+5\right)^2-\left(x+3\right)^2-8x\left(x+3\right)=12\)
\(\Leftrightarrow9x^2+30x+25-x^2-6x-9-8x^2-24x-12=0\)
\(\Leftrightarrow4=0\) (vô lý)
=> pt vô nghiệm
b) \(\left(2x-5\right)^2-\left(x-2\right)^2-\left(x-1\right)\left(3x+2\right)=8\)
\(\Leftrightarrow4x^2-20x+25-x^2+4x-4-3x^2+x+2-8=0\)
\(\Leftrightarrow-15x=-13\)
\(\Rightarrow x=\frac{13}{15}\)
NC
28 tháng 8 2020
c) \(-2x\left(x+3\right)+\left(2x-5\right)^2=-3\left(x+2\right)\)
\(\Leftrightarrow-2x^2-6x+4x^2-20x+25+3x+6=0\)
\(\Leftrightarrow2x^2-23x+31=0\)
\(\Leftrightarrow2\left(x^2-\frac{23}{2}x+\frac{529}{16}\right)-\frac{281}{8}=0\)
\(\Leftrightarrow\left(x-\frac{23}{4}\right)^2-\left(\frac{\sqrt{281}}{4}\right)^2=0\)
\(\Leftrightarrow\left(x-\frac{23+\sqrt{281}}{4}\right)\left(x-\frac{23-\sqrt{281}}{4}\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x-\frac{23+\sqrt{281}}{4}=0\\x-\frac{23-\sqrt{281}}{4}=0\end{cases}}\Rightarrow\orbr{\begin{cases}x=\frac{23+\sqrt{281}}{4}\\x=\frac{23-\sqrt{281}}{4}\end{cases}}\)
BB
2
KN
2 tháng 12 2019
3x^2-2x+1 3x^4-8x^3-10x^2+8x-5 x^2-2x-16/3 3x^4-2x^3+x^2 -6x^3-12x^2+8x-5 -6x^3+4x^2-2x -16x^2+10x-5 -16x^2+32/3x-16/3 -2/3x+1/3
Vậy
- (3x4-8x3-10x2+8x-5):(3x2-2x+1) = \(x^2-2x-\frac{16}{3}\)dư \(\frac{-2}{3}x+\frac{1}{3}\)
KN
2 tháng 12 2019
x^2-1 x^4-2x^3+2x-1 x^2-2x+1 x^4-x^2 -2x^3+x^2+2x-1 -2x^3+2x x^2-1 x^2-1 0
8 tháng 7 2019
Đề là phân tích đa thức thành nhân tử hả bn
\(8x^2y^2-12y^3+16x^2\)
\(4\left(2x^2y^2-3y^3+4x^2\right)\)
Tích giúp mình nka ^^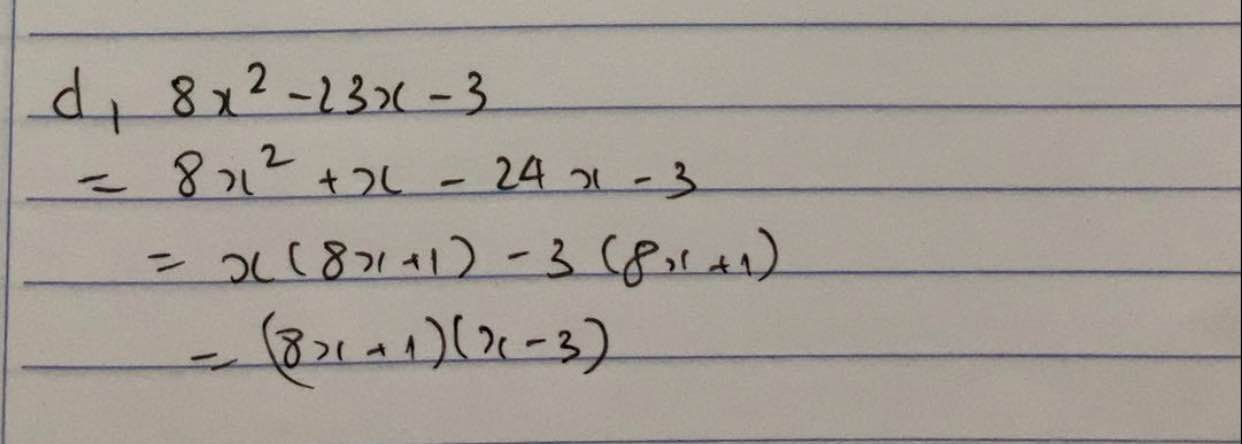
`8x^2-23x-3`
`=8x^2-24x+x-3`
`=8x(x-3)+(x-3)`
`=(8x+1)(x-3)`