K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
NT
25 tháng 8 2021
\(\sqrt{12+2\sqrt{35}}=\sqrt{7+2\sqrt{7.5}+5}=\sqrt{\left(\sqrt{7}+\sqrt{5}\right)^2}=\sqrt{7}+\sqrt{5}\)
24 tháng 9 2016
Cho mình sửa đề xí ạ!
b) \(\frac{\sqrt{10}+\sqrt{15}}{\sqrt{8}+\sqrt{12}}\)
10 tháng 3 2022
Để hệ pt có nghiệm duy nhât \(\dfrac{a+1}{1}\ne\dfrac{-1}{a-1}\Leftrightarrow a^2-1\ne-1\Leftrightarrow a^2\ne0\Leftrightarrow a\ne0\)
\(\left\{{}\begin{matrix}\left(a^2-1\right)x-\left(a-1\right)y=a^2-1\\x+\left(a-1\right)y=2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}a^2x=a^2+1\\y=\dfrac{2-x}{a-1}\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{a^2+1}{a^2}\\y=\dfrac{2-\dfrac{a^2+1}{a^2}}{a-1}=\dfrac{\dfrac{a^2-1}{a^2}}{a-1}=\dfrac{\left(a^2-1\right)\left(a-1\right)}{a^2}\end{matrix}\right.\)
Ta có \(\dfrac{a^2+1}{a^2}-\dfrac{\left(a^2-1\right)\left(a-1\right)}{a^2}=0\)
\(\Leftrightarrow a^2+1-a^3+a^2+a-1=0\)
\(\Leftrightarrow-a^3+2a^2+a=0\Leftrightarrow a^2-2a-1=0\)
\(\Leftrightarrow\left(a-1\right)^2-2=0\Leftrightarrow\left(a-1-\sqrt{2}\right)\left(a-1+\sqrt{2}\right)=0\Leftrightarrow a=1\pm\sqrt{2}\)
22 tháng 6 2023
8:
BC=BH+CH=64+81=145cm
\(AB=\sqrt{BH\cdot BC}=8\sqrt{145}\left(cm\right)\)
\(AC=\sqrt{81\cdot145}=9\sqrt{145}\left(cm\right)\)
tan C=AB/AC=8/9
=>góc C=42 độ
=>góc B=48 độ
16 tháng 7 2021
a) Xét tứ giác ABOC có
\(\widehat{ABO}+\widehat{ACO}=180^0\)
nên ABOC là tứ giác nội tiếp(Dấu hiệu nhận biết tứ giác nội tiếp)
13 tháng 10 2021
Bài 9:
b: Tọa độ giao điểm là:
\(\left\{{}\begin{matrix}2x-3=-3x+7\\y=2x-3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=2\\y=1\end{matrix}\right.\)
24 tháng 8 2021
Bài 8:
a: Ta có: \(\sqrt{4x}=\sqrt{5}\)
\(\Leftrightarrow4x=5\)
hay \(x=\dfrac{5}{4}\)
b: Ta có: \(\sqrt{4\cdot\left(1-x\right)^2}-6=0\)
\(\Leftrightarrow2\left|x-1\right|=6\)
\(\Leftrightarrow\left|x-1\right|=3\)
\(\Leftrightarrow\left[{}\begin{matrix}x-1=3\\x-1=-3\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=4\\x=-2\end{matrix}\right.\)
c: Ta có: \(\sqrt{2x-3}=\sqrt{7}\)
\(\Leftrightarrow2x-3=7\)
hay x=5
d: Ta có: \(\sqrt{\left(3x-2\right)^2}=4\)
\(\Leftrightarrow\left|3x-2\right|=4\)
\(\Leftrightarrow\left[{}\begin{matrix}3x-2=4\\3x-2=-4\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}3x=6\\3x=-2\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-\dfrac{2}{3}\end{matrix}\right.\)
3 tháng 2 2022
\(\dfrac{\sqrt{15}-\sqrt{6}}{\sqrt{35}-\sqrt{14}}=\dfrac{\sqrt{3}\left(\sqrt{5}-\sqrt{2}\right)}{\sqrt{7}\left(\sqrt{5}-\sqrt{2}\right)}=\dfrac{\sqrt{21}}{7}\)
15 tháng 8 2021
đoạn cuối thiếu dấu"+"
\(A=\dfrac{\sqrt{4}-\sqrt{5}}{4-5}+\dfrac{\sqrt{5}-\sqrt{6}}{5-6}+....+\dfrac{\sqrt{34}-\sqrt{35}}{34-35}+\dfrac{\sqrt{35}-\sqrt{36}}{335-36}\)
\(A=\dfrac{\sqrt{4}-\sqrt{5}+\sqrt{5}-\sqrt{6}+....+\sqrt{35}-\sqrt{36}}{-1}=\dfrac{\sqrt{4}-\sqrt{36}}{-1}\)
\(A=\sqrt{36}-\sqrt{4}=6-2=4\)
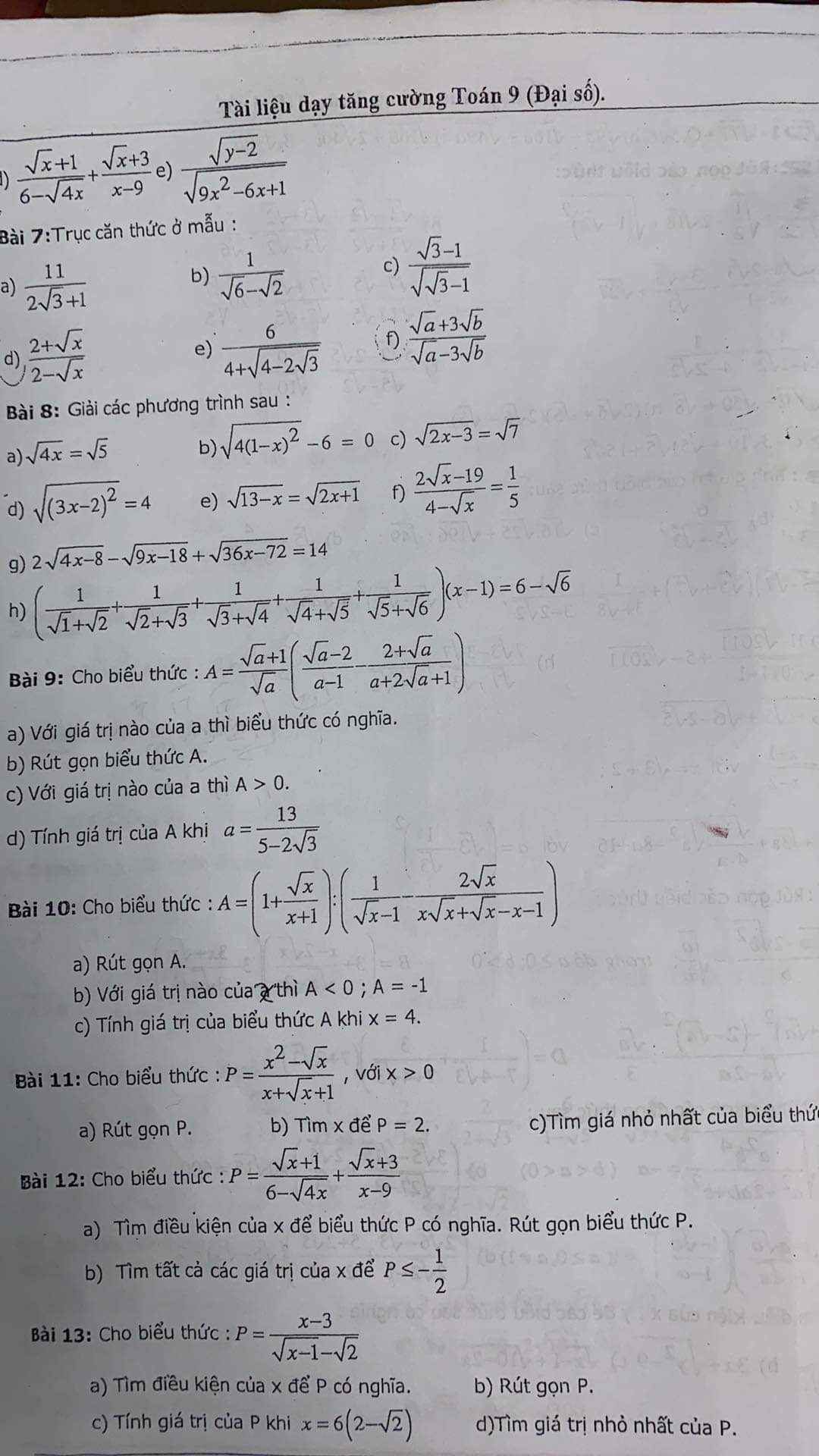
Sửa đề: \(\sqrt{8-\sqrt{15}}\)
\(=\dfrac{1}{\sqrt{2}}\cdot\sqrt{16-2\sqrt{15}}\)
\(=\dfrac{1}{\sqrt{2}}\left(\sqrt{15}-1\right)=\dfrac{\sqrt{15}-1}{\sqrt{2}}=\dfrac{\sqrt{30}-\sqrt{2}}{2}\)