Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

1.
\(3cos2x-7=2m\)
\(\Leftrightarrow cos2x=\dfrac{2m-7}{3}\)
Phương trình đã cho có nghiệm khi:
\(-1\le\dfrac{2m-7}{3}\le1\)
\(\Leftrightarrow2\le m\le5\)
2.
\(2cos^2x-\sqrt{3}cosx=0\)
\(\Leftrightarrow cosx\left(2cosx-\sqrt{3}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx=0\\cosx=\dfrac{\sqrt{3}}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{2}+k\pi\\x=\pm\dfrac{\pi}{6}+k2\pi\end{matrix}\right.\)
\(\Rightarrow\) Có 4 nghiệm \(\dfrac{\pi}{2};\dfrac{3\pi}{2};\dfrac{\pi}{6};\dfrac{11\pi}{6}\) thuộc đoạn \(\left[0;2\pi\right]\)

1.
\(\Leftrightarrow1-2sin^2x+sinx+m=0\)
\(\Leftrightarrow2sin^2x-sinx-1=m\)
Đặt \(sinx=t\Rightarrow t\in\left[-\dfrac{1}{2};\dfrac{\sqrt{2}}{2}\right]\)
Xét hàm \(f\left(t\right)=2t^2-t-1\) trên \(\left[-\dfrac{1}{2};\dfrac{\sqrt{2}}{2}\right]\)
\(-\dfrac{b}{2a}=\dfrac{1}{4}\in\left[-\dfrac{1}{2};\dfrac{\sqrt{2}}{2}\right]\)
\(f\left(-\dfrac{1}{2}\right)=0\) ; \(f\left(\dfrac{1}{4}\right)=-\dfrac{9}{8}\) ; \(f\left(\dfrac{\sqrt{2}}{2}\right)=-\dfrac{\sqrt{2}}{2}\)
\(\Rightarrow-\dfrac{9}{8}\le f\left(t\right)\le0\Rightarrow-\dfrac{9}{8}\le m\le0\)
Có 2 giá trị nguyên của m (nếu đáp án là 3 thì đáp án sai)
2.
ĐKXĐ: \(sin2x\ne1\Rightarrow x\ne\dfrac{\pi}{4}\) (chỉ quan tâm trong khoảng xét)
Pt tương đương:
\(\left(tan^2x+cot^2x+2\right)-\left(tanx+cotx\right)-4=0\)
\(\Leftrightarrow\left(tanx+cotx\right)^2+\left(tanx+cotx\right)-4=0\)
\(\Rightarrow\left[{}\begin{matrix}tanx+cotx=\dfrac{1+\sqrt{17}}{2}\\tanx+cotx=\dfrac{1-\sqrt{17}}{2}\left(loại\right)\end{matrix}\right.\)
Nghiệm xấu quá, kiểm tra lại đề chỗ \(-tanx+...-cotx\) có thể 1 trong 2 cái đằng trước phải là dấu "+"


Đặt t = sin x + cos x − 2 ≤ t ≤ 2 ⇒ sin x cos x = t 2 − 1 2 .
Phương trình trở thành t 2 − 1 2 − t + m = 0 ⇔ − 2 m = t 2 − 2 t − 1 ⇔ t − 1 2 = − 2 m + 2 .
Do − 2 ≤ t ≤ 2 ⇒ − 2 − 1 ≤ t − 1 ≤ 2 − 1 ⇔ 0 ≤ t − 1 2 ≤ 3 + 2 2 .
Vậy để phương trình có nghiệm
⇔ 0 ≤ − 2 m + 2 ≤ 3 + 2 2 ⇔ − 1 + 2 2 2 ≤ m ≤ 1 → m ∈ ℤ m ∈ − 1 ; 0 ; 1 .
Chọn đáp án C.

\(\left\{{}\begin{matrix}9-8m>0\\9-5m>0\end{matrix}\right.\) \(\Rightarrow m< \dfrac{9}{8}\)
Gọi a là nghiệm chung của 2 pt
\(\Rightarrow\left\{{}\begin{matrix}a^2+3a+2m=0\\a^2+6a+5m=0\end{matrix}\right.\)
\(\Rightarrow3a+3m=0\Rightarrow a=-m\)
Thay vào 2 pt ban đầu:
\(\Rightarrow\left\{{}\begin{matrix}m^2-3m+2m=0\\m^2-6m+5m=0\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}m=0\\m=1\end{matrix}\right.\)

Nếu phương trình là \(\left(2m^2-5m+2\right)\left(x-1\right)^{2021}\left(x^{2020}-2\right)+2x^2-3=0\) thì còn có cơ hội giải quyết
Chứ đề đúng thế này thì e rằng không có cơ hội nào cả.
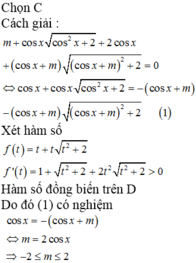
14) đề bài tương đương với
cotx = -\(\sqrt{3}\Leftrightarrow cotx=cot\left(\frac{-\pi}{6}\right)\Leftrightarrow x=\frac{-\pi}{6}+k\pi\)
16) đề bài tương đương với
cosx=2m-1
ta có cosx \(\in\left[-1;1\right]\Leftrightarrow-1\le2m-1\le1\\ \Leftrightarrow\left\{{}\begin{matrix}2m-1\ge-1\\2m-1\le1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}m\ge0\\m\le1\end{matrix}\right.\)
vì n là số nguyên nên m=0 hoặc m=1