1.17 ạ
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
27 tháng 12 2021
b: Tọa độ giao điểm là:
\(\left\{{}\begin{matrix}\dfrac{2}{3}x=-\dfrac{1}{3}x+2\\y=\dfrac{2}{3}x\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=2\\y=\dfrac{4}{3}\end{matrix}\right.\)
NP
0
TM
0
12 tháng 10 2021
Câu 2:
Ta có: \(\sqrt{x^2-4x+4}=x-1\)
\(\Leftrightarrow2-x=x-1\left(x< 2\right)\)
\(\Leftrightarrow-2x=-3\)
hay \(x=\dfrac{3}{2}\left(tm\right)\)
NC
4
1 tháng 11 2021
\(11,\\ a,=4\cdot5+14:7=20+2=22\\ b,=3\sqrt{2}-12\sqrt{2}+5\sqrt{2}=-4\sqrt{2}\\ c,=\dfrac{3-\sqrt{2}+3+\sqrt{2}}{\left(3-\sqrt{2}\right)\left(3+\sqrt{2}\right)}=\dfrac{6}{7}\\ 12,\\ a,P=\dfrac{\sqrt{x}+3+\sqrt{x}-3}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}\cdot\dfrac{\sqrt{x}-3}{\sqrt{x}}\\ P=\dfrac{2\sqrt{x}}{\sqrt{x}\left(\sqrt{x}+3\right)}=\dfrac{2}{\sqrt{x}+3}\\ b,P=\dfrac{1}{2}\Leftrightarrow\sqrt{x}+3=4\Leftrightarrow x=1\left(tm\right)\)
1 tháng 11 2021
a: \(=4\cdot5+14:7=20+2=22\)
b: \(=3\sqrt{2}-8\sqrt{2}+5\sqrt{2}=0\)
TB
1
29 tháng 7 2023
1:
a: Khi m=1 thì (1) sẽ là x^2+2x-5=0
=>\(x=-1\pm\sqrt{6}\)
b: Δ=(2m)^2-4(-2m-3)
=4m^2+8m+12
=4m^2+8m+4+8=(2m+2)^2+8>=8>0
=>Phương trình luôn có hai nghiệm phân biệt
2:
Thay x=-1 và y=2 vào (P), ta được:
a*(-1)^2=2
=>a=2
NT
1
22 tháng 8 2021
Với \(x\ge0;x\ne\pm16\)
\(B=\left(\frac{\sqrt{x}}{\sqrt{x}+4}+\frac{4}{\sqrt{x}-4}\right):\frac{x+16}{\sqrt{x}+2}\)
\(=\left(\frac{x-4\sqrt{x}+4\sqrt{x}+16}{x-16}\right):\frac{x+16}{\sqrt{x}-2}=\frac{\sqrt{x}-2}{x-16}\)
V
0
T
0
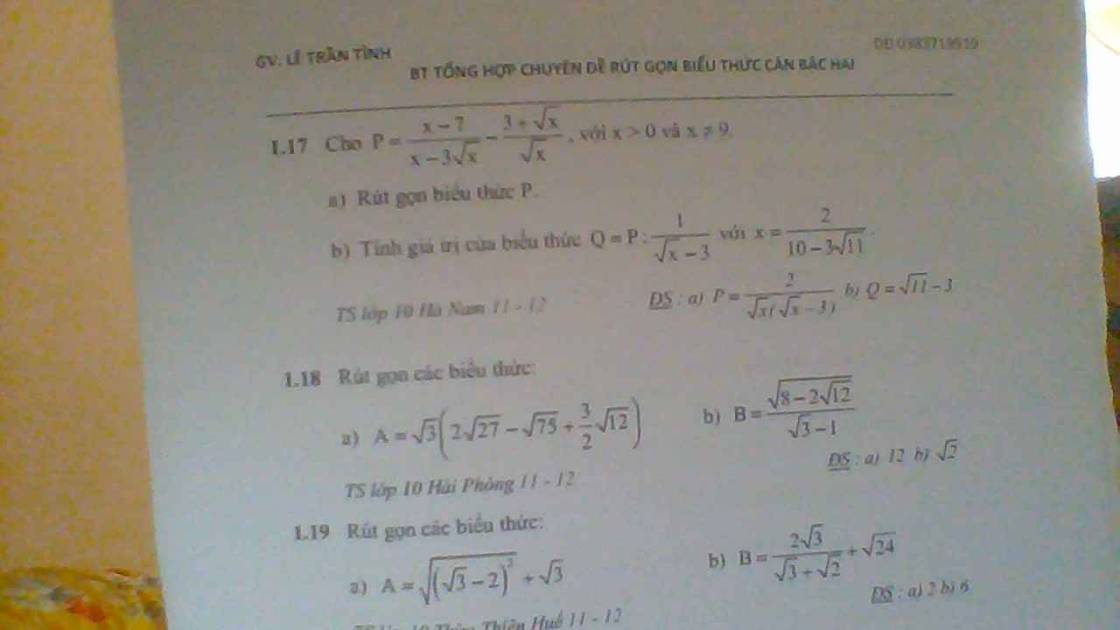1.17:
a: \(P=\dfrac{x-7}{\sqrt{x}\left(\sqrt{x}-3\right)}-\dfrac{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}{\sqrt{x}\left(\sqrt{x}-3\right)}\)
\(=\dfrac{x-7-x+9}{\sqrt{x}\left(\sqrt{x}-3\right)}=\dfrac{2}{\sqrt{x}\left(\sqrt{x}-3\right)}\)
b: \(Q=P:\dfrac{1}{\sqrt{x}-3}=\dfrac{2}{\sqrt{x}\left(\sqrt{x}-3\right)}\cdot\dfrac{\sqrt{x}-3}{1}=\dfrac{2}{\sqrt{x}}\)
\(x=\dfrac{2}{10-3\sqrt{11}}=\dfrac{2\left(10+3\sqrt{11}\right)}{100-99}=20+6\sqrt{11}=\left(\sqrt{11}+3\right)^2\)
Khi x=(căn 11+3)^2 thì \(Q=\dfrac{2}{\sqrt{11}+3}=\sqrt{11}-3\)