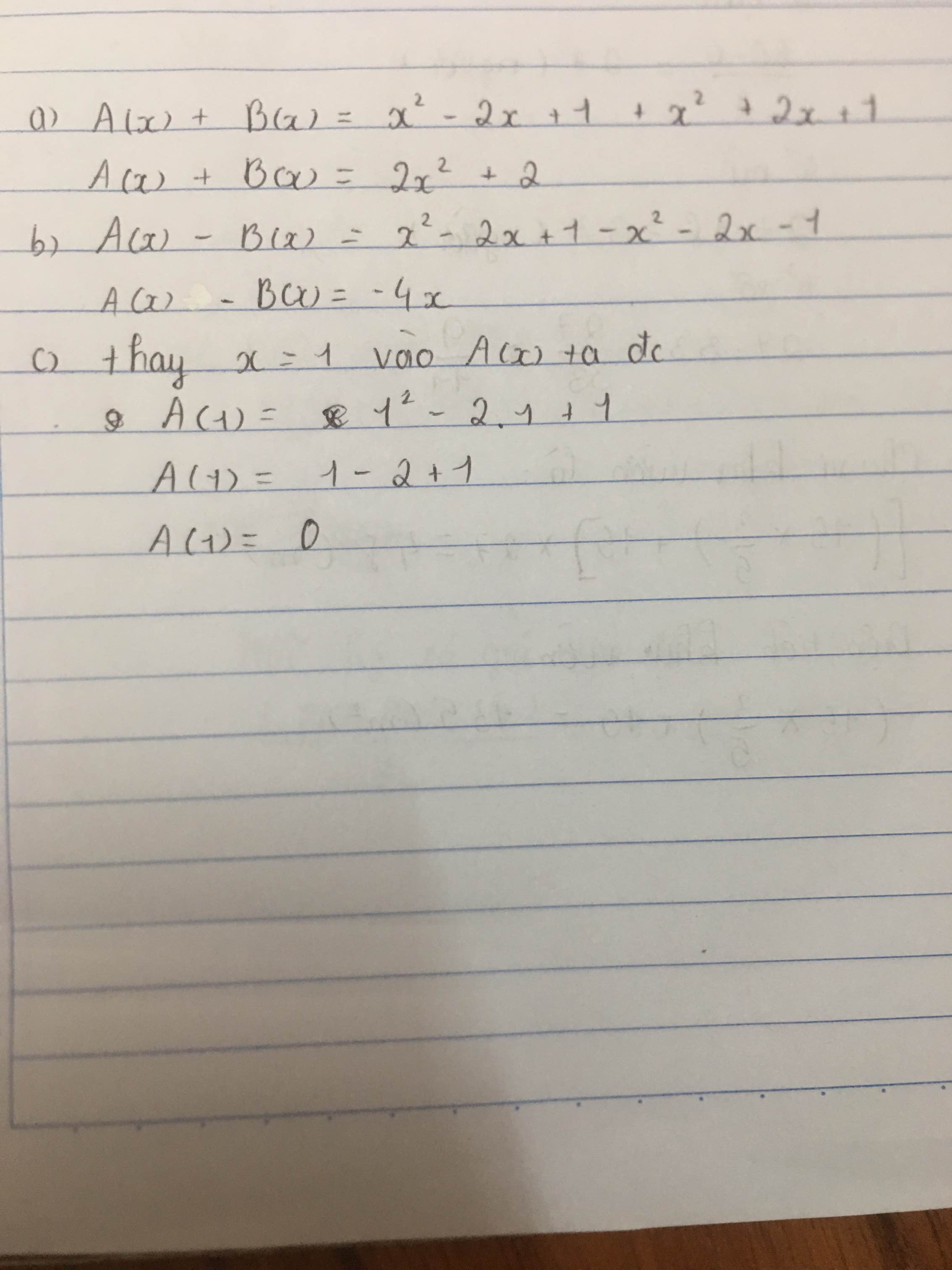K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
3 tháng 8 2021
A(x)+B(x)=2x-3x3+2x2+1+4x3+2x2-5
= x3+4x2+2x-4
thay x=1 vào B(x) ta được
B(x)=4.13+2.13-5
=4+2-5
=1
3 tháng 8 2021
\(A\left(x\right)+B\left(x\right)=\left(x+2\right)\left(x^2+2x-2\right)\)
thay x=1 \(=>A\left(1\right)+B\left(1\right)=3\left(1+2-2\right)=3\)
T
8 tháng 9 2023
\(a,A=x^3+3x^2-4x-12\)
\(=x^2\left(x+3\right)-4\left(x+3\right)\)
\(=\left(x^2-4\right)\left(x+3\right)\)
\(=\left(x-2\right)\left(x+2\right)\left(x+3\right)\)
Thay \(x=2\) vào A, ta được:
\(A=\left(2-2\right)\left(2+2\right)\left(2+3\right)\)
\(=0\)
⇒ \(x=2\) là nghiệm của A
\(B=-2x^3+3x^2+4x+1\)
Thay \(x=2\) vào B, ta được:
\(B=-2\cdot2^3+3\cdot2^2+4\cdot2+1\)
\(=-16+12+8+1\)
\(=5\)
⇒ \(x=2\) không là nghiệm của B
\(b,A+B=x^3+3x^2-4x-12+\left(-2x^3\right)+3x^2+4x+1\)
\(=\left[x^3+\left(-2x^3\right)\right]+\left(3x^2+3x^2\right)+\left(-4x+4x\right)+\left(-12+1\right)\)
\(=-x^3+6x^2-11\)
\(A-B=x^3+3x^2-4x-12-\left(-2x^3+3x^2+4x+1\right)\)
\(=x^3+3x^2-4x-12+2x^3-3x^2-4x-1\)
\(=\left(x^3 +2x^3\right)+\left(3x^2-3x^2\right)+\left(-4x-4x\right)+\left(-12-1\right)\)
\(=3x^3-8x-13\)
#\(Toru \)
9 tháng 4 2016
Chiều rộng là : 15 : ( 5 - 3 ) x 3 = 22,5 m
Chiều dài là : 15 + 22,5 = 37,5 m
Chu vi là : ( 37,5 + 22,5 ) x 2 = 120 m
Diện tích là : 37,5 x 22,5 = 843,75 m2
9 tháng 4 2016
Ta có: (a+b-c)/c=(b+c-a)/a=(c+a-b)/b=(a+b-c+b+c... (a+b+c)=(a+b+c)/(a+b+c)=1
=>(a+b-c)/c=1 => a+b-c=c =>a+b=2c (1)
Tương tự: (b+c-a)/a=1 =>b+c=2a (2)
(c+a-b)/b=1 =>c+a=2b (3)
Thay (1), (2), (3) vào P, ta có:
P=(a+b)/a . (b+c)/b .(a+c)/c=2c/a.2a/b.2b/c=2.2.2=8. Hết nhưng sách thì chia ra hai trường hợp như sau:
Từ giả thiết, suy ra:
(a+b-c)/c+2=(b+c-a)/a+2=(c+a-b)/b+2
<=> (a+b+c)/c=(b+c+a)/a=(c+a+b)/b
Xét 2 trường hợp:
Nếu a+b+c=0 => (a+b)/a.(b+c)/b.(c+a)/c=((-c)(-a)(-b))/a...
Nếu a+b+c khác 0 =>a=b=c =>P=2.2.2=8
HN
5 tháng 4 2022
a) M=(x3-x2-2x+1)+(-x3+x2)=x3-x2-2x+1-x3+x2=-2x+1.
b) Với x=1, M=-2.1+1=-1.
c) M=0 \(\Leftrightarrow\) -2x+1=0 \(\Leftrightarrow\) x=1/2.
LM
Lê Minh Vũ
CTVHS
19 tháng 6 2023
a) Ta có: \(A\left(x\right)=ax^2+bx+c\)
Thay \(A\left(-1\right)\) ta được:
\(A\left(-1\right)=a\left(-1\right)^2+b\left(-1\right)+c=a+c-b\)
\(=b-8-b=-8\)
b) \(\left\{{}\begin{matrix}A\left(0\right)=4\\A\left(1\right)=9\\A\left(2\right)=14\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}c=4\\a+b+c=9\\4a+2b+c=14\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}c=4\\a+b=5\\4a+2b=10\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}c=4\\a+b=5\\2a+b=5\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}c=4\\a=0\\b=5\end{matrix}\right.\)
c)
Ta có: \(\left\{{}\begin{matrix}A\left(2\right)=4a+2b+c\\A\left(-1\right)=a-b+c\end{matrix}\right.\)
\(\Leftrightarrow A\left(2\right)+A\left(-1\right)=5a+b+2c=0\)
\(\Leftrightarrow A\left(2\right)=-A\left(-1\right)\)
\(\Leftrightarrow A\left(2\right)\times A\left(-1\right)=-\left[A\left(2\right)\right]^2\le0\)
16 tháng 8 2023
a) Ta có A = 21 + 22 + 23 + ... + 22022
2A = 22 + 23 + 24 + ... + 22023
2A - A = ( 22 + 23 + 24 + ... + 22023 ) - ( 21 + 22 + 23 + ... + 22022 )
A = 22023 - 2
Lại có B = 5 + 52 + 53 + ... + 52022
5B = 52 + 53 + 54 + ... + 52023
5B - B = ( 52 + 53 + 54 + ... + 52023 ) - ( 5 + 52 + 53 + ... + 52022 )
4B = 52023 - 5
B = \(\dfrac{5^{2023}-5}{4}\)
b) Ta có : A + 2 = 2x
⇒ 22023 - 2 + 2 = 2x
⇒ 22023 = 2x
Vậy x = 2023
Lại có : 4B + 5 = 5x
⇒ 4 . \(\dfrac{5^{2023}-5}{4}\) + 5 = 5x
⇒ 52023 - 5 + 5 = 5x
⇒ 52023 = 5x
Vậy x = 2023
S
18 tháng 7 2018
a, \(A+B=x^2-2x-y^2+3y-1+\left(-2x^2+3y^2-5z+3\right)\)
\(=x^2-2x-y^2+3y-1-2x^2+3y^2-5z+3\)
\(=-x^2-2x+2y^2+3y-5z+2\)
b, \(A-B=x^2-2x-y^2+3y-1-\left(-2x^2+3y^2-5z+3\right)\)
\(=x^2-2x-y^2+3y-1+2x^2-3y^2+5z-3\)
\(=3x^2-2x-4y^2+3y+5z-4\)
c, Thay x=-2,y=1 vào biểu thức A-B ta được:
\(A-B=3.\left(-2\right)^2-2.\left(-2\right)-4.1^2+3.1+5z-4=12+4-4+3+5z-4=11+5z\)
18 tháng 7 2018
\(A=x^2-2x-y^2+3y-1\)
\(B=-2x^2+3y^2-5z+3\)
a) A+B =
\(\left(x^2-2x-y^2+3y-1\right)+\left(-2x^2+3y^2-5z+3\right)\)
\(=\left(x^2-2x^2\right)-\left(y^2+3y^2\right)-2x+3y-5z-1+3\)
\(=-x^2-4y^2-2x+3y-5z-1+3\)
\(=\left(-1-4-2+3-5-1+3\right).\left(x^2-x\right).y^2.z\)
\(=-7xy^2z\)
b ) Tính A-B ( tương tự A+B )
C) Thay x=-2 và y=1 vào biểu thức ta có :
\(-7xy^2z\)
\(=-7.-2.1.z\)
\(=14z\)